Constructing an exact sequence from a monomorphism using projective resolutions

Question

Let $\mathcal{A}$ be an abelian category (hence every object in $\mathcal{A}$ has a projective resolution), let $M$ and $N$ be two objects in $\mathcal{A}$. Consider the following commutative diagram 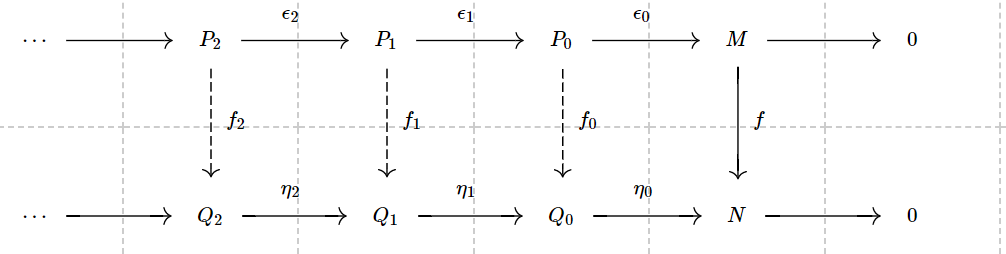where each row is a projective resolution and the existence of $f_0, f_1, \dots$ is given by comparison theorem.

Do we have the following or similar conclusion?

The map $f$ is a monomorphism if and only if the following sequence is exact: $$ \cdots \xrightarrow{} P_1\oplus Q_2 \xrightarrow{\left[ \begin{smallmatrix} \epsilon _1& 0\\ -f_1& \eta _2\\ \end{smallmatrix} \right] } P_0\oplus Q_1 \xrightarrow{\left[ \begin{smallmatrix} f_0& \eta_1 \end{smallmatrix} \right] } Q_0. $$

And how to prove that?

---

Enkidu told me I should consider Horseshoe lemma, but I don't know what to do.

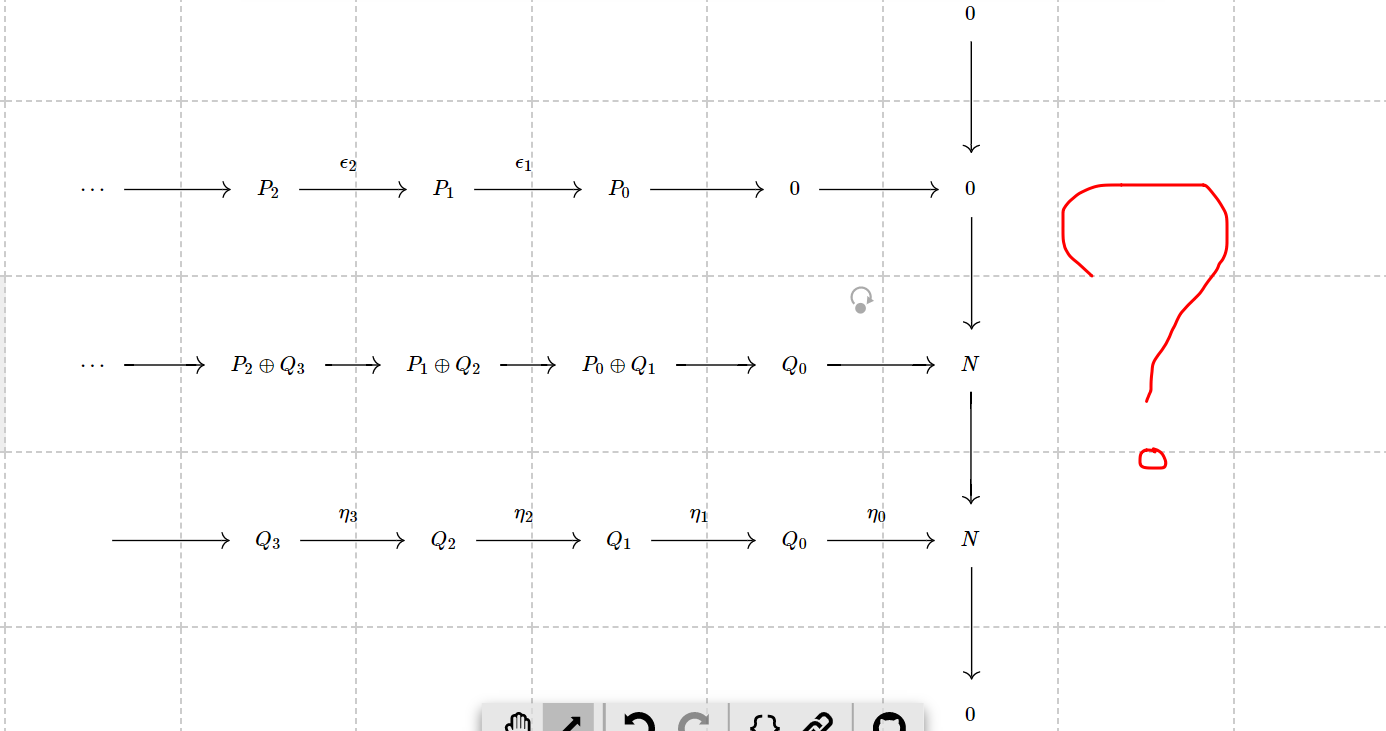
Thank you very much.

Answer 1

Note that not every abelian category has enough projectives (e.g. $\mathscr A = \mathbf{FAb}$, the category of finite abelian groups, does not), but of course you're free [no pun intended] to assume that $\mathscr A$ has enough projectives.

Note that the complex $$ \cdots \to P_1 \oplus Q_2 \to P_0 \oplus Q_1 \to M \oplus Q_0 \to N \to 0 $$ is exact, being the totalisation of a double complex with exact rows (the maps are the same matrices you wrote down, with the correct signs to make it a chain complex). It contains $0 \to M \to N \to 0$ as a subcomplex, and the quotient is the complex you describe. This gives a short exact sequence of chain complexes (written vertically): $$ \begin{array}{ccccccccccc} & & 0 & & 0 & & 0 & & 0 & & 0 \\ & & \downarrow & & \downarrow & & \downarrow & & \downarrow & & \downarrow \\ \cdots & \to & 0 & \to & 0 & \to & M & \to & N & \to & 0 \\ & & \downarrow & & \downarrow & & \downarrow & & \downarrow & & \downarrow \\ \cdots & \to & P_1 \oplus Q_2 & \to & P_0 \oplus Q_1 & \to & M \oplus Q_0 & \to & N & \to & 0 \\ & & \downarrow & & \downarrow & & \downarrow & & \downarrow & & \downarrow\\ \cdots & \to & P_1 \oplus Q_2 & \to & P_0 \oplus Q_1 & \to & Q_0 & \to & 0 & \to & 0 \\ & & \downarrow & & \downarrow & & \downarrow & & \downarrow & & \downarrow \\ & & 0 & & 0 & & 0 & & 0 & & 0.\!\end{array} $$ Taking the long exact homology sequence gives the result: the bottom sequence is exact at $P_0 \oplus Q_1$ if and only if $M \to N$ is injective. $\square$