Let $G$ be a simple $3$-connected plane graph with the vertices of its outer face pinned to the $xy$-plane. View each edge as an ideal rubber band, and each internal node given a weight proportional to its vertex-degree. The weights pull down the nodes vertically under gravity, so that the network sags into the halfspace $z \le 0$.
So this is a Tutte embedding with the addition of degree-weights pulling in the $-z$ direction. For example, here is the graph of a cube:
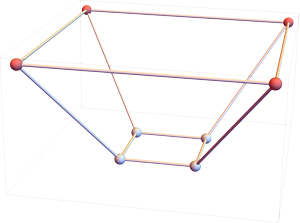
This is not so interesting because the four internal nodes each have the same degree, $3$. Here is an example where $u_1$ has degree-$3$ and $u_2$ has degree-$4$. Despite $u_2$'s heavier weight, $u_1$ is slightly lower in equilibrium.
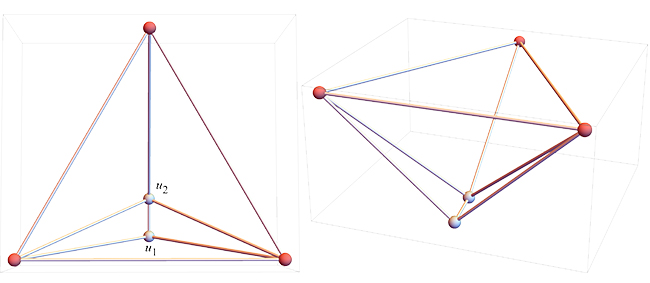
Two questions:
Q1. Has this degree-weighted representation been studied before? If so, does it possess any "nice" properties?
Q2. (This is too much to hope for, but...) Is every node of $G$ on the convex hull of the network in equilibrium?
I expect the answer to Q2 to be No because one can arrange, say, a high-degree node surrounded by low-degree nodes. But the balance of the rubber band forces complicates matters, as hinted in the example with a lighter node below a heavier node.
