Conditions on the velocity ensuring that a flow moves points along the boundary of a manifold

Question

Let

• $\tau>0$;
• $d\in\mathbb N$;
• $v:[0,\tau]\times\mathbb R^d\to\mathbb R^d$ be Lipschitz continuous in the second argument uniformly with respect to the first with $v(\;\cdot\;,x)\in C^0([0,\tau],\mathbb R^d)$;
• $X^{s,\:x}$ denote the unique element of $C^0([s,\tau],\mathbb R^d)$ with $$X^{s,\:x}(t)=x+\int_s^tv(r,X^{s,\:x}(r))\:{\rm d}r\;\;\;\text{for all }t\in[s,\tau]\tag1$$ for $(s,x)\in[0,\tau]\times\mathbb R^d$ and $$T_t(x):=X^x(t):=X^{0,\:x}(t)\;\;\;\text{for }x\in E$$ for $t\in[0,\tau]$.

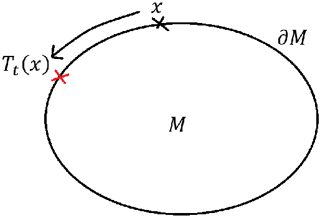
Now let $M$ be a $d$-dimensional embedded $C^1$-submanifold of $\mathbb R^d$ with boundary and $\partial M$ denote the manifold boundary of $M$.

I would like to know which assumption on $v$ we need to impose in order to ensure that $$T_t(\partial M)=\partial M\;\;\;\text{for all }t\in[0,\tau]\tag2.$$

Phrased differently, I want to find a condition on $v$ ensuring that $T_t$ moves a "particle" $x$ "along the boundary" $\partial M$ for all $t\in[0,\tau]$:

             

I've read that we need to assume that $$\langle\left.v\right|_{[0,\:\tau]\times\partial M},\nu_{\partial M}\rangle=0\tag3,$$ where $\nu_{\partial M}$ denotes the unique outer unit normal field on $\partial M$. However, I don't see why $(3)$ implies the $(2)$. And is $(3)$ an additional assumption at all? It seems like $(3)$ should always hold: Let $(s,x)\in[0,\tau]\times\partial M$. The claim $\langle v(s,x),\nu_{\partial M}(x)\rangle=0$ is equivalent to $v(s,x)\in T_x\:\partial M$, where $T_x\:\partial M$ denotes the tangent space of $\partial M$ at $x$. Now, if $$\gamma(h):=X^{s,\:x}(s+h)\;\;\;\text{for }h\in[0,\tau-s],$$ then $\gamma(0)=x$ and $\gamma'(0)=v(s,x)$. Thus, $v(s,x)\in T_x\:\partial M$. Am I missing something?

Answer 1

In the situation you described, if $M$ is properly embedded (i.e., topologically embedded and closed), the flow of a vector field takes $\partial M$ to itself if and only if the vector field is everywhere tangent to the boundary. For a proof of the "if" direction, see Lemma 9.33 in my Introduction to Smooth Manifolds. (That lemma shows that the flow takes $M$ to itself if the vector field is everywhere tangent to the boundary, but if you look closely at the proof, it also shows that the flow preserves $\partial M$.)

The condition that $v$ is tangent to the boundary at a point $x\in \partial M$ is equivalent to the condition that $v$ is orthogonal to the unit normal vector there. (This is essentially the definition of a normal vector.)

If $M$ is not closed, the result is not true. For example, consider the case in which $M$ is the closed unit disk in $\mathbb R^2$ with one boundary point removed, and $v$ is a vector field that generates rotations. Then there is no nonzero time such that $T_t(\partial M)\subset\partial M$.

To answer the question in your comment: Once we know that $T_t(\partial M)\subset \partial M$ for each $t$, here's how to show that in fact equality holds.

First suppose $\partial M$ is connected. Because $T_t$ is a diffeomorphism from $\mathbb R^d$ to itself and $\partial M$ is closed in $\mathbb R^d$, it follows that $T_t(\partial M)$ is closed in $\mathbb R^d$ and therefore also (relatively) closed in $\partial M$. On the other hand, since $T_t$ restricts to a diffeomorphism from $\partial M$ to itself, $T_t|_{\partial M}$ is an open map (as a map between $(d-1)$-dimensional manifolds), and therefore $T_t(\partial M)$ is (relatively) open in $\partial M$. By connectivity, therefore $T_t(\partial M) = \partial M$.

If $\partial M$ is not connected, just apply the argument above to each connected component.