Existence of twisted metaplectic categories

Question

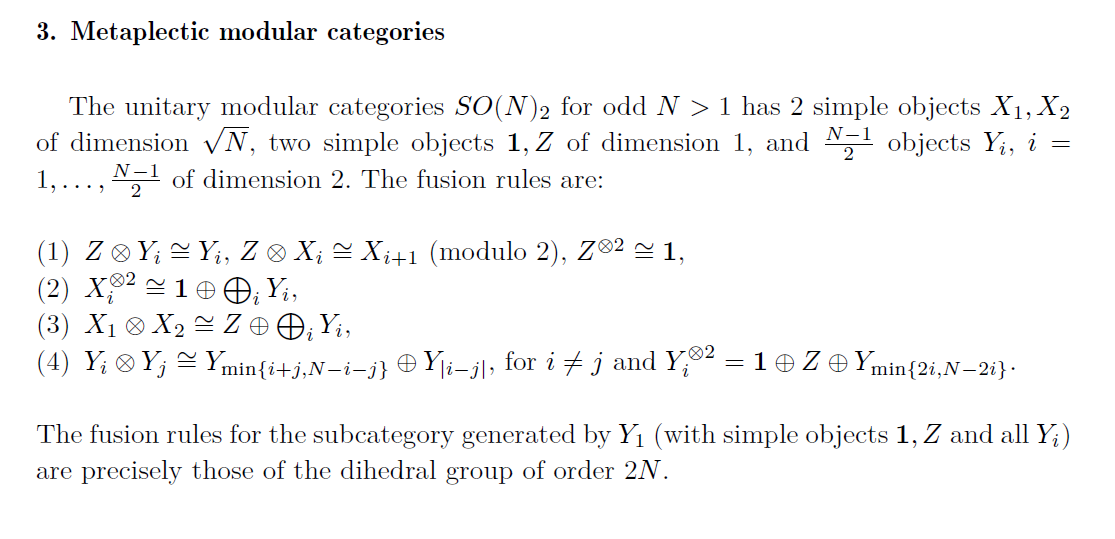
The paper Classification of metaplectic modular categories by Ardonne-Cheng-Rowell-Wang (2016) mentions (in Section 3) the Grothendieck ring for the metaplectic modular categories, i.e. $SO(N)_2$, $N>1$ odd (see below).

Now by exchanging $1$ and $Z$ in lines (2) and (3), we get a new family of fusion rings. 

Question 1: Are there (unitary) fusion categories corresponding to this new family?
(which could be called twisted metaplectic)

Question 2: Can this procedure be extended to other fusion categories?

There is a way to make two new families: in the right hand-side of (2) and (3), put $$\oplus (X_1 \oplus X_2)^{\oplus \frac{N-1}{2}}$$ By this modification, you still have a fusion ring, but the objects $X_1, X_2$ are now of FPdim $N$. Again you have the usual and the twisted version. Note that after this modification, the usual case with $N=3$ corresponds to the Grothendieck ring of $\mathrm{Rep}(S_4)$.

Bonus question 1: Are there (unitary) fusion categories corresponding to these new families?

Bonus question 2: Can this procedure be extended to other fusion categories?

Answer 1

In https://arxiv.org/pdf/2005.05544.pdf we describe a general procedure that accomplishes (1) and (2) which we call zesting.

In this case, let $\mathcal{C}$ be an odd metaplectic category. In the notation above, let $\lambda\in Z^2(\mathbb{Z}/2,Inv(\mathcal{C}))$ be chosen with $\lambda(1,1)=Z$. Then a new tensor product defined by $U\hat{\otimes} V\cong U\otimes V\otimes \lambda(d(U),d(V))$ where $d$ is the grading degree function, utilizing the $\mathbb{Z}/2$ grading on $\mathcal{C}$. In particular $X_1\hat{\otimes} X_2\cong X_1\otimes X_2\otimes Z\cong X_1\otimes X_1$ etc. As $Z$ is a boson (we are using the braiding on $\mathcal{C}$ in our construction) Proposition 6.3 in loc. cit. says that we further twist the associativity by a $3$-cocycle.
Proposition 6.4 shows that the resulting fusion category does not admit a braiding.

One can proceed in a similar way for general $N$-metaplectic categories too (i.e. $N$ can be odd or even).