Construct closed chain of $k$-gon around $n$ points-$n, k$ are odd primes number

Question

Question 1: I am looking for a proof of the conjectures 1, 2, 3 as follows?

Question 2: In conjecture 3, in general case, I can not give a formula of $X$. But I think, If $n, k$ are odd primes number then $X=\frac{2nk}{gcd(k-2,2k)gcd(n,k)}$, I checked with some small case. Can You give a general formula of $X$?

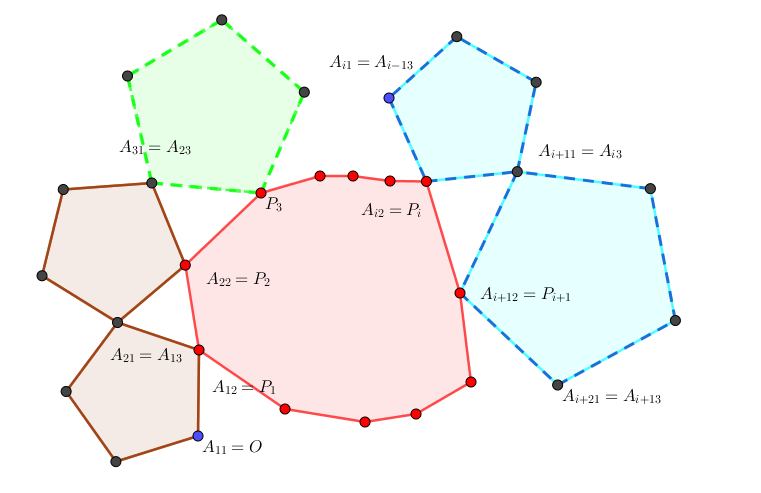
Consider $n, k \ge 3$ be two integer numbers, given $n$ general points $P_1$, $P_2$,....,$P_n$ and $O$ is arbitrary point in the plane, let $P_{n+i}=P_i$ for $i=1,\ldots,....$. Construct a chain of $m$ regular $k$-gon:

• Construct first $k$-gon: $A_{1\;1}A_{1\;2}....A_{1\;k}$ such that $A_{1\;1}=O$; $A_{1\;2}=P_1$, the centroid of the first $k$-gon is $A_1$

• Second $k$-gon: $A_{2\;1}A_{2\;2}....A_{2\;k}$ such that $A_{2\;1}=A_{1\;3}$; $A_{2\;2}=P_2$ the centroid of the second $k$-gon is $A_2$

• $.................................$

• $i$ th $k$-gon: $A_{i\;1}A_{i\;2}....A_{i\;k}$ such that $A_{i+1\;1}=A_{i\;3}$; $A_{i+1\;2}=P_{i+1}$ the centroid of the $i$ th $k$-gon is $A_i$

• $.................................$

• $m$ th $k$-gon: $A_{m\;1}A_{m\;2}....A_{m\;k}$ such that $A_{m\;1}=A_{m-1\;3}$; $A_{m\;2}=P_{m}$ the centroid of the $m$ th $k$-gon is $A_m$

and all regular polygon is same direction.

Definition: The chain is closed if exist $m$ such that $A_{m\;3}=A_{1\;1}=O$. The chain is open if no exist $m$ such that $A_{m\;3}=A_{1\;1}=O$

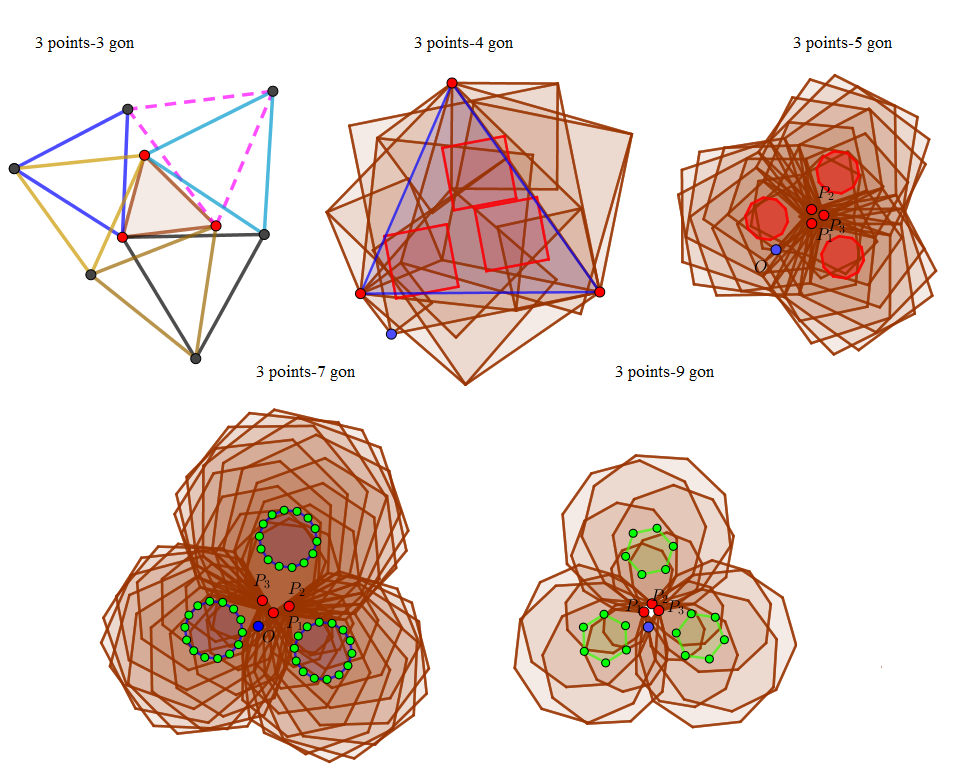
Conjecture 1: If $n=\frac{2k}{gcd(k-2,2k)}$ then the chain is opened.

Conjecture 2: If $n\ne\frac{2k}{gcd(k-2,2k)}$ then the chain is closed.

Conjecture 3: If the chain is closed then $m=n.X$ and $X$ points $A_i, A_{n+i}, A_{2n+i},...,A_{nx+i}$ be form $X$-gon for $i=1, 2,...,n$ which the centroid of the $X$-gon is fixed when we moved $O$, these regular polygon equal.

UPDATE GEOGEBRA SOFTWARE APPLET

• 3-gon and 3 points

• 4-gon and 3 points

• 5-gon and 3 points

• 7-gon and 3 points

• 8 gon and 3 points

• 9-gon and 3 points

• 11-gon and 3 points

• 3-gon and 5 points

• 4-gon and 5 points

• 4-gon and 6 points

• 3-gon and 7 points

See also:

• Napoleon theorem

• Van Aubel theorem

• Petr–Douglas–Neumann theorem

Answer 1

We can consider all given points as complex numbers, points of the complex plane. Then, as far as I understood, for all integers $m\ge 1$, $1\le j\le k$ we we have $$A_{m,j}=A_m+(P_m-A_m)\xi^{j-2},$$
where $\xi=\exp\frac{2\pi i}{k}$.

Since $A_{m+1,1}= A_{m,3}$, we have

$$A_{m+1}+(P_{m+1}-A_{m+1})\xi^{-1}=A_m+(P_m-A_m)\xi.$$

It follows

$$A_{m+1}=-A_m\xi+\frac{P_m\xi^2-P_{m+1}}{\xi-1}.$$

This formula suggests that the chain can be closed for a specific choice of $O$ and $P_m$’s, but I guess that you are looking for a stable general pattern. So let’s look when the sequence $\{A_m\}$ is periodic.

Putting $B_m=A_m(-\xi)^{-m}$, we obtain

$$B_{m+1}=B_m+\frac{P_m\xi^2-P_{m+1}}{\xi-1}(-\xi)^{-m-1}.$$

Since the sequence $\{P_n\}$ has a period $n$, we have

$$B_{m+2n}-B_{m+n}=(B_{m+n}-B_{m}) (-\xi)^{-n},$$

that is

$$A_{m+2n}-A_{m+n}(1+(-\xi)^{n})+A_m(-\xi)^{n}=0.$$

An equation $\lambda^2-(1+(-\xi)^{n})\lambda +(-\xi)^{n}$ has roots $1$ and $(-\xi)^{n}$. The following cases are possible.

1)) $(-\xi)^{-n}=1$. This holds iff ($n$ is even and $k|n$) or ($n$ is odd, $k$ is even and $k|2n$). The theory of recurrence relations implies that $A_{r n+m}=c_1(m) + c_2(m)r$ for each $r$ and some constants $c_1(m)$ and $c_2(m)$ depending on $m$. If all $c_2(m)$ are zeroes then the sequence $\{A_m\}$ has a period $n$ (or its divisor). Otherwise the sequence $\{A_m\}$ is not periodic. Thus the sequence $\{A_m\}$ is periodic iff for each $m$ we have $A_m=A_{m+n}$. This can happen iff the choice of $P_m$’s is specific. Namely,

1.1)) If $n$ is even and $k|n$ then

$$0=A_{m+n}(-\xi)^{-m-n}- A_{m}(-\xi)^{-m}=B_{m+n}-B_{m}=\frac{1+\xi }{1-\xi}\sum_{j=1}^n P_{m+j} (-\xi)^{-(m+j)},$$

that is, $(-\xi)^{-1}$ is a root of a polynomial $P(x)=\sum_{j=1}^n P_{j} x^j$.

1.2)) If $n$ is odd, $k$ is even, and $k|2n$ then

$$0=A_{m+2n}(-\xi)^{-m-2n}- A_{m}(-\xi)^{-m}=B_{m+2n}-B_{m}=\frac{1+\xi }{1-\xi}\sum_{j=1}^{2n} P_{m+j} (-\xi)^{-(m+j)},$$

that is, $(-\xi)^{-1}$ is a root of a polynomial $(1+x^n)P(x)=\sum_{j=1}^{2n} P_{j} x^j$

2)) $(-\xi)^{-n}\ne 1$. (This case holds, in particular, when both $n$ and $k$ are odd). The theory of recurrence relations implies that $A_{r n+m}=c_1(m) + c_2(m)(-\xi)^{nr}$ for each $r$ and some constants $c_1(m)$ and $c_2(m)$ depending on $m$. If all $c_2(m)$ are zeroes then the sequence $\{A_m\}$ has a period $n$ (or its divisor). Otherwise $-\xi$ is a primitive $q$-th root of unity, where $$q=\cases{k, \mbox{ if }k\equiv 0\pmod 4\\ k/2, \mbox{ if }k\equiv 2\pmod 4\\ 2k, \mbox{ if }k\equiv 1,3\pmod 4}.$$

Remark that $q=\frac{2k}{\gcd(k-2,2k)}=\frac{2k}{\gcd(k-2,4)}$. Thus $(-\xi)^n$ is a primitive $\tfrac{q}{\gcd(q,n)}$-th root of unity, and so the sequence $\{A_m\}$ has a period $\tfrac{qn}{\gcd(q,n)}=\operatorname{lcm}(q,n)$ (or its divisor). Moreover, for each $m$, points $\{A_{r n+m}: 0\le r\le q-1\}$ are vertices of a $q$-qon.

Finally, recall that for each $m\ge 1$, $1\le j\le k$ we have $A_{m,j}=A_m+(P_m-A_m)\xi^{j-2}$. It follows that if the sequence $\{A_m\}$ has a period $p$ then for each fixed $j$ a sequence $\{A_{m,j}\}$ has a period $\operatorname{lcm}(p,n)$ (or its divisor).