I am trying to understand the exact implications between local solvability and a general version of the Cauchy-Kovalevskaya (CK) theorem, in the context of PDEs.
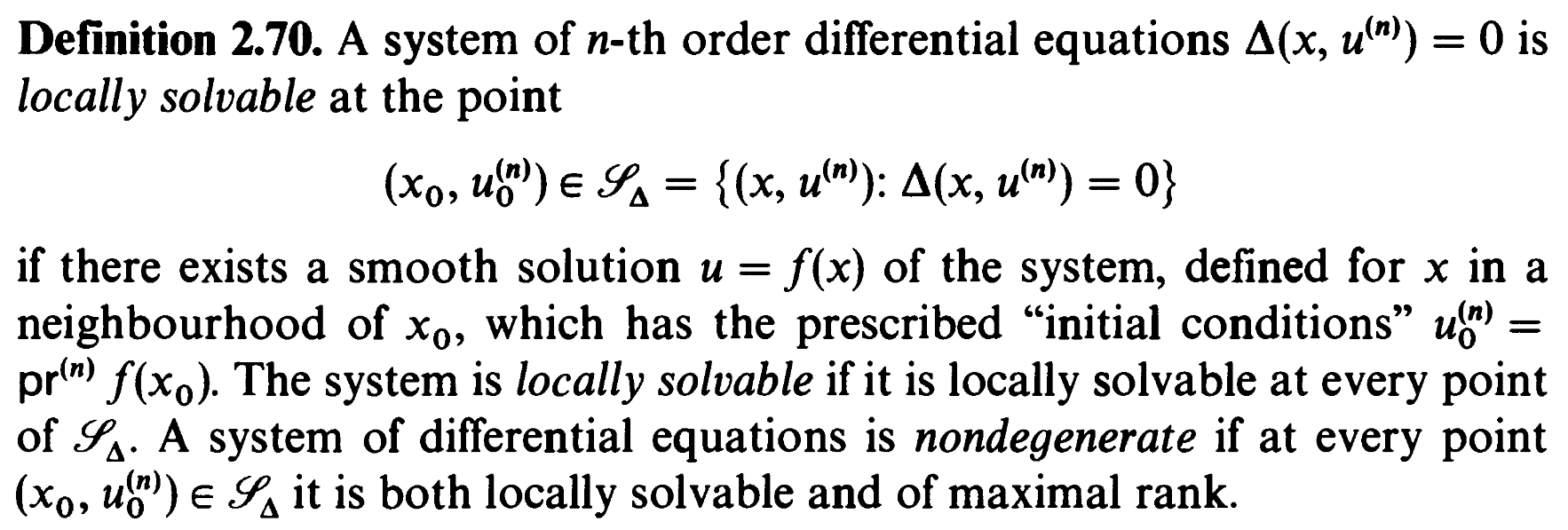
Let $\Delta(x,u^{(n)})=0$ be a system of PDEs of order $n$. Here $x$ is the vector of independent variables, and $u^{(n)}$ is the vector of dependent variables and all their derivatives up to order $n$. According to Olver ("Applications of Lie groups to Differential Equations", 2/e, Ch. 2, Def. 2.70), $\Delta$ is locally solvable if the variety it induces on $\mathbb{R}^{|x|+|u^{(n)}|}$
$V(\Delta):=\{(x_0,u_0^{(n)}):\Delta(x_0,u_0^{(n)})=0\}$
coincides with its solution variety
$S(\Delta):={\small \{(x_0,u_0^{(n)})\in V(\Delta) : \text{ $\exists$ an analytic solution $U$ of $\Delta$ in a neighborhood of $x_0$ s.t. } U^{(n)}(x_0)=u_0^{(n)} \}}$
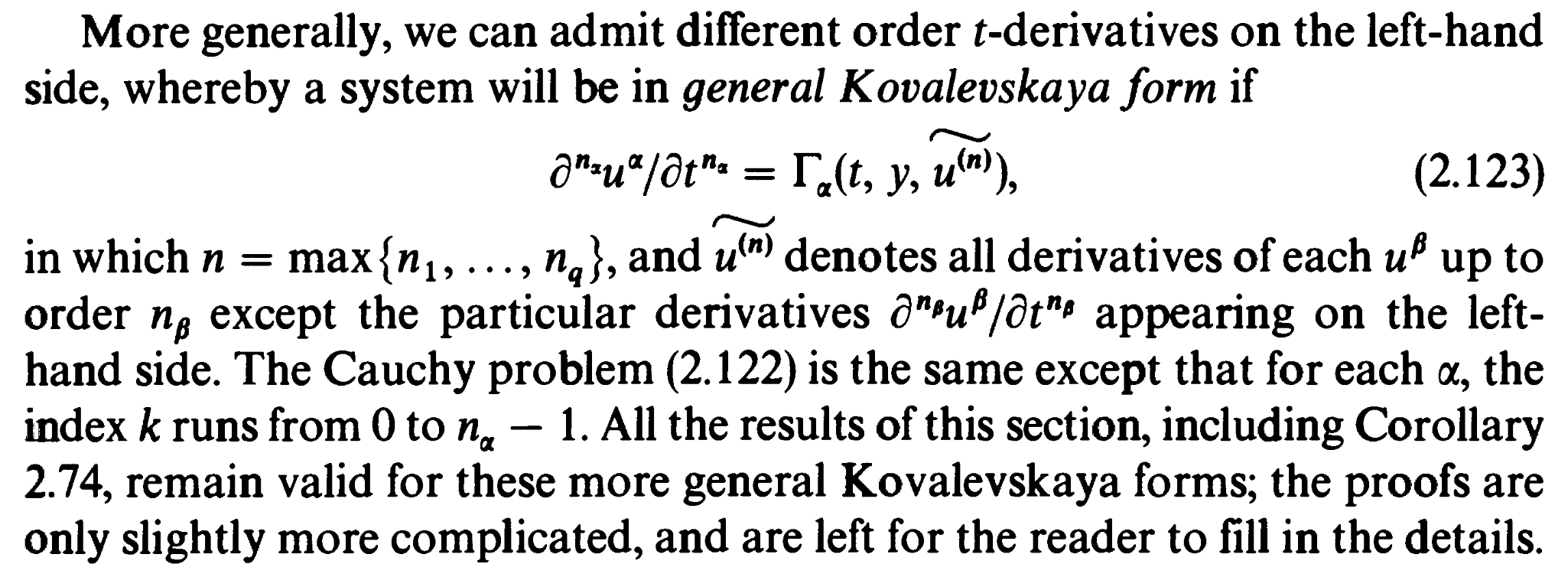
Olver shows that if $\Delta$ is in Kovalevskaya form then it is locally solvable (Corollary 2.74, p. 163; this is indeed an easy consequence of CK theorem). He then states that the same result still holds when $\Delta$ is in general Kovalevskaya form:
I am struggling to convince myself of the validity of this statement. For instance, consider the 2nd order system $\Delta$ in the independent variables $t,x$ and the dependent variables $u,v$: \begin{align*} u_t & = v\\ v_{tt} &= u_x\,. \end{align*} From what I gather, this system is not locally solvable. Indeed, there are differential consequencences that are not captured algebraically by the two equations above, such as $u_{tx}=v_x$. So there are points $(x_0,u_0^{(2)})\in V(\Delta)$ s.t. (with obvious notation) $u_{0,tx}\neq v_{0,x}$, hence not in $S(\Delta)$.
Yet, $\Delta$ is in general Kovalevskaya form, is it?
Edit: for reference, I paste Olver's original definition. Note that $pr^{(n)}f$ denotes the $n$-th prolongation of $f$.