5
$\begingroup$

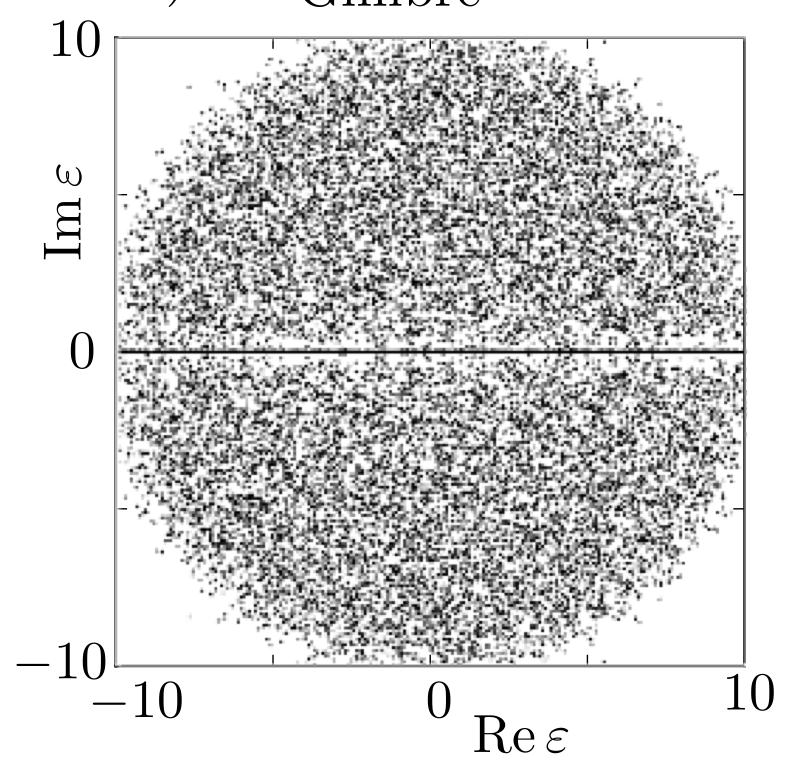
Let us assume we have a square matrix $A$ whose entries are sampled from a standard Gaussian distribution of mean $0$. Do we have any information about the distribution of its eigenvalues?

Particularly, I'm aware that there are different results on symmetric gaussian matrices (or, the Gaussian orthogonal ensemble of $A$):

  • The eigenvalues follow a semicircle law

Is there an equivalent result for standard, non-symmetric Gaussian matrices?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ I have answered this for the ensemble of nonsymmetric matrices, but do note that your statements on the symmetric case (GOE) are not correct: the eigenvalues follow a semicircle law, not a Gaussian distribution. $\endgroup$
    – Carlo Beenakker
    Commented Mar 28, 2020 at 11:42
  • $\begingroup$ You are right. Thanks for the answer, I'll edit for future readers! $\endgroup$
    – Alfred
    Commented Mar 30, 2020 at 17:22

1 Answer 1

Reset to default
8
$\begingroup$

This is the Ginibre ensemble, see Eigenvalue statistics of the real Ginibre ensemble for the eigenvalue distribution. For an $N\times N$ matrix with $N\gg 1$ there are on average $\sqrt{2N/\pi}$ eigenvalues on the real axis, uniformly in the interval $(-\sqrt N,\sqrt N$). The rest of the eigenvalues fill a disc of radius $\sqrt N$ in the complex plane, uniformly except for a depleted strip along the real axis. Here is a scatter plot of the eigenvalues for $N=100$ (taken from arXiv:1305.2924).

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .