$A$ a Noetherian local ring, $M\neq 0$ a finite $A$-module. I'm not quite sure about the relation between finiteness of projective and injective dimensions of $M$. Does the finiteness (or infiniteness) of one necessarily imply the finiteness (or infiniteness) of another?
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
7
To complement Mariano's answer: If finite projective dimension implies finite injective dimension for any module $M$, then $R$ better have finite injective dimension (the converse is also quite easy).
The local rings $R$ which have finite inj. dim. over themselves are also known as Gorenstein rings. In fact, a theorem by Foxby says that $R$ possesses a module of both finite proj. and inj. dim. if and only if $R$ is Gorenstein.
answered Aug 6, 2010 at 0:55
-
$\begingroup$ Thanks. How do I see that if $R$ has a finite injective dimension then finite projective dimension of $M$ implies finite injective dimension of $M$? $\endgroup$– ashpoolCommented Aug 6, 2010 at 16:10
-
$\begingroup$ You can use induction on the length of the min. free res. of $M$, for instance. $\endgroup$ Commented Aug 6, 2010 at 17:23
-
$\begingroup$ I'm sorry, I still have no idea how to proceed. To begin with, if $A$ has a finite injective dimension and $M$ is projective over $A$, why does $M$ have a finite injective dimension? If you could point to any reference that would be great, too. $\endgroup$– ashpoolCommented Aug 7, 2010 at 3:34
-
3$\begingroup$ @kwan: break the res. into short exact sequences and use the fact that if 2 modules have fi. inj. dim., so is the third one. For ref (without proof), look at "Cohen-Macaulay rings" 1st ed by Bruns-Herzog, Section 3.1, esp. exer. 3.1.25. $\endgroup$ Commented Aug 7, 2010 at 18:08
-
1$\begingroup$ @kwan: here is a proof from top of my head, but there might be easier ones: replacing $M$ by high syzygy, can assume $M$ is max. Cohen-Macaulay. Then kill a full reg. seq., -> assume $R$ is Artinian. But then $M$ is injective, so projective. $\endgroup$ Commented Aug 7, 2010 at 21:08
$\begingroup$
$\endgroup$
No. For example, there are rings $A$ for which the residue field $k$ is of infinite projective dimension but of finite injective dimension. On the other hand, if $k$ is of finite projective dimension, then $A$ is of finite global dimension, so $k$ (and everything else) has finite injective dimension.
For a small non-commutative example, consider the algebra $A$ which is the quotient of the path algebra of the quiver
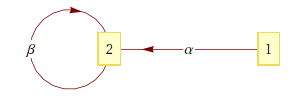
modulo the ideal generated by $\beta\alpha$ and $\beta^2$. Then the simple module supported on the vertex $1$ is an injective of infinite projective dimension. By duality, the opposite algebra has a projective module of infinite injective dimension.
answered Aug 6, 2010 at 0:21