This is false. I will use freely that $f \colon A \to B$ is an epimorphism if and only if $B \otimes_A B \to B$ is an isomorphism; in particular this is also equivalent to $\operatorname{Spec} B \to \operatorname{Spec} A$ being a monomorphism.
Example. Let $k$ be a field of characteristic $0$, and let
$$R = k\left[x,\tfrac{1}{x-1},\tfrac{1}{x-2},\ldots\right].$$
Then $R$ has a self-map $x \mapsto x-1$, whose image on spectra is the complement $U$ of the origin. Note that $U \amalg \{0\} \to \operatorname{Spec} R$ is a monomorphism, so the map
\begin{align*}
g \colon R &\to R \times k\\
x &\mapsto (x-1,0)
\end{align*}
is an epimorphism. Now let $A = R \times k$, and let $f$ be the composition $A \stackrel\pi\twoheadrightarrow R \stackrel g\to A$, where $\pi$ is the natural projection. It is an epimorphism since both $\pi$ and $g$ are, and it is clearly not injective. $\square$
Picture. Here is a picture of the situation: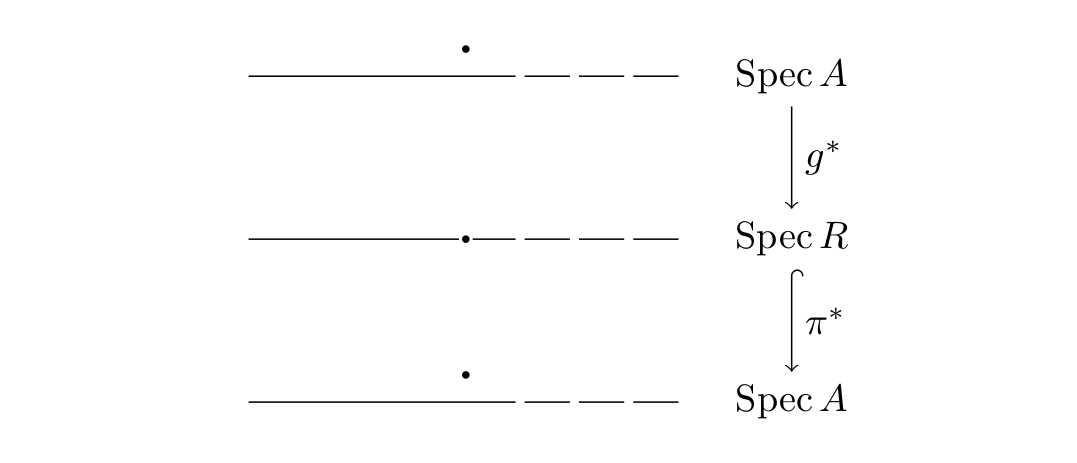
Remark. However, the result is true if $A$ has a unique associated prime $\mathfrak p$ (i.e. $A$ is irreducible without embedded primes). Indeed, we get an ascending chain
$$\mathfrak p \subseteq f^{-1}(\mathfrak p) \subseteq f^{-1}(f^{-1}(\mathfrak p)) \subseteq \ldots,$$
which stabilises by the Noetherian hypothesis. But if $\operatorname{Spec} A \to \operatorname{Spec} A$ is a monomorphism, in particular it is injective, so this forces $\mathfrak p = f^{-1}(\mathfrak p)$. Then the base change $A_{\mathfrak p} \to (f_*A)_{\mathfrak p}$ of $f \colon A \to f_*A$ along $A \to A_{\mathfrak p}$ is an epimorphism as well, hence so is the composition $A_{\mathfrak p} \to (f_*A)_{\mathfrak p} \to A_{\mathfrak p}$ since it is a further localisation. But this is an epimorphism of Artinian rings, hence surjective by this post. Since both rings are Artinian of the same length, we conclude that it's an isomorphism. Since $\mathfrak p$ is the unique associated prime, the map $A \to A_{\mathfrak p}$ is injective, which gives the result.
I'm not sure what happens if $A$ is allowed to have embedded primes. It seems hard to imagine what a counterexample could look like, but I also have no argument to rule it out.
Remark. Note also that there are trivial counterexamples without the Noetherian hypothesis, even if $A$ is a domain. For example, $A = k[x_0,x_1,\ldots]$ has a surjective endomorphism that is not injective.

