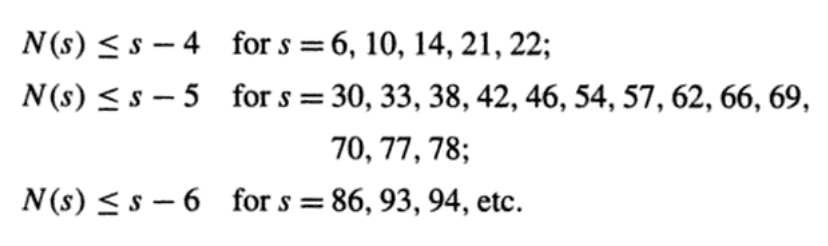As is usual, let $N(n)$ denote the maximum size of a set of mutually orthogonal Latin squares of order $n$. I am wondering what results hold that bound $N(n)$ from above; the only ones I can think of are the following:
$N(n)\leq n-1$ for all $n\geq 2$, with equality if $n$ is a prime power. This is well known.
$N(6)=1$. This is also quite famous.
$N(10)\leq 8$. This was done using a computer search. (Source)
If $n=1$ or $2~(mod~4)$, and if $n$ is not a sum of two squares, then $N(n)< n-1$. This is the Bruck-Ryser Theorem from 1949, though stated in Latin squares instead of projective planes.
Are there any other results of this sort? I know of many results bounding $N(n)$ from below (mainly Beth's result that $N(n)\geq n^{1/14.8}$ if $n$ is large enough, and several results of the form "If $n\geq n_\nu$ then $N(n)\geq \nu$"), but neither I nor anyone I know can add to this list, and I haven't had much luck on Google either.