Given a manifold $M$ we have a geometric stack associated to it namely $\underline{M}$ whose objects are smooth maps to $M$. For the sake of consistency I am writing $BM$ for $\underline{M}$.
Given a Lie group $G$ we have a geometric stack associated to it namely $BG$ whose objects are principal $G$ bundles.
Given a Lie groupoid $\mathcal{G}$ we have a geometric stack associated to it namely $B\mathcal{G}$ whose objects are principal $\mathcal{G}$ bundles.
These are called as Yoneda embedddings (I do not have precise reference where it is called so, except for manifolds corollary $4.16$).
Given a smooth map $f:M\rightarrow N$, if it is a submersion, then, $M\times_NM$ is a manifold. We have $2$-fibre product $\underline{M}\times_{\underline{N}}\underline{N}$ and the stack $\underline{M\times_NM}$.
I am able to see that $\underline{M\times_NM}\cong \underline{M}\times_{\underline{N}}\underline{M}$. We have $B(M\times_NM)\cong BM\times_{BN}BN$.
David Roberts say here that same holds in case of Lie groups and Dimitri Pavlov say here that same holds for Lie groupoids i.e., we have following.
Given a morphism of Lie groups $\theta:G\rightarrow H$ which is a surjective submersion (submersion is to ensure $G\times_H G$ is a Lie group), then $$B(G\times_HG)\cong BG\times_{BH}BG.$$
Given a morphism of Lie groupoids $f:\mathcal{G}\rightarrow \mathcal{H}$ such that the fibered product (in page no $5$, section $2.3$) $\mathcal{G}\times_{\mathcal{H}}\mathcal{G}$ is a Lie groupoid, then $$B(\mathcal{G}\times_{\mathcal{H}}\mathcal{G})\cong B\mathcal{G}\times_{B\mathcal{H}}B\mathcal{G}$$
Dmitri Pavlov said here that this has something to do with Preservation of limits by the Yoneda embedding and suggested this and this. But I am not familiar with $(\infty,1)$ categories. So, I am asking here (I am asking as a separate question).
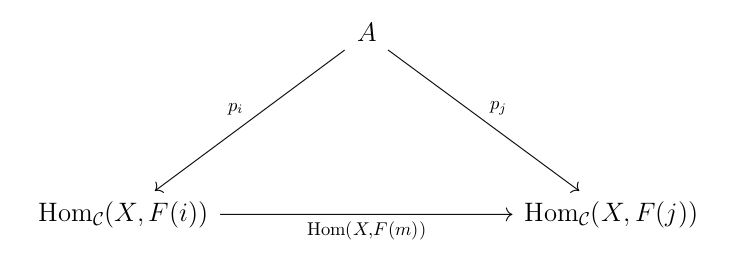
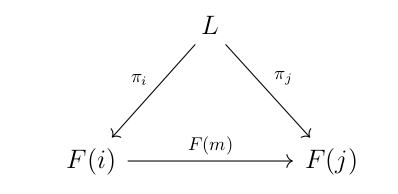
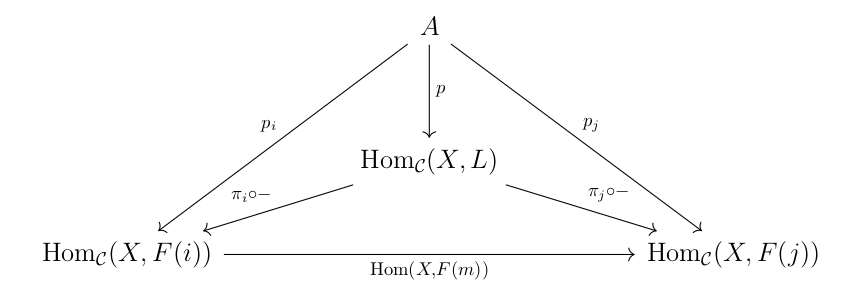
How does one see that Yoneda embedding preserves limits in this setup? Please see my answer, I see the case in classical category theory.
Just an outline is also ok, just that it would be good if it is not mixed with $(\infty,1)$ categories.