Certain formulas I really enjoy looking at like the Euler-Maclaurin formula or the Leibniz integral rule. What's your favorite equation, formula, identity or inequality?
$\begingroup$
$\endgroup$
7
-
2$\begingroup$ Voting to close. People are at the repeating-other-people's-answers stage now. $\endgroup$– Qiaochu YuanCommented Aug 21, 2010 at 18:23
-
3$\begingroup$ The question has been closed as no longer relevant. It had a long and healthy life, but the large number of answers has become unwieldy. If the question had been asked more recently, it would probably have been closed sooner as being "overly broad". I encourage people who are interested in following up issues raised in the question or the answers with further questions. Please be specific! $\endgroup$– Pete L. ClarkCommented Aug 22, 2010 at 9:02
-
2$\begingroup$ Sadly my favourite $\sum \frac{1}{n^2 +a^2} = \frac{\pi}{a} cth(\pi a)$ wasn't listed $\endgroup$– Ostap ChervakCommented May 1, 2011 at 16:57
-
1$\begingroup$ $$Rf_*R\hbox{Hom}(F,f^!G)\approx R\hbox{Hom}(Rf_!F,G)$$ $\endgroup$– Steven LandsburgCommented Oct 22, 2016 at 22:01
-
1$\begingroup$ Mine is Poisson formula which can take the form $\int_H f = \int_{H^{\perp}} \hat f$. $\endgroup$– WatsonCommented Dec 21, 2019 at 9:36
|
Show 2 more comments
62 Answers
$\begingroup$
$\endgroup$
With the stuff I've seen in the literature of sequence transformations, I've started to love the formulae for Aitken's Δ² process:
$S_n^{\prime}=S_{n+1}-\frac{(\Delta S_n)^2}{\Delta^2 S_n}$
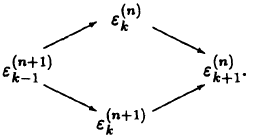
and its generalization the Wynn ε algorithm:
$\varepsilon_{k+1}^{(n)}=\varepsilon_{k-1}^{(n+1)}+\frac1{\varepsilon_{k}^{(n+1)}-\varepsilon_{k}^{(n)}}$
for the latter one especially because it is nicely represented as a lozenge diagram: