4
$\begingroup$

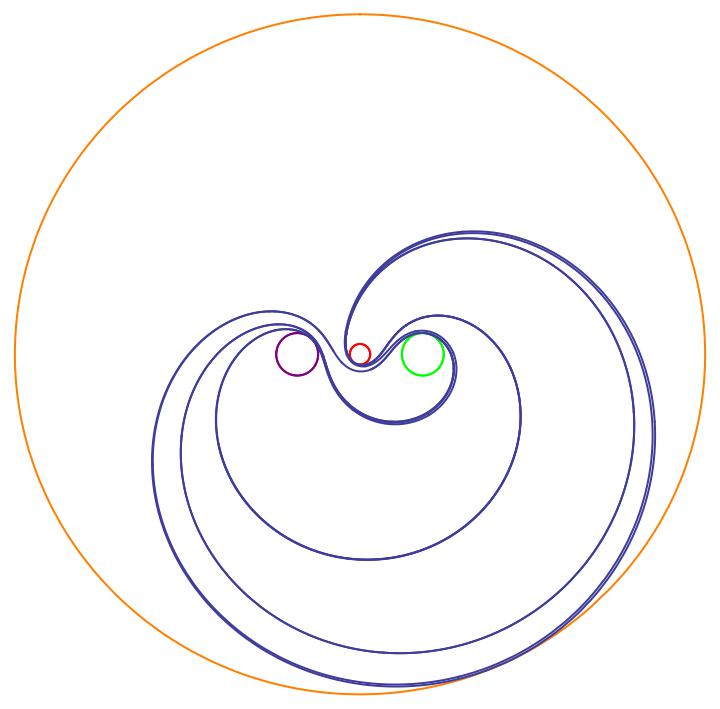
Thinking of $\mathbb {CP^1}$ as the sphere $S^2\subset\mathbb R^3$, we can define the notion of a circle on it to be a subset that is got by a hyperplane section of $S^2$ inside $\mathbb R^3$. This notion is known to be invariant under the complex automorphism group $PSL_2(\mathbb C)$ of $\mathbb {CP^1}$.

Suppose $n\ge 3$ is an integer. Then, it is known that the complement of $n$ distinct points $\{z_1,\ldots,z_n\}$ on $\mathbb {CP^1}$ carries a unique complete hyperbolic conformal metric of finite area, call it $g$. It is also known that if $\gamma$ is a simple closed curve on $X = \mathbb {CP^1}-\{z_1,\ldots,z_n\}$ which is homotopically non-trivial, then it is homotopic to a unique simple closed geodesic for $g$. Let us continue to denote this by $\gamma$. Is it then true that $\gamma\subset \mathbb {CP^1}$ is a circle in the sense of the previous paragraph?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
6
$\begingroup$

If the points are (setwise) invariant under reflection through a plane perpendicular to the sphere, then the the great circle of the reflection plane intersecting the sphere will be geodesic in the hyperbolic metric on the complement of the points. More generally one may consider inversions through spheres preserving the points (conformally equivalent to the plane example). It seems unlikely otherwise that a hyperbolic geodesic could be a circle (for example, one cannot just rotate the two hemispheres in the symmetric situation above, since the great circle will likely not be isometric to the hyperbolic geodesic). If we had a single configuration with two disjoint hyperbolic geodesic circles, we could get infinitely many more by cutting along the two circles, and doing inversions of the annulus through them $n$ times, then capping off with the disks on either end. But I suspect such examples are unlikely to exist.

Here are pictures of hyperbolic geodesic curves in the 4-punctured sphere iterated by a braid (one puncture is at $\infty$). The first blue curve is a circle (stereographic projection will take it to a circle on the sphere). The colored circles surround the punctures, and are horocycles, not round circles.

enter image description here

enter image description here

enter image description here

enter image description here

enter image description here

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Sorry for reviving an old question, but is it clear that the horocycles around the punctures will not be circles (in the sense of the question) on the original $\mathbb P^1$? $\endgroup$
    – Mohan Swaminathan
    Commented Mar 4, 2019 at 16:49
6
$\begingroup$

Unless I misunderstand the question, the answer is no. The homotopy class of the circle is determined by the partition it determines on the set of marked points, so there are only finitely many homotopy classes. By contrast, there are infinitely many homotopy classes of simple closed geodesics as long as the number of marked points is greater than $3.$

See, e.g.

Rivin, Igor, Simple curves on surfaces, Geom. Dedicata 87, No. 1-3, 345-360 (2001). ZBL1002.53027.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks! Are those circles (determined by the various partitions) geodesics though? $\endgroup$
    – Mohan Swaminathan
    Commented Oct 5, 2018 at 0:00
  • $\begingroup$ I see no reason why they should be (and notice that if there are enough points there will be NO circle defining a given partition). $\endgroup$
    – Igor Rivin
    Commented Oct 5, 2018 at 0:54

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .