I'm currently studying Iwasawa theory.
1) There are many $\mathbb{Z}_p$-modules on which some Galois groups act. So I often face some facts on the group representation over local fields or p-adic integer ring. But I can't find any references yet.
Of course, there are articles on p-adic representation. But I want references that are not too deep. I want references using just easy-to-follow arguments of algebra and representation theory.
Can you suggest any references?
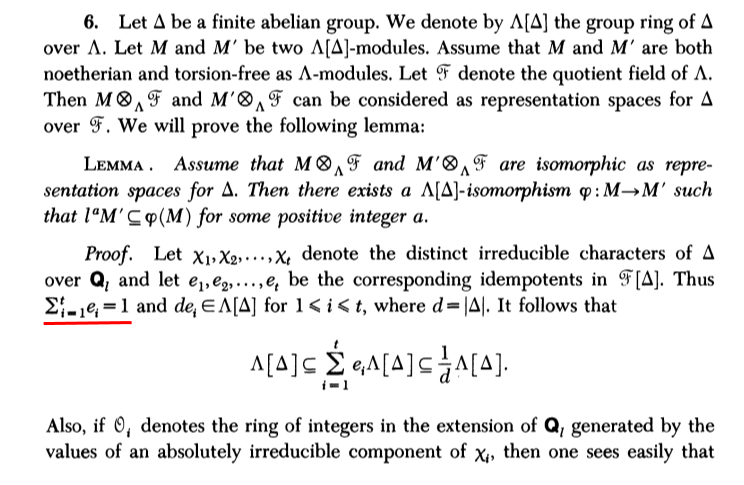
2) Currently, I'm reading the paper "On the Iwasawa Invariants of Totally Real Number Fields" written by Ralph Greenberg. There I cannot understand a line which I have underlined with red line.
I'm afraid that there are many counter-examples against the line.(For example we can take cyclic group of order prime to the order of the group of units.) Can you please explain the line to me?