Summary: I don't think the average $\langle {\rm Tr}(M^n)\rangle \to 0$ for $N\rightarrow\infty$, at least for $n$ even I will argue this average is $\propto\sqrt N$ because of the contributions from eigenvalues on the real axis.
• Consider first the eigenvalues $\lambda_p=r_p e^{i\phi_p}$ of $M$ off the real axis. Because $M$ is real these must come in complex conjugate pairs, $\lambda_p=\lambda^\ast_{N-p}$, so
$${\rm Tr}\, M^n=2\sum_{p=1}^{N/2} r_p^n \cos n\phi_p,$$
and the average
$$\langle{\rm Tr}\,M^n\rangle_{\rm complex}=N\int_0^\infty dr \int_0^{2\pi}d\phi\, P(r,\phi)\, r^{n+1} \cos n\phi.$$
Under the assumption that the eigenvalue density $P(r,\phi)$ in the complex plane is rotationally invariant in the unit disc this average is zero.
• Now turn to the eigenvalues on the real axis. There are $N_{\rm real}=c\sqrt N$ real eigenvalues for $N\gg 1$, where the coefficient $c$ depends on the distribution of $M$. (For a Gaussian $M$ one has $c=\sqrt{2/\pi}$.) The real eigenvalues are uniformly distributed in the interval $(-1,1)$. So their contribution to the average is
$$\langle {\rm Tr}\,M^n\rangle_{\rm real}=c\sqrt{N}\int_{-1}^{1} r^n dr=\begin{cases}
\frac{2c}{n+1}\sqrt{N}&\text{if n is even},\\
0&\text{if n is odd}.
\end{cases}$$
It is likely that the deviations from uniformity in the distribution of complex eigenvalues also give a nonzero contribution of the same order $\sqrt N$ as those from the real axis. (Basically because the eigenvalues that are pinned to the real axis repel nearby eigenvalues with nonzero imaginary part, see plot below.)
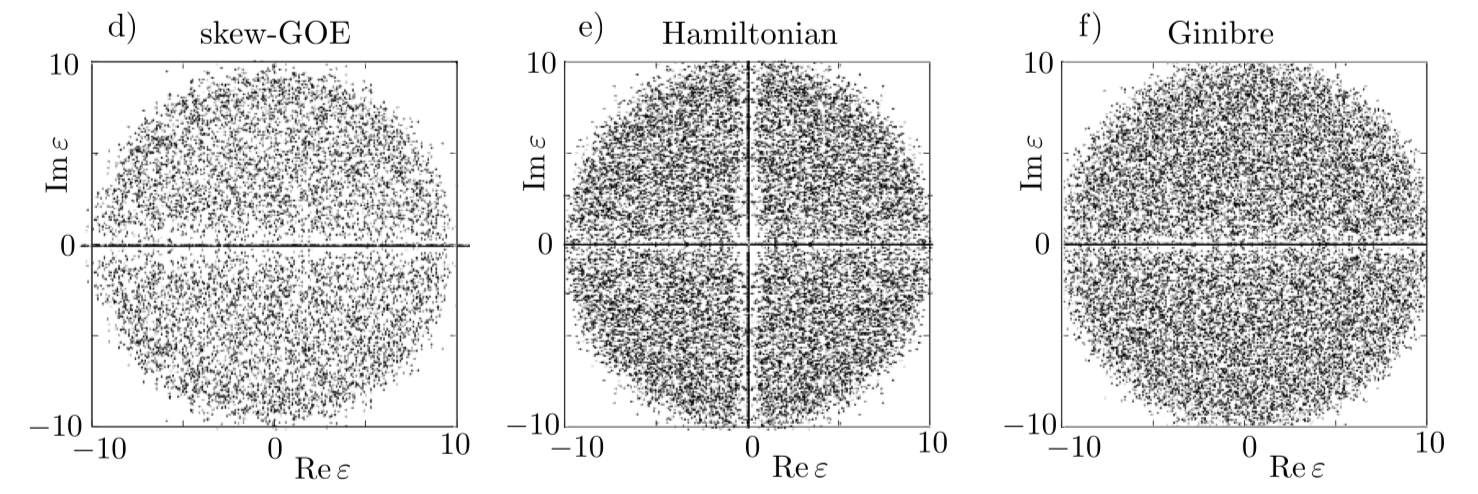
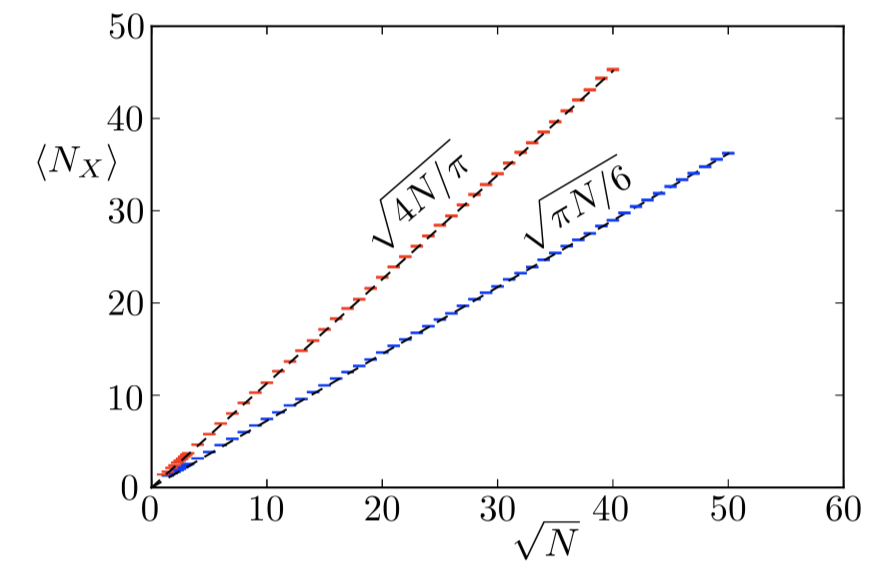
Illustration of the eigenvalue distribution in the complex plane for three different ensembles of real random matrices (of size $N=100$). You can see the eigenvalues pinned to the real axis (they appear as a horizontal black line of dense points), surrounded by a depletion zone (white region of few points). The ensemble of Hamiltonian matrices has an additional symmetry that also pins eigenvalues to the imaginary axis. In each case the number $N_X$ of real eigenvalues scales as $\sqrt N$, with a different numerical prefactor (red line for Hamiltonian ensemble, blue for skew-GOE, Ginibre has $\sqrt{2N/\pi}$).
Figures from arxiv:1305.2924.


