12
$\begingroup$

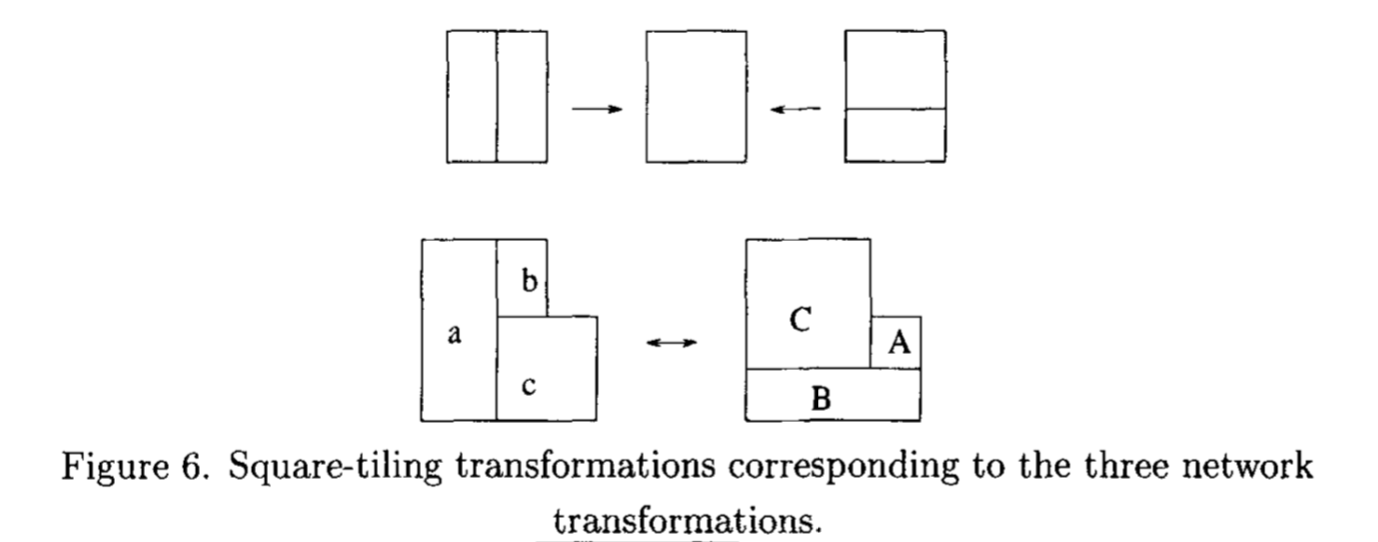
There is a simple discrete combinatorial analogue of manifolds and homeomorphisms: Replace manifolds by simplicial complexes and homeomorphisms by Pachner moves. Equivalence classes of manifolds under homeomorphism are in one-to-one correspondence with equivalence classes of simplicial complexes under Pachner moves (not mentioning technical details, e.g. that we should add "piece-wise linear" to both manifolds and complexes).

A very similar construction can be made for spin manifolds and spin homeomorphisms: In e.g. https://arxiv.org/abs/1505.05856 they give a discrete representant of the second Stiefel-Whitney class for $3$ dimensional simplicial complexes as a $1$-cycle in the complex. Then a discrete representation of the spin structure is given by a $2$-cycle that has this $1$-cycle as boundary.

My question: Is there any construction similar in spirit for manifolds and conformal maps (in $2$ dimensions)? I.e. is there any kind of $2$ dimensional combinatorial structure and a set of local moves such that equivalence classes of the combinatorial structure under local moves correspond to equivalence classes of manifolds under conformal maps?

One could for example still use simplicial complexes and restrict the set of moves such that a combinatorial analogue of "angles are preserved" holds, or add a new kind of structure to the combinatorial description as in the case of spin structures above.

Improve this question
$\endgroup$
1
  • 3
    $\begingroup$ Circle-packing has proven to be the natural discrete analogue of conformal transformations, which in the limit yield the Riemann conformal map. $\endgroup$
    – Thomas Kojar
    Commented Jul 9, 2018 at 21:26

3 Answers 3

Reset to default
8
$\begingroup$

There are various ways of putting additional data on a fixed 2-complex and then defining a notion of "discrete conformal equivalence" between different assignments of data, see e.g. the answers to this question or the field of circle packing (mentioned in the comment of Thomas Kojar and the answer of Adam P. Goucher).

In some of these settings there do indeed exist local transformations which allow you to change the combinatorics of the 2-complex; e.g. one can subdivide triangles in a circle packing or perform star-triangle transformations on certain types of discrete Riemann surfaces, e.g. isoradial graphs or "surfel" surfaces.

A bit more classically, tilings of rectilinear polygons by rectangles (à la Brooks, Smith, Stone, Tutte) can be viewed in this light as well (see e.g. "Squaring rectangles" by Cannon, Floyd and Parry). The rough idea is that rectangle tilings can be constructed from currents and potential differences in a resistor network; the potentials in a resistor network and the currents form a pair of conjugate harmonic functions. Then the classical electrical equivalence moves (including the "original" star-triangle transformation) lead to local transformations of square tilings which change the combinatorics; here are two figures from Kenyon's "Tilings and discrete Dirichlet problems".

First, a depiction of the transformations of the underlying resistor network:

electrical circuit transformations

And their realization as transformations of the rectangle tiling:

rectangle tiling transformations

There are likely more examples. Unfortunately I don't know of an overarching framework which captures this phenomenon (nor even of an exhaustive survey), as this is a rather broad field with influences from conformal geometry, combinatorics, statistical physics, and computer graphics. The references given above are by no means meant to be complete or even representative.

EDIT (6 Nov 2018): I recently saw a set of course notes "Conformal Geometry of Simplicial Surfaces" by Keenan Crane which looks to be a very nice overview of various approaches to discrete conformal geometry.

Improve this answer
$\endgroup$
3
  • 2
    $\begingroup$ Mercat's thesis to provide addition to the surfel paper. $\endgroup$
    – AHusain
    Commented Jul 9, 2018 at 22:18
  • $\begingroup$ Thanks a lot, this is already very close to what I had in mind! The only thing that still bothers me is that all the constructions you give seem to involve putting real (i.e. continous) variables on top of some combinatorial structure. Do you see any possibility to somehow also discretize those continous variables, such that we are left with a purely combinatorial structure? $\endgroup$
    – Andi Bauer
    Commented Jul 28, 2018 at 17:22
  • $\begingroup$ @AndiBauer I'm not sure if this is what you want, but under certain circumstances the combinatorics determines the conformal equivalence class of one of these surfaces. See e.g. this paper on circle packing tori ams.org/journals/proc/2011-139-07/S0002-9939-2010-10690-1 $\endgroup$
    – j.c.
    Commented Jul 28, 2018 at 17:54
4
$\begingroup$

There is an approach to defining a suitable notion of holomorphic functions on the discrete lattice and this is related to rigorous work on 2d conformal field theory by probabilists.

I recommend looking at the slides by Kalle Kytola at the recent Columbia workshop on constructive quantum field theory. It is an update on his joint results with Hongler and Viklund form this preprint.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks, already had those notes open in the neighboring tab ;) This is nice but not what I'm looking for: They give a representation of holomorphic functions as functions from the lattice to $\mathbb{C}$. What I'm looking for is holomorphic functions as functions "from lattice to lattice", or more precisely, a set of local moves such that deformations of the lattice under a sequence of such moves is a discrete analogue of a conformal transformation on the manifold. $\endgroup$
    – Andi Bauer
    Commented Jul 9, 2018 at 21:27
  • 2
    $\begingroup$ @AndiBauer: I'm not sure that what you are looking for exists at the moment. From a categorical point of view conformal maps would be some morphisms between objects corresponding to conformal structures. What would such a discrete conformal structure be? You have in mind a kind of triangulation of a manifold plus some extra data. I don't know what that is. Another idea in this vein is to use circle packings. $\endgroup$
    – Abdelmalek Abdesselam
    Commented Jul 9, 2018 at 21:32
3
$\begingroup$

Thurston produced a constructive discretisation of the Riemann mapping theorem which proceeds as follows: given a region $D$, approximate it by densely packing circles into its interior (as a fragment of hexagonal lattice), then transform the resulting arrangement to fill a disc (by using the circle packing theorem). Wikipedia illustrates this better than I can:

Image courtesy of Oded Schramm

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Indeed, this is the Rodin-Sullivan theorem (conjectured by Thurston). $\endgroup$
    – j.c.
    Commented Jul 9, 2018 at 23:38

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .