5
$\begingroup$

Let $K$ be a p-adic local field, $X$ a smooth projective variety over $Spec(K)$, $CH^k(X)$ the Chow group of pure codimension $k$. Then there are cycle maps

$cl^X_{DR}:CH^k(X)\to H^{2k}_{DR}(X/K)$ and $cl^X_{et}:CH^k(X)\to H^{2k}_{et}(X,\mathbb{Q}_p)$.

There is also a comparison morphism $\alpha$ which comes from p-adic Hodge theory:

$\alpha\colon B_{dR} \otimes_K H^*_{dR}(X/K) \cong B_{dR}\otimes_{\mathbb{Q}_p} H^*_{et}(X\times_K \overline{K},\mathbb{Q}_p).$

Question:

(1) Are these two cycle class maps related via the comparison morphism $\alpha$? This would be analogous to what happens in the complex case: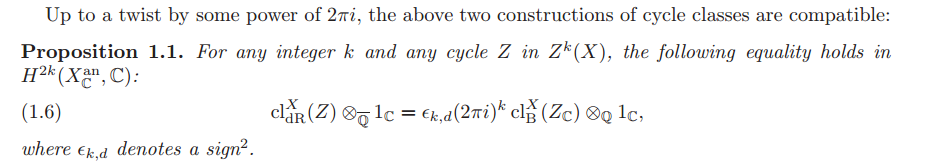enter image description here (Perhaps Fontaine's $t\in B_{dR}$ appears?)

(2) If (1) is too difficult, I would be interested in just the case where $X=Y\times Spec(K)$ where $Y$ is defined over a number field $E$ and $K$ is a $p$-adic completion of $E$.

Thanks for your help.

Improve this question
$\endgroup$
10
  • $\begingroup$ Is there a reason you wrote the first cycle class map having $Z^k(X)$ instead of $\operatorname{CH}^k(X)$ as its domain? $\endgroup$
    – R. van Dobben de Bruyn
    Commented May 7, 2018 at 19:58
  • 3
    $\begingroup$ You should look at Niziol's paper "On Uniqueness of p-adic period morphisms." In particular, she shows that comparison theorems are compatible with Chern classes. $\endgroup$
    – Jesse Silliman
    Commented May 7, 2018 at 21:17
  • 1
    $\begingroup$ @JesseSilliman: that paper looks a bit fancy. I feel that this shouldn't involve studying Chern classes on higher $K$-theory. My strategy would be similar, though: replace $\operatorname{CH}^*(X)$ by $K_0(X)$ (they agree after tensoring with $\mathbb Q$), and use the splitting principle to reduce to the case of $c_1(\mathscr L)$ for $\mathscr L$ a line bundle. For this case an actual argument is needed, though. $\endgroup$
    – R. van Dobben de Bruyn
    Commented May 7, 2018 at 21:30
  • 1
    $\begingroup$ I think that one can deduce that $\alpha$ respects the cycle class maps up to a scalar purely from the functorial properties of the comparison isomorphism. For $X=\mathbb{P}^1$ the element $\alpha(1\otimes c_1^{dR}(O(1)))$ is stable under the Galois action. Hence, $\alpha(1\otimes c_1^{dR}(O(1)))=t\otimes a\cdot c_1^{\'et}(O(1))$ for some $a\in\mathbb{Q}_p$. For any curve $C$ considering a finite cover $C\to\mathbb{P}^1$ we get that $\alpha$ is the multiplication by $at$ on the second cohomology of $C$. $\endgroup$
    – SashaP
    Commented May 9, 2018 at 16:33
  • 1
    $\begingroup$ Finally, for any line bundle $\mathcal{L}$ on a smooth proper $X$ we can conclude that $\alpha(1\otimes c_1^{dR})(\mathcal{L})=t^{-1}\otimes a\cdot c_1^{\'et}(\mathcal{L})$ since the class of $\mathcal{L}$ in the Neron-Severi group modulo torsion is completely determined by the restriction of $\mathcal{L}$ to all curves. However, to compute $a$ it is necessary to look at the actual construction of the comparison isomorphism which you have in mind. $\endgroup$
    – SashaP
    Commented May 9, 2018 at 16:33

0

Reset to default

You must log in to answer this question.