$\newcommand{\de}{\delta} \newcommand{\De}{\Delta} \newcommand{\ep}{\epsilon} \newcommand{\ga}{\gamma} \newcommand{\Ga}{\Gamma} \newcommand{\la}{\lambda} \newcommand{\si}{\sigma} \newcommand{\Si}{\Sigma} \newcommand{\thh}{\theta} \newcommand{\R}{\mathbb{R}} \newcommand{\F}{\mathcal{F}} \newcommand{\E}{\operatorname{\mathsf E}} \newcommand{\PP}{\operatorname{\mathsf P}} \newcommand{\ii}[1]{\operatorname{\mathsf I}\{#1\}}$
One wants to estimate $n$ unknown real numbers $x_1,\dots,x_n$. Toward this end, one can measure $n$ linear combinations \begin{equation} a_{11}x_1+\dots+a_{1n}x_n,\ \dots,\ a_{n1}x_1+\dots+a_{nn}x_n \end{equation} of $x_1,\dots,x_n$ with coefficients $a_{ij}\in\{-1,0,1\}$. Each measurement involves a random error, which has mean zero and the same standard deviation $\si\in(0,\infty)$; all measurements are independent or, more generally, non-correlated. So, one knows the values of the coordinates $y_1,\dots,y_n$ of the random column vector \begin{equation} y:=Ax+\xi, \end{equation} where $A$ is the $n\times n$ matrix with entries $a_{ij}$, $x:=[x_1,\dots,x_n]^T$, and $\xi=[\xi_1,\dots,\xi_n]^T$ is the column vector of the errors of the $n$ measurements.
Thus, one obtains the unbiased estimate \begin{equation} \hat x:=A^{-1}y=x+A^{-1}\xi \end{equation} of $x$, provided that the matrix $A$ is nonsingular; the unbiasedness means that $\E\hat x=x$. The covariance matrix of the estimation error $A^{-1}\xi$ is \begin{equation} \E A^{-1}\xi\xi^T(A^{-1})^T=\si^2(A^T A)^{-1}=:(b_{A;\,ij})_{i,j=1}^n, \end{equation} so that the standard error of this estimation of $x_i$ is $\sqrt{b_{A;\,ii}}$.
The question is this:
What can be said about \begin{equation} \si_n^2:=\min\big\{\max_1^n b_{A;\,ii}\,\colon A\in\{-1,0,1\}^{n\times n},\ A\text{ is nonsingular}\big\}, \end{equation} the minimum of $\max\limits_1^n\mathsf{Var}\,\hat x_i$ over all choices of nonsingular $n\times n$ matrices $A$ with entries $a_{ij}\in\{-1,0,1\}$?
For small enough $n$, the problem can be solved by direct calculation. In particular, for $n=2$ we have $\si_n^2=\si^2/2$ -- attained e.g. at $A=\begin{bmatrix}1&1\\1&-1\end{bmatrix}$. For $n=3$, $\si_n^2$ is $\si^2/2$ as well -- attained e.g. at $A=\begin{bmatrix}1&1&1\\1&1&-1\\1&-1&0\end{bmatrix}$ and at $A=\begin{bmatrix}1&1&1\\1&1&-1\\1&-1&1\end{bmatrix}$; the first of these two choices of $A$ may be considered a better one, because for it $\{b_{11},b_{22},b_{33}\}=\{\frac38,\frac38,\frac12\}\si^2$, whereas for the latter choice of $A$ we have $\{b_{11},b_{22},b_{33}\}=\{\frac12,\frac12,\frac12\}\si^2$.
Of course, choosing $A=I_n$, we see that $\si_n^2\le\si^2$ for all $n$. It would be interesting to find the asymptotics of $\si_n^2$ as $n\to\infty$.
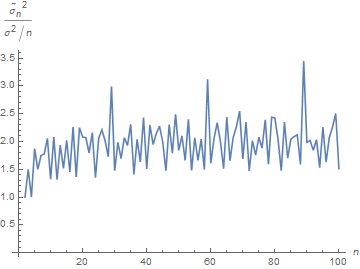
Consider the (hopefully nearly orthogonal) imitation of the Rademacher orthogonal system given by formula \begin{equation} a_{n,s;ij}=\text{sgn}_s\,\sin\frac{\pi i j}{n+\pi/3} \end{equation} for $i,j=1,\dots,n$, where $\text{sgn}_s\,x:=\ii{|x|\ge s}\,\text{sign}\,x$ and $\ii\cdot$ denotes the indicator function. Then we have the following (connected) plot of the points $\Big(n,\dfrac{\tilde\si_n^2}{\si^2/n}\Big)$ for $n=2,\dots,100$, where \begin{equation} \tilde\si_n^2:=\max_1^n b_{A_{n,0.2};\,ii} \end{equation} and $A_{n,s}$ is the $n\times n$ matrix with entries $a_{n,s;ij}$.
So, one may conjecture that $\si_n^2$ behaves as $\si^2/n$ for large $n$, possibly with an extra logarithmic factor. That would be an (almost) $n$ times as good as with the "naive" choice $A=I_n$.
This question was sparked by the somewhat similar one at Optimal linear measurement operator, which in turn was inspired by the question at Can I really double my accuracy?, which in turn goes back to Mosteller.