Relationship between the signs of different notions of curvature in complex geometry

Question

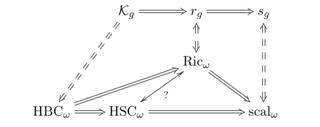
Let $(X,\omega)$ be a complex hermitian manifold, and call $\Theta$ its Chern curvature tensor. Out of this we can consider different notions of curvature, namely the holomorphic bisectional curvature $\operatorname{HBC}_\omega$, the holomorphic sectional curvature $\operatorname{HSC}_\omega$, the Chern-Ricci curvature $\operatorname{Ric}_\omega$, and the Chern scalar curvature $\operatorname{scal}_\omega$ (for definitions, see for instance F. Zheng's excellent book "Complex Differential Geometry").

If we call $(X,g)$ the underlying riemannian manifold, one can also consider the Riemann tensor $R$, and out of this the riemannian sectional curvature $\mathcal K_g$, the Ricci curvature $r_g$, and the scalar curvature $s_g$. Moreover, it is classically known that the sectional curvature completely determines the Riemann tensor.

The two theories above "coincide" if and only if the metric $\omega$ is Kähler. If it is so, it is also classically known that the holomorphic sectional curvature (which contains a priori much less information than the riemannian one) completely determines the Chern tensor, and hence the Riemann tensor.

My question is about how the sign of the different notions of curvature propagates and determines the sign of other types of curvature.

Here is what is more or less straightforward to obtain:

The arrows $\Rightarrow$ in the diagram mean that the positivity (resp. semi-positivity, negativity, semi-negativity) of the source curvature implies the positivity (resp. semi-positivity, negativity, semi-negativity) of the target curvature. These arrows are always valid, even in the non Kähler setting. On the other hand, the dashed arrows are valid in the Kähler case only.

It is however a priori unclear if and how the sign of the holomorphic sectional curvature propagates and determines the signs of the Ricci curvature.

I do know examples showing that some of these arrows are "strict", that is that they cannot be be reversed. These are:

1. $\mathcal K_g\Rightarrow r_g$, $r_g\Rightarrow s_g$;

2. $\operatorname{Ric}_\omega\Rightarrow\operatorname{scal}_\omega$, $\operatorname{HBC}_\omega\Rightarrow\operatorname{Ric}_\omega$, $\operatorname{HSC}_\omega\Rightarrow\operatorname{scal}_\omega$.

3. In addition, it is not difficult to construct examples of Kähler metrics whose Ricci curvature is negative but the holomorphic sectional curvature does not have sign.

So, the following two very basic questions whose answers I don't know (shame on me?), seem to be quite relevant.

Question 1. Is there an example of a (compact) Kähler manifold $(X,\omega)$ such that $\operatorname{HSC}_\omega\le 0$ (or even $<0$) but $\operatorname{HBC}_\omega$ does not have a sign?

Question 2. Is there an example of a (compact) Kähler manifold $(X,\omega)$ such that $\operatorname{HSC}_\omega\le 0$ (or even $<0$) but $\operatorname{Ric}_\omega$ does not have a sign?

Concerning Question 1, I must confess that the only examples of (semi)negative holomorphic sectional curvature I know are given by quotients of bounded symmetric domains, or submanifolds of such quotients, or submanifolds of flat Kähler manifolds. But for these, the holomorphic bisectional curvature does have a sign (semi negative)!

Concerning Question 2, recent breakthroughs by Wu-Yau in the projective case and Tosatti-Yang, Wu-Yau in the compact Kähler case show that if $\operatorname{HSC}_\omega< 0$, then one can always find a (possibly) different Kähler metric $\omega'$ such that $\operatorname{Ric}_{\omega'}< 0$. So, one may rephrase Question 2 asking: do we always really need to change the metric in order to achieve this?

Thank you very much in advance!

Answer 1

As to your first question, I'm interpreting it to include cases where $HSC_\omega >0$ but $HBC_\omega$ does not have a sign. For this, there is a paper by Bo Yang and Fangyang Zheng which finds some examples of metrics on Hirzebruch manifolds with positive holomorphic sectional curvature. However, by the generalized Frankel conjecture (proven by Mok), any compact Kahler manifold with non-negative bisectional curvature must be biholomorphically isometric to a product of tori and projective space. As such, these spaces do not have $HBC_\omega$.

If you're interested in metrics with $HSC_\omega<0$ but where $HBC_\omega$ does not have a sign, it's possible to construct examples defined on tube domains in $\mathbb{C}^n$. However, the metrics I'm aware of with this property are somewhat unsatisfying because they are not complete. I'll fill in more details if I find a better example.