If $n$ tests are independent of each other, and the probability of an event in each test form an arithmetic progression, that is, the probability of the event in the first test is $p$, and in the following tests it is $p + d, p + 2d, p + 3d,\ldots ,p + (n - 1)d$. The question is how to calculate the probability $P(X=k)$ where $X$ is the total number of events which occurs in all of the tests combined.
We use the approximation below to deal with this question,
$$p(X = k) = \left( \begin{array}{l} n \\ k \\ \end{array} \right)q^k (1 - q)^{n - k} ,q = p + \frac{{d(n - 1)}}{2} \, . $$
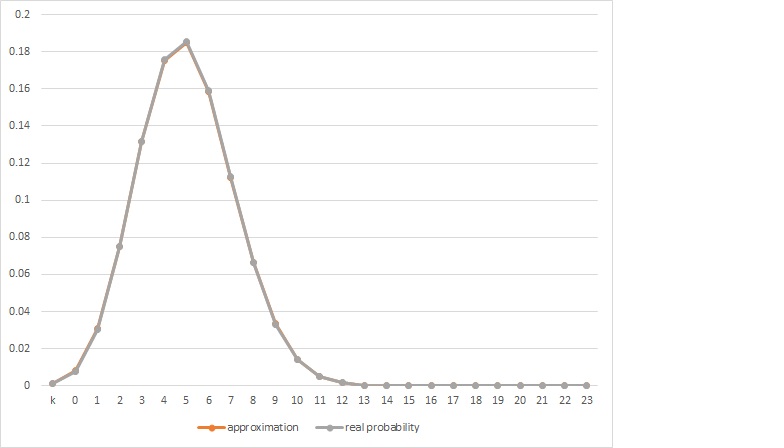
This approximation in fact uses the mean probability. The result as below is somewhat good, However, we don't know how to estimate the deviation/error from the exact probability. I think that the result will be much better if $d/p$ is smaller. The parameters used for the result figure are $n=24,p=0.2,d=0.1/23$. In this situation the result is very good.