6
$\begingroup$

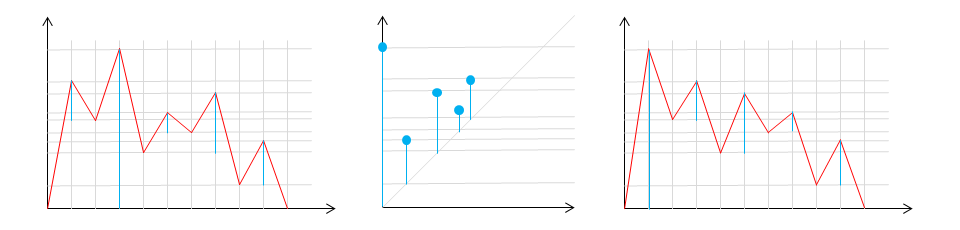
The panels in the figure below show, from left to right:

  • a piecewise affine function with support equal to a bounded interval and an indication of its superlevel filtration;
  • the corresponding persistence diagram;
  • a different function in the same spirit as the first, and with the same persistence diagram.

enter image description here

This example shows that there is a many-to-one mapping between functions and persistence diagrams, even after accounting for an obvious parity symmetry.

However, not every ordering of the blue line segments indicating lifetimes of components is consistent with a filtration of some function. While it's probably not too hard to sort this all out, it seems likely that somebody already has.

So: has the equivalence class of [some class of] functions having the same persistence diagram been considered in the literature? 1D is fine for my considerations.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

Your question is precisely the subject of Justin Curry's recent preprint.

Bottom line: if you agree to identify functions $f,g:[0,1] \to \mathbb{R}$ whenever they have the same merge-tree, then there are only finitely many equivalence classes of functions which produce a given barcode $B$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ A quick glance at it tells me it is hard to sort out the details! Glad I didn't wonder about this a few months earlier $\endgroup$
    – Steve Huntsman
    Commented Oct 6, 2017 at 14:09
  • $\begingroup$ Yes, it takes some work! And I'm not sure if the higher dimensional case is even tractable, since the merge tree is clearly insufficient. $\endgroup$
    – Vidit Nanda
    Commented Oct 6, 2017 at 14:56

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .