(I posted earlier a false solution which I have removed. Sorry for all this).
Perhaps the following is safe(?), while I'll prove the critical special case (discussed earlier by participants in this thread):
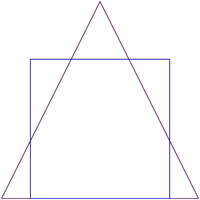
CONJECTURE Let a triangle and a square, both having area $\ 1,\ $ have one edge of each parallel to the other one. Then the intersection of the square and the triangle has an intersection of maximal area for instance when the said edges are contained in the same straight line and both figures are on the same side of that line and the said edges have the same center and the said triangle has the height equal to $\ \sqrt 2\ $ (the @JosephO'Rourke example).
THEOREM If we restrict ourselves to the case of an isosceles triangle such that its base and a square edge are contained in the same straight line, and that the centers of the two edges coincide then O'Rourke's example provides the maximal intersection.
Let me start with an elementary lemma:
Lemma Let positive $\ a\ b\in\Bbb R\ $ be such that $\ a\cdot b=\frac 12.\ $ Then
$$ (1-a)^2 + (1-b)^2\ \ge\ 3-2\sqrt 2 $$
Proof
$$ (1-a)\cdot(1-b)\ =\ \frac 32 - (a+b)\ \le
\ \frac 32 - 2\cdot\sqrt{a\cdot b}\ =
\ \frac 32-\sqrt 2 $$
hence
$$ (1-a)^2 + (1-b)^2\ \ge\ 2\cdot(1-a)\cdot(1-b)
\ \ge\ 3-2\cdot\sqrt 2 $$
End of Proof
Remark Our lemma features equality $\ \Leftrightarrow\ a=b=\frac 1{\sqrt{2}}.$
Proof of the THEOREM (I'll keep applying Tales Theorem).
Let the length of the base be $\ 2\cdot x.\ $ When $\ x=1\ $ then we get the OP Wlodek's example with the intersection area $\ \frac 34.\ $ (We already know that it's not optimal).
When $\ x\ge 1\ $ then the area of the intersection is:
$$ 1\ -\ \left(\frac{x-\frac 12}x\right)^2\ =\ \frac 34\ +
\ \left(\frac 12\right)^2 -\left(\frac{x-\frac 12}x\right)^2 $$
$$ =\,\ \frac 34\ -\ \frac {(x-1)\cdot(3\cdot x-1) } {(2\cdot x)^2}
\,\ \le\,\ \frac 34 $$
for $\ x\ge 1.$ Thus, the situation is not better than for $\ x=1.$
Dually, let's now consider the case of
$\ 0<x\le\frac 12.\ $ Then, the area of the intersection is equal:
$$ 1 - \left(\frac{\frac 1x-1}{\frac 1x}\right)^2
\ =\ 1-(1-x)^2\ = $$
$$ \frac 34 - (x-\frac 12)\cdot(x-\frac 32)\,\ \le
\,\ \frac 34 $$
when $\ 0<x\le\frac 12.$
In the final intermediate case of
$\ \frac 12\le x\le 1,\ $ the area of the intersection is as follows:
$$ 1\ -\ \left(\frac{x-\frac 12}x\right)^2
\ -\ \left(\frac{\frac 1x-1}{\frac 1x}\right)^2\ = $$
$$ 1\ -\ \left(1-\frac 1{2\cdot x}\right)^2
\ -\ (1-x)^2\ \le\ 2\cdot(\sqrt 2 - 1) $$
by lemma. And by Remark, this inequality is actually equality
$\ \Leftrightarrow\ x=\frac 1{\sqrt 2}.$
End of Proof