I want to know is there any interesting properties of this approach or generalization to find $S_k(n)=1^k+2^k+3^k+\cdots+n^k$ by using Pick's Theorem $S=i+\tfrac{b}{2}-1$, where $i$-number of interior points and $b$ - number of boundary points and the following geometric interpretation:
$(\textbf{1})$ - Let consider $S_1(n)$ then for first steps:
\begin{array}{|c|c|c|} \hline n& b_n & i_n \\ \hline 1& 4& 0\\ \hline 2& 8& 0\\ \hline 3& 12& 1\\ \hline 4& 16& 3\\ \hline 5& 20& 6\\ \hline ...& ...& ...\\ \hline n& 4n& \tfrac{(n-1)(n-2)}{2}\\ \hline \end{array}
$(\textbf{2})$ - Let consider $S_2(n)$ then for first steps:
\begin{array}{|c|c|c|} \hline n& b_n & i_n \\ \hline 1& 4& 0\\ \hline 2& 10& 1\\ \hline 3& 18& 6\\ \hline 4& 28& 17\\ \hline 5& 40& 36\\ \hline ...& ...& ...\\ \hline n& n(n+3)& \tfrac{(n-1)(n^2+n-3)}{3}\\ \hline \end{array}
$(\textbf{3})$ - Let consider $S_3(n)$ then for first steps:
\begin{array}{|c|c|c|} \hline n& b_n & i_n \\ \hline 1& 4& 0\\ \hline 2& 14& 3\\ \hline 3& 30& 22\\ \hline 4& 52& 75\\ \hline ...& ...& ...\\ \hline n& n(3n+1)& \tfrac{(n-1)(n+1)(n^2+2n-4)}{4}\\ \hline \end{array} $...$
$(\textbf{k})$ - Let consider $S_k(n)$ :$\textbf{???}$
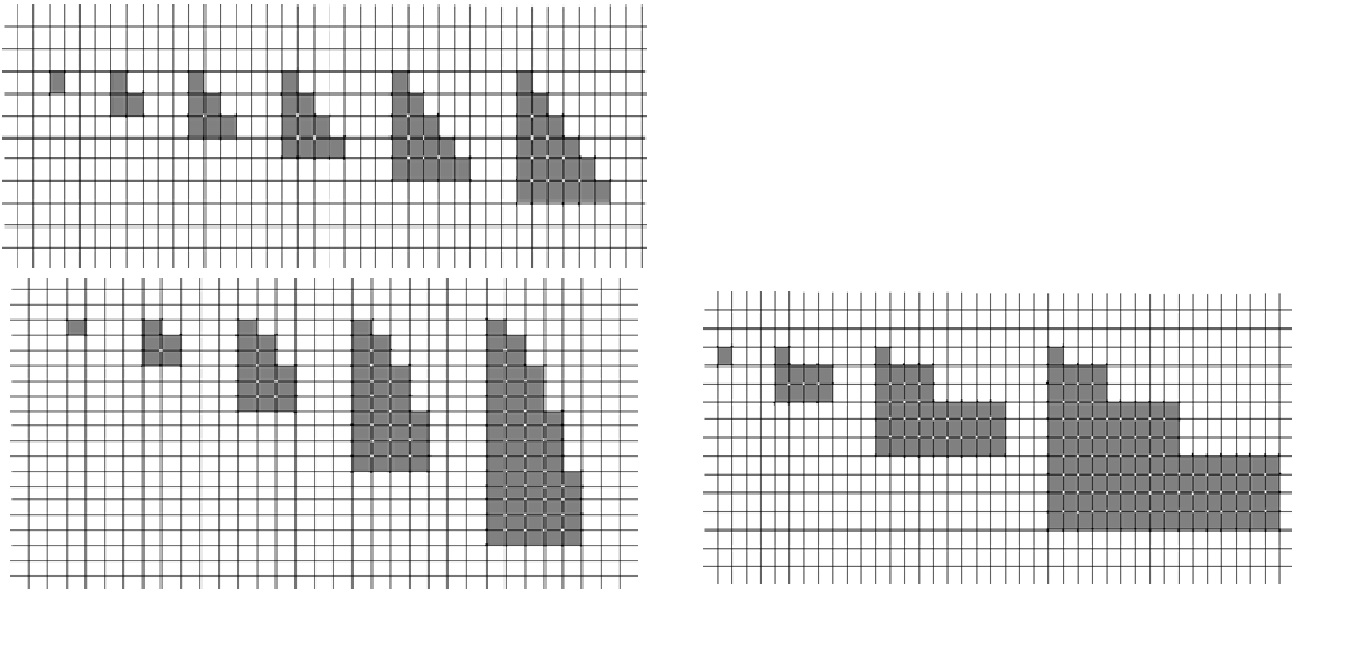So, $\textbf {the questions}$ are: How to find general formulas for $b_n$ and $i_n$ for any step $k$ when we depict our sums as follows: $S_k(n)=1^2\cdot1^{k-2}+2^2\cdot2^{k-2}+3^2\cdot3^{k-2}+...+n^2\cdot n^{k-2}$ - so $n^{k-2}$ times we take $n^2$ and add belows the previous squares? And, the second question: are such constructions involved in any math research or have some interesting properties?
