In light of Tao's discussion of Quantitative Continuity I've decided to post some thoughts on Winding number.
The Jordan curve theorem is non-trivial because closed curves can be very complicated. ( Image taken from Wordpress )
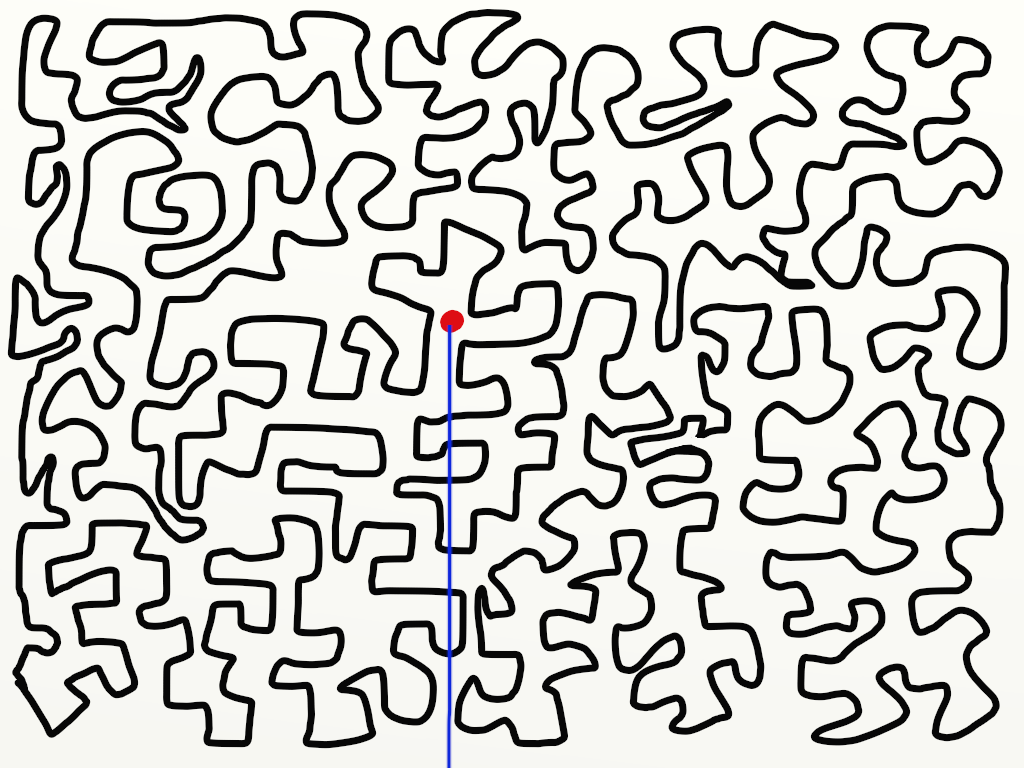
I am going to raise two doubts in this discussion. Mainly the Jordan curve theorem and winding numbers are maybe not robust:
- the red dot as nearby points that inside and other nearby points that are outside.
- if I erase a small segment maybe (maybe on the outside) the curve
Despite being an open curve, I may not have the infinite time or resources to compute a way out. Is there any way to formalize this notion of approximate winding number?
For an open curve we no longer have that the winding number is an integer can we have:
$$ \int_\gamma d \big( \log \, z \big) \in \mathbb{Z} + [-\epsilon, \epsilon] $$
for some small number $\epsilon > 0$. This may still not be a good measure of the ``tangledness" of the curve surrounding the red point.
Another example showing these closed curves can be arbitrary.