I'm studying Robert Ghrist papers on integration against Euler Characteristic. I am particularly interested in the relation with Morse Theory. I am trying to understand the proof of Theorem 25.1 (page 52) of this preprint, which I reproduce in an extended version (the parts I understand):
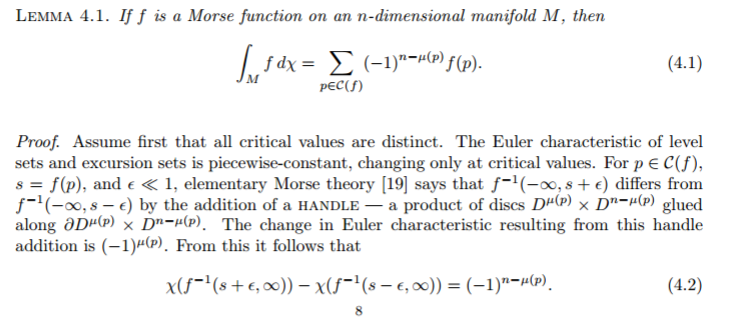
Theorem. Suppose $h\colon M\to \mathbb{R}$ is a function integrable against Euler characteristic and a Morse function on the closed (compact without boundary) manifold $M$. Then:
$$\int_M [h] \: d \chi=\sum_{p\in Cr(h)}(-1)^{n-\mu(p)}h(p)$$
where $Cr(h)$ is the set of critical points of $h$.
Remark. We follow the usual conventions of Milnor's book in Morse theory.
The proof as I understand it. First we use a previous result (Proposition 24.8 of the same paper) which says that:
$$\int_M [h] \: d \chi=\int_{s=0}^{\infty}\chi\{h\ge s\}-\chi\{h<-s\}\: ds.$$
Now, using Theorem 3.2 from Milnor's book I know that $\chi\{h\le s\}$ is piecewise constant and only changes at critical values of $h$. Moreover, the change is due to the addition of a cell of the dimension of the Morse index of that critical value. So, the change in the Euler Characteristic at a critical value $s=h(p)$ is: $$\chi\{h\le s + \epsilon\}-\chi\{h\le s - \epsilon\}=(-1)^{\mu(p)}.$$
At this point I get lost.
So I insert as a picture the proof given in the paper, and a similar proof of the same result in another preprint(page 8):
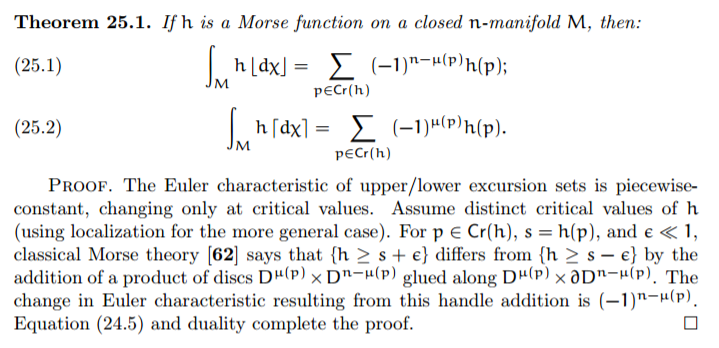
Any help would be appreciated! By the way, there is a proof which involves Stratified Morse Theory, but I am interested in this one using elementary methods.