2
$\begingroup$

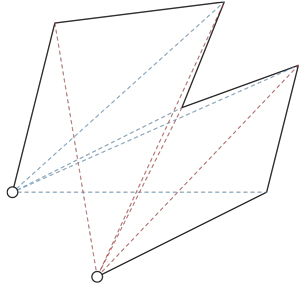
Question:

Is there already a name for polylines in the euclidean plane, that have the property, that no interior of none the triangles, defined by one of the polyline's endpoints and a non-adjacent edge, is not intersected by the polyline?


I am tempted to call that property "bipolar monotonicity" if no other name has been coined yet.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

I haven't seen this named, but you might include either "visibility" or "star" in your name, as it is roughly doubly star-shaped. Perhaps bipolar star?

          2Star

Improve this answer
$\endgroup$
2
  • $\begingroup$ Good idea to introduce binary solar systems into computational geometry. $\endgroup$
    – Manfred Weis
    Commented May 10, 2017 at 21:04
  • 1
    $\begingroup$ Thinking a bit about your suggestion, I guess, I like "bipolar star-shaped" best $\endgroup$
    – Manfred Weis
    Commented May 10, 2017 at 23:34

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .