While I was working on a variational problem, I met this equation as its Euler-Lagrange equation, but I cannot solve it: $ x= \frac{af'(x)}{\sqrt{1+af'(x)^{2}}} + \frac{bf'(x)}{\sqrt{1+bf'(x)^{2}}} \ (a\neq b) $.
1
-
$\begingroup$ For completeness and readers in the future, I would include the Lagrangian, since going backwards to find it is non-trivial. $\endgroup$– JamalSCommented May 4, 2017 at 19:22
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
9
You can solve it parametrically as follows: Write $$ x(t) = \frac{at}{\sqrt{1+at^2}}+\frac{bt}{\sqrt{1+bt^2}} $$ and $$ y(t) = c - \frac{\sqrt{1+at^2}+\sqrt{1+bt^2}}{\sqrt{1+at^2}\sqrt{1+bt^2}} $$ where $c$ is a constant. Then this gives the general solution as $c$ varies.
If you want an explicit relation between $x$ and $y$, you can eliminate $t$, but it won't be pretty.
answered May 4, 2017 at 14:13
-
2$\begingroup$ To see what the solution looks like: desmos.com/calculator/xsuj2he8ts $\endgroup$ Commented May 4, 2017 at 14:33
-
$\begingroup$ @WillieWong: Thanks! That's a neat demo. FYI: The solution curve is algebraic, of course. It might be worth mentioning that it is an algebraic curve in the $xy$-plane of degree 8 in general. $\endgroup$ Commented May 4, 2017 at 14:43
-
2$\begingroup$ We have the following polynomial relations among $x, y, t, u = \sqrt{1+at^2}$ and $v = \sqrt{1+bt^2}$: $u^2 - (1+at^2)=0$, $v^2 - (1+bt^2)=0$, $uvx - but - avt = 0$, $uvy - cuv+u+v=0$. Eliminate $u,v,t$ by computing a "plex" Groebner basis of the ideal generated by the left sides of these equations. The first element of the basis is a rather complicated polynomial of degree $8$ in $x$ and $y$. $\endgroup$ Commented May 4, 2017 at 15:52
-
1$\begingroup$ You might find this graph of the curve in the case $a=1$, $b=2$, $c=3$ interesting. $\endgroup$ Commented May 4, 2017 at 16:03
-
1$\begingroup$ And here is an animation of the curve for $a=1, c=0$ with varying $b$. $\endgroup$ Commented May 4, 2017 at 19:05
$\begingroup$
$\endgroup$
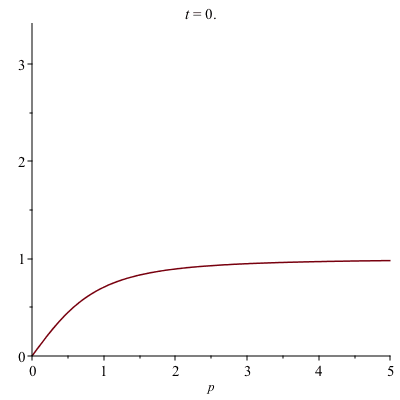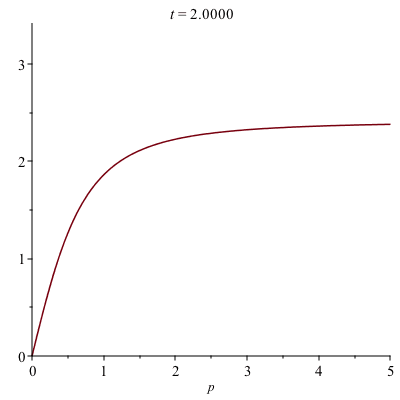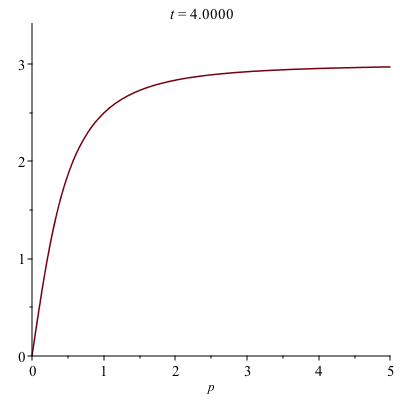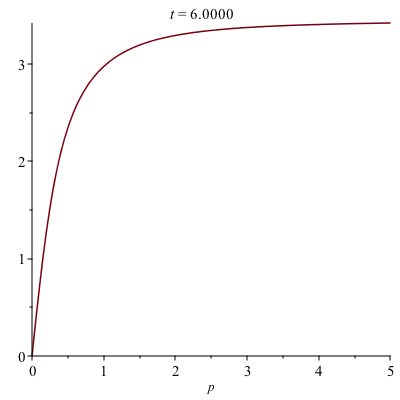
Set $p:=f'(x)$ and $$ \Phi(p)=\Phi_{a,b}:=\frac{ap}{\sqrt{1+ap^2}}+\frac{bp}{\sqrt{1+bp^2}}. $$ The differential equation you wrote can be rewritten as $$ x=\Phi(p). $$ If we could invert $\Phi$, then we could write $$ f'(x)= p=\Phi^{-1}(x). $$ For $a, b>0$ the function $\Phi$ seems to be increasing. The animation below depicts the graphs of $\Phi_{1,t}$ for $t\in [0,6]$
It already shows that the solution blows up in finite time. (Here I think of $x$ as time.)
The next animation depicts $\phi_{1,t}$ for $t=-1..0$ and you can see that the injectivity of $\Phi$ is lost for some values of $t$.
Remark. Here is an animation of the curve described by Robert Bryant for $a=1$ and $b\in [-0.1,0.2]$, $t\in[-3,3]$
answered May 4, 2017 at 14:14