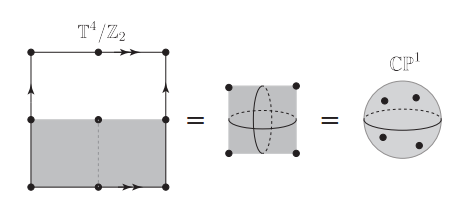
A physics paper [1] states the moduli space of flat $SU(N)$ connections on an elliptic curve $E$ is the projective space $\mathbb{C}P^{N-1}$. However, I need some clarifications:
- the paper vaguely states some sort of "holomorphic" correspondence between flat $SU(N)$ connections on $E$ and $\mathbb{C}P^{N-1}$
- the paper has a disclaimer that the equivalence is only at the level of algebraic varieties, that that metric on the space of connections is not the Fubini-Study metric
- the paper gives an explicit map between $\mathcal{M}_{flat}$ and $\mathbb{C}P^{N-1}$ using theta functions
I am having trouble picturing these flat $SU(N)$ structures. Can they be parameterized by Abelian differentials? or mapped into a Euclidean space?