In short: unlike the mapping between alternating-sign matrices and the configurations of the six-vertex model with domain-wall boundary conditions, the mapping between the six-vertex model and domino tilings is not one-to-one. This accounts for the fact that the relevant special case of the six-vertex model (with $a=b=c/\sqrt{2}$) corresponds to the 2-enumeration of alternating-sign matrices (ASMs).
On the statistical-mechanical side we're dealing with the special case $a=b<c$, known as the F-model, of the six-vertex model.
(Historical aside: today I finally found out that the name "F-model" was chosen by Rys in honour of his thesis advisor, Fierz, who apparently came up with the model.)
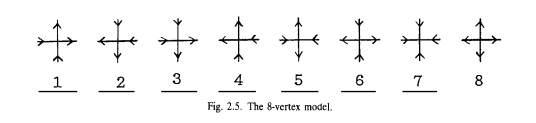
Recall that $c$ is the weight of the two 'saddle-like' arrow configurations on the edges surrounding a vertex: these are vertices 5 and 6 in the OP's figure. The ice rule implies that along any row (and any column) the two vertex configurations of weight $c$ must occur alternatingly, with any number of intermediate vertices of weight $a$ and $b$. The domain-wall boundary conditions further imply that one of the two vertices of weight $c$ occurs less than the other: it must occur precisely one time less in every row and in every column. (Whether this is vertex 5 or 6 in the OP depends on which of the two possible domain-wall boundaries one chooses; the two are related by reversing all arrows.) Of course these facts are precisely what allows one to relate configurations of the six-vertex model with domain walls to ASMs, cf. Kuperberg [arXiv:math/9712207].
On the combinatorial side recall that an $x$-enumeration counts ASMs with weight $x^k$ when the ASM contains $k$ entries equal to $-1$. Now the latter ASM entries precisely correspond to the vertex configuration of weight $c$ that occurs less (cf above). Thus, if a row contains $l$ of these vertices, it must contain precisely $2l+1$ vertices of weight $c$. But this is true for every row of the lattice. The upshot is that if the lattice has size $L\times L$ then the domain-wall partition function of the F-model accounts for the various $x$-enumerations of ASMs:
$$c^L \ Z_L(a=b,c) \quad\longleftrightarrow\quad \text{$c^2$-enumeration of $L\times L$ ASMs} \ . $$
(Since common rescalings of $a,b,c$ only yield a physically unimportant normalization factor for $Z_L$ we can set $a=b=1$ to remove the overall factor in the above correspondence.)
In particular, at the combinatorial or ice point ($a=b=c$) the domain-wall partition function just counts the number of ASMs up to an overall normalization.
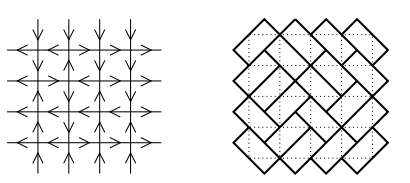
By assigning two domino tiles for every $-1$ in the ASM (as in Fig 1 of Zinn-Justin cited in the OP) we thus get a combinatorial interpretation for the 2-enumeration of ASMs (counted by the domain-wall partition function at $a=b=c/\sqrt{2}$) in terms of the number of domino tilings of the so-called Aztec diamond.
PS. Just to mention some more related terminology consider the combination
$$\Delta=\frac{a^2+b^2-c^2}{2\,a\,b}$$
of vertex weights. For the F-model we have $\Delta = 1-(c/a)^2/2$. The ice-model corresponds to $\Delta =1/2$, the 2-enumeration of ASMs to $\Delta = 0$ and their 3-enumeration to $\Delta=-1/2$. The value $\Delta = 0$ is known as the free-fermion point. That this value is quite special is clear from the viewpoint of the XXZ spin chain related to the six-vertex model, whose Hamiltonian is of the form
$$H_{XXZ} = \sum_{j\in\mathbb{Z}/L\mathbb{Z}} (S_j^x \, S_{j+1}^x + S_j^y \, S_{j+1}^y + \Delta \, S_j^z \, S_{j+1}^z) \ \in \ \text{End}((\mathbb{C}^2)^{\otimes L}) \ ,$$
where $\Delta$ now sets the (partial) anisotropy, breaking the $SU(2)$-symmetry of Heisenberg's isotropic XXX spin chain ($\Delta=1$) to the subgroup $U(1)\subseteq SU(2)$ of rotations around the $z$-axis.