5
$\begingroup$

The spin bordism group for the classifying space $BG$ of group $G$ can be denoted as $\Omega^{Spin}_d(BG)$.

For example, $\Omega^{Spin}_d(pt)$ are computed by Anderson-Brown-Peterson (D. W. Anderson, E. H. Brown, Jr. and F. P. Peterson, The structure of the Spin cobordism ring, Ann. of Math. 86, 271-298 (1967).).

Below we denote the finite cyclcic group of order $n$ as $\mathbb{Z}/n\mathbb{Z}=\mathbb{Z}_n$.

If we take $BG=pt$, one get

$\Omega^{Spin}_1(pt)=\mathbb{Z}_2$, $\Omega^{Spin}_2(pt)=\mathbb{Z}_2$, $\Omega^{Spin}_3(pt)=0$, $\Omega^{Spin}_4(pt)=\mathbb{Z}$

If we take $G=\mathbb{Z}_2$, we get,

$\Omega^{Spin}_1(B\mathbb{Z}_2)=\mathbb{Z}_2^2$, $\Omega^{Spin}_2(B\mathbb{Z}_2)=\mathbb{Z}_2^2$, $\Omega^{Spin}_3(B\mathbb{Z}_2)=\mathbb{Z}_8$, $\Omega^{Spin}_4(B\mathbb{Z}_2)=\mathbb{Z}$

Here are my questions, if we take $G=(\mathbb{Z}_2)^2$, or $G=(\mathbb{Z}_2)^3$, $G=(\mathbb{Z}_2)^4$, what are the answers for the following:

$$\Omega^{Spin}_1(B(\mathbb{Z}_2)^2)=?, \Omega^{Spin}_2(B(\mathbb{Z}_2)^2)=?, \Omega^{Spin}_3(B(\mathbb{Z}_2)^2)=?, \Omega^{Spin}_4(B(\mathbb{Z}_2)^2)=?$$

$$\Omega^{Spin}_1(B(\mathbb{Z}_2)^3)=?, \Omega^{Spin}_2(B(\mathbb{Z}_2)^3)=?, \Omega^{Spin}_3(B(\mathbb{Z}_2)^3)=?, \Omega^{Spin}_4(B(\mathbb{Z}_2)^3)=?$$

$$\Omega^{Spin}_1(B(\mathbb{Z}_2)^4)=?, \Omega^{Spin}_2(B(\mathbb{Z}_2)^4)=?, \Omega^{Spin}_3(B(\mathbb{Z}_2)^4)=?, \Omega^{Spin}_4(B(\mathbb{Z}_2)^4)=?$$

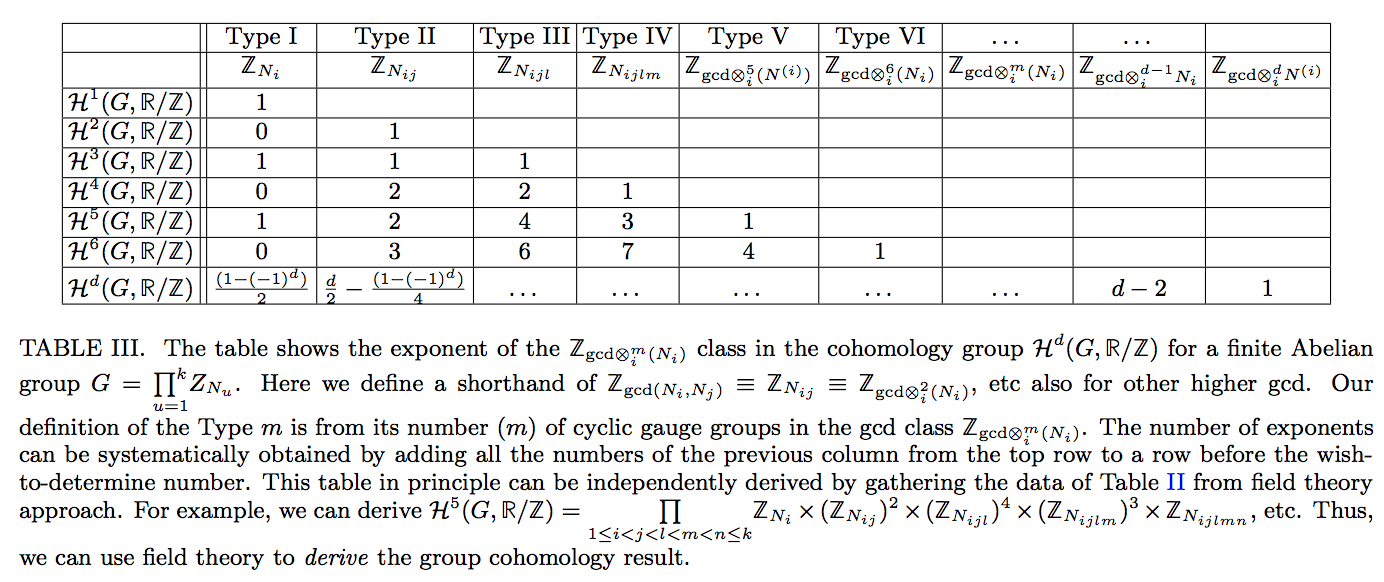
P.S. Part of bordism group data may overlap with the group (co)homology data $H_d[G,\mathbb{Z}]$ or $H_d[G,\mathbb{R}/\mathbb{Z}]$, $H^d[G,\mathbb{Z}]$ or $H^d[G,\mathbb{R}/\mathbb{Z}]$. Fortunately, a Ref here in SUPPLEMENTAL MATERIAL shows the useful data in a table:

enter image description here

Any partial answers and any Refs are welcome.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Can you include what Atiyah-Hirzebruch gives you so far? $\endgroup$
    – AHusain
    Commented Dec 14, 2016 at 21:57
  • $\begingroup$ Thanks for the nice comment. There should be some recursion relation for the spin bordism group, here of the product of cyclic group. But I could not see it very easily. $\endgroup$
    – miss-tery
    Commented Dec 14, 2016 at 22:09
  • $\begingroup$ Other G will nicely clear up on this page, but I suppose that is maximally unhelpful. $\endgroup$
    – AHusain
    Commented Dec 14, 2016 at 22:13
  • 1
    $\begingroup$ AHusain is right, the standard method would be to use the Atiyah-Hirzebruch spectral sequence. The input on the $E^2$-page will be the homology (either mod 2 or integral, in these low dimensions) of these groups $G$. Since they are elementary abelian 2-groups, their homology should be easy to look up or calculate. $\endgroup$
    – Mark Grant
    Commented Dec 15, 2016 at 9:53

1 Answer 1

Reset to default
8
$\begingroup$

The paper of Anderson, Brown and Peterson also shows that the localisation of the spectrum $MSpin$ splits as a wedge of suspensions of the real connective $K$-theory spectrum $kO$ and various closely related spectra. It is also a theorem of Hopkins and Hovey that $KO_*(X)=KO_*\otimes_{MSpin_*}MSpin_*(X)$. This shows that the calculation of the groups $\Omega^{spin}_*(BG)=MSpin_*(BG)$ is very closely related to the calculation of the groups $kO_*(BG)$ and $KO_*(BG)$. Greenlees, Bruner and various collaborators have written extensively about this kind of question. However, the answers are often very complicated.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .