This is the definition given in Vistoli's paper.
Let $F$ be an integral stack. A rational function of $F$ is a morphism $G \rightarrow A^1_S$ defined on a nonempty open substack $G$ of $F$.
Rational functions can be added and multiplied, and they form a field $K(F)$, the quotient field of $F$.
Then he gives a simple example. A rational function on $[X/G]$ is an invariant rational function on $X$.
I have no trouble understanding this. But it's hard for me to think about the general case. I think a rational function is defined on some atlas $U$ over some open substack of $F$ and satisfies certain gluing conditions.
Can someone give some other examples which are not quotient stacks?
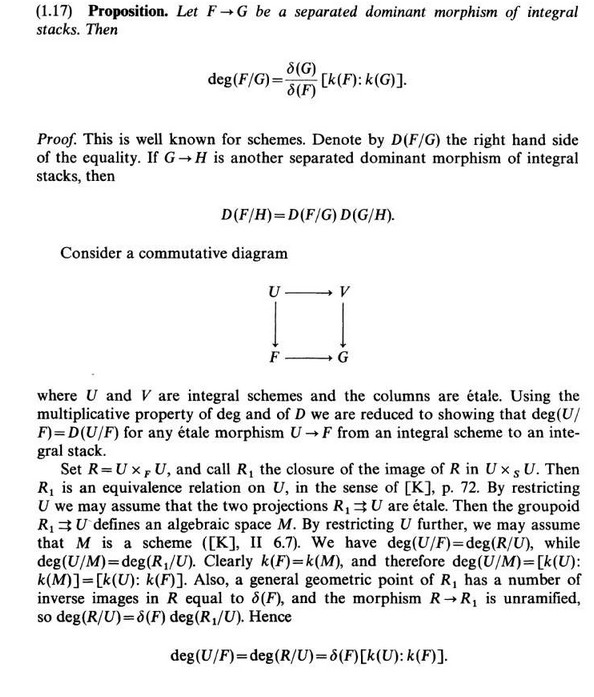
Also in his proof of the proposition 1.17, I don't understand why $k(F)=k(M)$.