Can you prove Givental's conjecture on wavefronts and the icosahedron?

Question

In his remarkable book The Theory of Singularities and its Applications, Vladimir Arnol'd discussed a conjecture of A. B. Givental, which asserts that the symmetry group of the icosahedron is secretly lurking in the problem of finding the shortest path from one point to another in a region of the plane. Via Huyghen's principle, this problem is connected to the motion of waves in such a region.

Arnol'd nicely expresses the awe mathematicians feel when they discover connections like this:

Thus the propagation of waves, on a 2-manifold with boundary, is controlled by an icosahedron hidden at an inflection point at the boundary. This icosahedron is hidden, and it is difficult to find it even if its existence is known.

Unfortunately, even now a full proof of Givental's conjecture seems hard to find! Can you find one, or give one?

Let me sketch the idea here. For more details, see:

• Involutes of a cubical parabola, Visual Insight, 1 May 2016.

• Discriminant of the icosahedral group, Visual Insight, 15 May 2016.

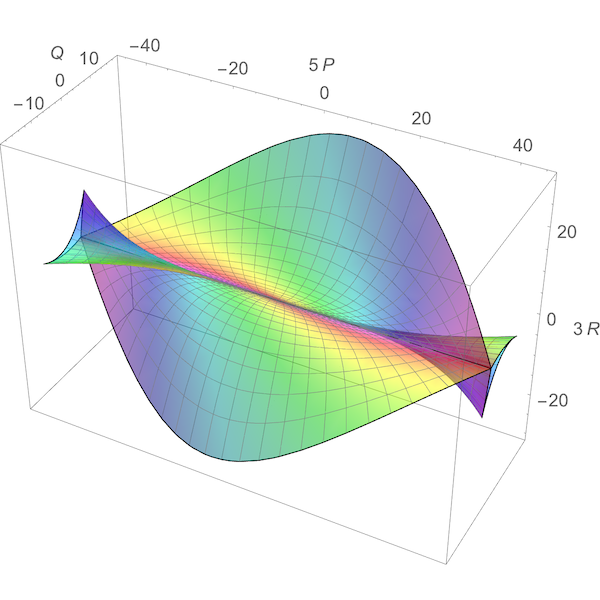
This image, created by Greg Egan, shows the discriminant of the symmetry group of the icosahedron, a 120-element group known as $\mathrm{H}_3$.

This group acts as linear transformations of $\mathbb{R}^3$, and thus also $\mathbb{C}^3$. By a theorem of Chevalley, the space of orbits of this group action is again isomorphic to $\mathbb{C}^3$. Each point in the surface shown here corresponds to a nongeneric orbit: an orbit with fewer than the maximal number of points. More precisely, the space of nongeneric orbits forms a complex surface in $\mathbb{C}^3$, called the discriminant of $\mathrm{H}_3$, whose intersection with $\mathbb{R}^3$ is shown above.

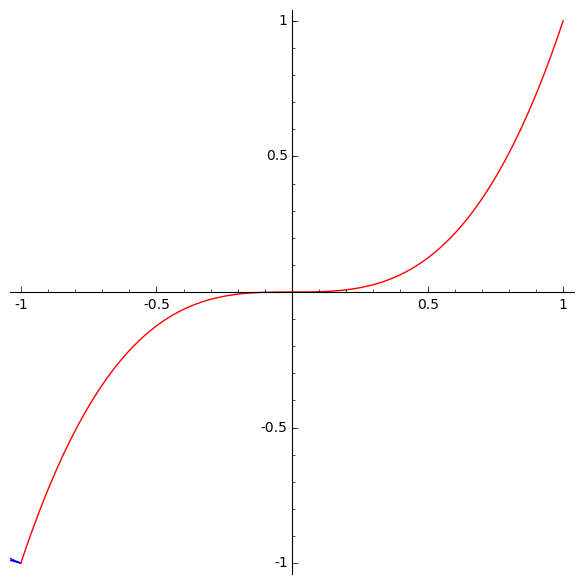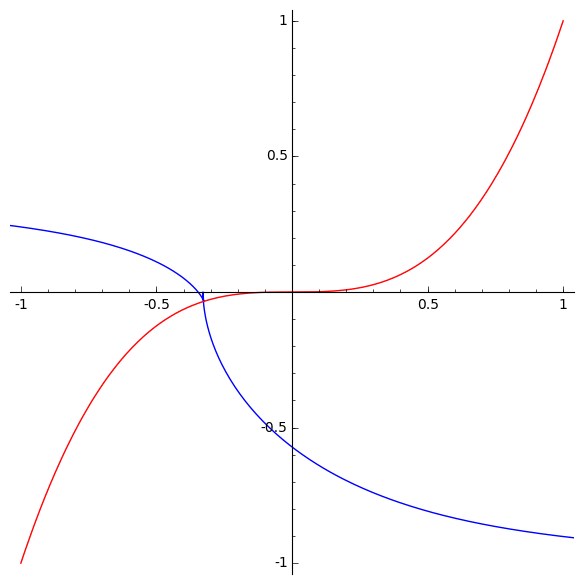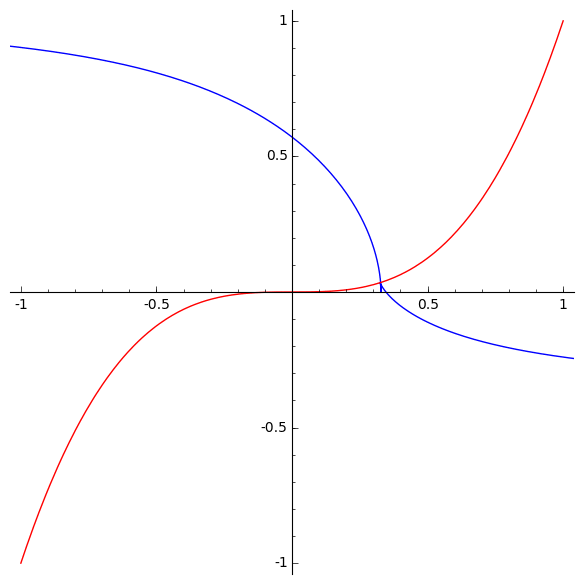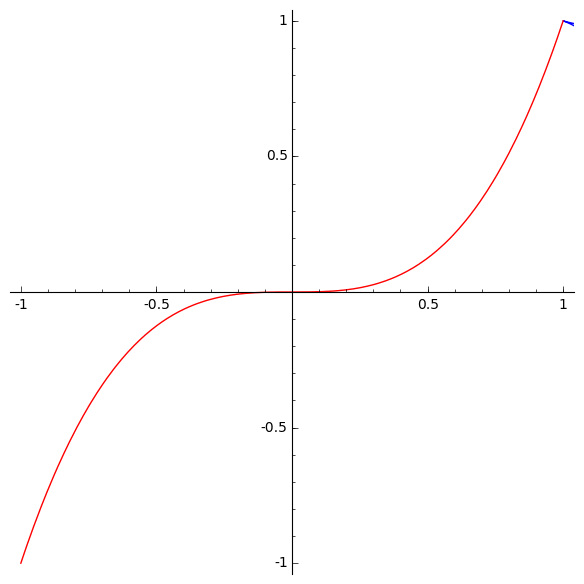
The following image, created by Marshall Hampton, shows the involutes of the curve $y = x^3$:

Loosely speaking, an involute of a plane curve $C$ is a new plane curve $D$ obtained by attaching one end of a taut string to a point $p$ on $C$ and tracing the path of the string's free end as you wind the string onto $C$. There are different involutes for different choices of $p$ and different lengths of string. I am ignoring some important nuances here, some of which are discussed on my Visual Insight post.

But here's the point: the involutes, shown in blue, look like slices of the discriminant of the icosahedral group!

Indeed, Givental conjectured this is true, and Arnol'd says it's been proved. In The Theory of Singularities and its Applications, he wrote:

The discriminant of the group $\mathrm{H}_3$ is shown in Fig. 18. Its singularities were studied by O. V. Lyashko (1982) with the help of a computer. This surface has two smooth cusped edges, one of order 3/2 and the other of order 5/2. Both are cubically tangent at the origin. Lyashko has also proved that this surface is diffeomorphic to the set of polynomials $x^5 + ax^4 + bx^2 + c$ having a multiple root.

The comparison of this discriminant with the patterns of the propagation of the perturbations on a manifold with boundary (studied as early as in the textbook of L'Hopital in the form of the theory of evolutes of plane curves), has led A. B. Givental to the conjecture (later proven by O. P. Shcherbak) that this discriminant it locally diffeomorphic to the graph of the multivalued time function in the plane problem on the shortest path, on a manifold with boundary, which is a generic plane curve.

(Figure 18 is a hand-drawn version of the picture at the top of this post.)

This seems like an exciting claim worthy of a nice conceptual proof. Unfortunately, I haven't been able to find a complete proof the literature, not even in these promising-looking papers:

• O. P. Shcherbak, Singularities of a family of evolvents in the neighbourhood of a point of inflection of a curve, and the group $\mathrm{H}_3$ generated by reflections, Funktsional. Anal. i Prilozhen. 17:4 (1983), 70–72. English translation in Functional Analysis and its Applications 17:4 (1983), 301–303; free version available here.

• O. P. Shcherbak, Wavefronts and reflection groups, Uspekhi Mat. Nauk 43:3 (1988), 125–160. English translation in Russian Mathematical Surveys 43:3 (1988), 1497–194.

The Russian versions are open-access; the English versions are not, but the first paper is currently available for free online.

Perhaps an expert could construct a full proof based on the ideas in these papers! For more clues and references, see my Visual Insight posts.

Answer 1

In http://www.sciencedirect.com/science/article/pii/S0167278998900057 (Remarks on quasicrystallic symmetries) Arnold so describes the idea of Shcherbak's proof:

Now the proof of this theorem depends on the relation of the icosahedron symmetry group $H_3$ to the so-called crystallographic (or Weyl) group $D_6$ (associated with the simple Lie algebra $D_6\approx O(12)$ and with the simple hypersurface singularity $x^2y+y^5+z^2$).

The Weyl group $D_6$ is a reflection group, acting in an Euclidean 6-space and conserving a crystallographic lattice (the set of integer linear combinations of 6 independent vectors). In his proof of the above stated theorem, Scherbak constructed a decomposition of this 6-space into two orthogonal 3-spaces, invariant under the two irreducible real representation of the isohedral symmetry group $H_3$ in $R^3$.

These two 3-spaces are irrational with respect to the $D_6$ integer lattice. The restrictions of the $D_6$-periodic functions to these irrational subspaces are the desired quasiperiodic functions in 3 variables having icosahedral symmetries.

Arnold cites Shcherbak's paper http://link.springer.com/article/10.1007%2FBF01076721 (can be found also here http://www.inp.nsk.su/~silagadz/Shcherbak.pdf).

For me it is rather cryptic how what Arnold writes is related to the content of the Shcherbak's paper but hope it will be clear for experts in the field.

Maybe the following well-illustrated review (in French) https://eudml.org/doc/110042 (Caustique mystique, by Daniel Bennequin) will be also somewhat useful.