natural weak factorization systems

Question

I am trying to understand the definition of natural weak factorization systems from this article by Tholen and Grandis, and these notes by Emily Riehl.

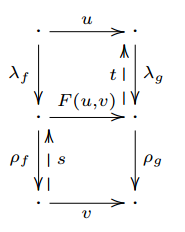
In Riehl's notes, the splitting $s,t$ are of $\rho_f,\lambda_g$, and it is explicitly shown (see diagram below) how their existence enables us to solve the lifting problem for $(f,g)$ to prove $f\pitchfork g$. On the other hand, Riehl does not go into much detail about how a natural choice of such splittings gives rise to (co)monads.

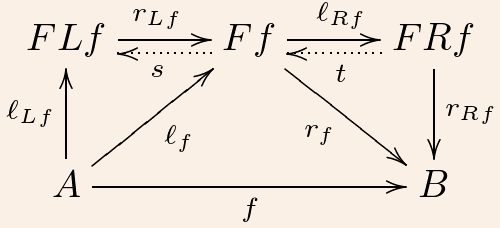
Tholen and Grandis's paper, on the other hand, looks at splittings $s,t$ of $\rho_{Lf},\lambda_{Rf}$. They just on to say these splitings are needed for constructing liftings, but I don't understand how. For instance, everything here is part of the data around the arrow $f$ (see diagram below) so how can it solve lifting problems?

So both papers discuss the significance of having a natural choice of splittings, but for different arrows, even though both seem to say their splittings are used to solve lifting problems (show weak orthogonality). Riehl shows this explicitly but does not demonstrate how this data is relevant to the monadic formalism, while Grandis and Tholen do the latter without the former.

Help!!

Answer 1

My answer mostly consists of the relevant details of Omar Antolín-Camarena's answer to this MO question.

First of all, all the splittings you mention arise as solutions to canonical lifting problems of arrows against their $(\mathcal L,\mathcal R)$-factorizations. Such solutions are fillers for a commutative square with one edge the identity, and this is what gives the splitting.

A crucial observation is that the existence of such sections for a given arrow is actually equivalent to solving all lifting problems it involves. This equivalence is described in detail in section 12.4 of Emily Riehl's book Categorical Homotopy Theory. She also describes exactly how splittings are necessary for constructing lifts - they solve a generic lifting problem encompassing all the other ones for a given arrow.

Naturality of the splittings is the additional structure which brings in the density comonad. Recall naturality into $\mathsf{C}$ is the same as functoriality into $\mathsf{C^2}$, and it is the filler functor (called $\phi$ in the linked answer) that solves a lifting problem for the counit of the density comonad. So naturality lets us involve density comonads and motivates the monad-heavy definitions that come later. I think such functoriality is reasonable since in the context of cofibrant generation on a set $J$ seen as a discrete subcategory, we mightaswell look at it as the full subcategory of $\mathsf{C^2}$ generated by these arrows, and ask for coherence w.r.t composition in $\mathsf{C^2}$. The formalism then generalizes to general categories over $\mathsf{C^2}$.

Finally, about the specific issue of existence of splittings for $\rho_{Lf},\lambda_{Rf}$ as opposed to $\rho_f,\lambda_g$: these ensure different things. The former splittings must exist because they are equivalent via the generic lifting problem to $\lambda_f\in \mathcal L$ and $\rho_f\in \mathcal R$, which we obviously want to hold. So I think the authors of the paper mean they must exist because they assume $\lambda,\rho$ are indeed $(\mathcal L,\mathcal R)$-factorizations. The existence of the latter splittings is, again, equivalent to generic lifting problems, and decides whether $f\pitchfork g$ or not.