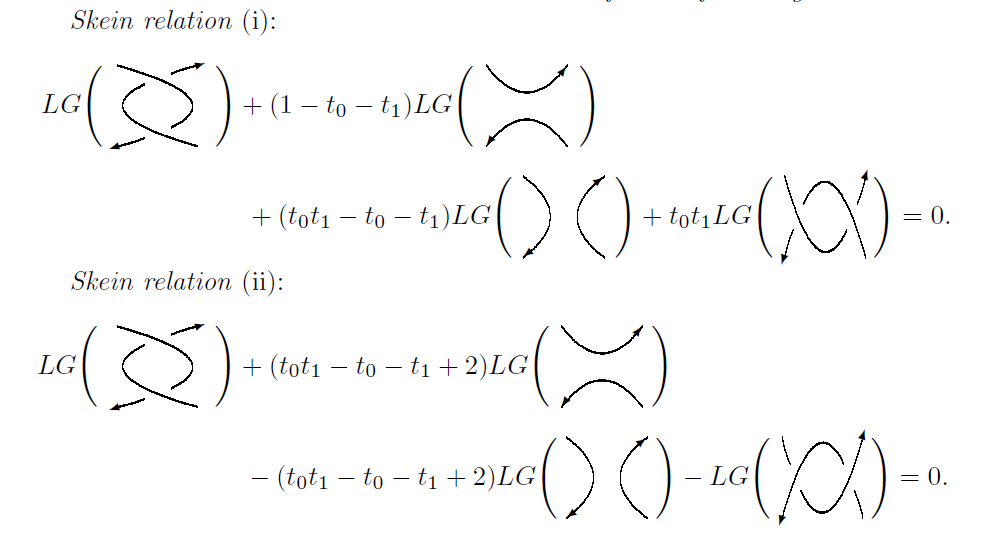Knot invariants with skein relation of order 3 or 4

Question

Many of the well-known knot/link invariants $I$, such as the Jones, Alexander, HOMFLY, Conway polynomials, satisfy a quadratic skein relation, i.e. an equation of the form $\alpha I(L_+)+\beta I(L_-)+\gamma I(L_0)=0$, where $L_\pm,L_0$ denote the same link up to a change of positive/negative or resolved crossing at a fixed crossing, and $\alpha,\beta,\gamma$ are coefficients related to the arguments of these polynomials.

I am wondering about link invariants which satisfy skein relations of "higher order", by which I mean an equation of the above form but with additional terms such as $I(L_{++})$, corresponding to two consecutive positve crossings, or similar.

In an $R$-matrix formulation, the quadratic skein relations correspond to a quadratic minimal polynomial, $\alpha R^2+\beta+\gamma R=0$. In this picture, my question is about link invariants corresponding to minimal polynomials of the form $\sum_{k=0}^N \alpha_k\,R^k=0$, with $N=3$ or $N=4$.

Specifically, are there any known invariants satisfying such "higher" skein relations?

Answer 1

For partial results in this direction, look at

Imre Tuba and Hans Wenzl, Representations of the braid group $B_3$ and of ${\rm SL}(2,{\bf Z})$, Pacific J. Math. 197 (2001), no. 2, 491--510.

which describes all the representations of $B_3$ with dimension at most five. (A knot invariant will give representations of all the braid groups, but whatever you get has to be consistent with the limited possibilities just for $B_3$.)

and

Scott Morrison, Emily Peters, and Noah Snyder, Categories generated by a trivalent vertex, to appear Selecta Mathematica.

in which we describe all link invariants arising from an object $X$ in a braided tensor category, in which $X \otimes X \cong 1 \oplus X \oplus A \oplus B$ for some objects $A$ and $B$ (so in particular the braiding on $X$ satisfies a quartic), and the braiding for $X$ can be written in terms of the map $X \otimes X \to X$.

Answer 2

As you mentioned, the Skein relation can be understood as R-matrix in the fundamental representation has two eigenvalues: in some normalization $$(R-q)(R+1/q)=0~.$$ In colored cases, the number of eigenvalues increases and one has $$\prod_i (R-\lambda_i(q))=0~,$$ which is no longer quadratic equation and is less convenient to use. For instance, in a certain basis, we have the relation $$(R-q^6)(R+q^2)(R-1)=0~,$$ for the second symmetric tensor product of the fundamental representation.

Eqn. (2.9) and (2.11) of this paper explicitly writes the Skein relations in colored cases. (See also sec 2 of this paper.)

Answer 3

The Links-Gould invariant, a 2-variable polynomial invariant coming from a one-parameter family of representations of the quantum superalgebra $\mathcal{U}_q(\mathfrak{gl}(2|1))$, satisfies the following skein relations:

The first was discovered by de-Wit, Kauffman, and Links:

De Wit, D., Links, J. R., & Kauffman, L. H. (1999). On the Links–Gould invariant of links. *Journal of Knot Theory and its Ramifications*, **8**(02), 165-199.

The second was discovered by Ishii (from where the image is taken):

Ishii, A. (2004). Algebraic links and skein relations of the Links-Gould invariant. *Proceedings of the American Mathematical Society*, **132**(12), 3741-3749.

Answer 4

The following two papers discuss the link invariants which satisfy "higher order" skein relations. Similar to the Jones polynomial, here only one crossing point is resolved, but all possible smoothing cases are considered.

New link invariants and Polynomials (I), oriented case

New link invariants and Polynomials (II), unoriented case