I'm reading the book "Elements of the representation theory of associative algebras, volume 1". And I can't understand the proof of the proposition 3.11 on page 124 (the place where marked green).
"because $P$ is injective, $u$ factors through $P$". How to get this result?
(There is a misprint in the statement of the proposition. It should say $R=\operatorname{rad} P$.)
You cannot get the result in green because it's false (in general). For example if the algebra is $$A=\begin{bmatrix} \mathbb C & \mathbb C & \mathbb C\\ 0 & \mathbb C & \mathbb C\\ 0 & 0 & \mathbb C \end{bmatrix}$$ and $P$ is the unique (up to isomorphism) indecomposable projective-injective module, then the monomorphism $u=\begin{bmatrix} q \\ i \end{bmatrix} \colon R \rightarrow R/S \oplus P$ is a counterexample. In this example there are no non-zero morphisms form $P$ to $R/S$, so $u$ cannot factor through $P$.
The proof of the proposition can be saved by assuming $U$ is indecomposable. The authors want to prove $\begin{bmatrix} q \\ i \end{bmatrix}$ is left almost split. In other words, whenever $u \colon R \to U$ is not a section, one needs to show that $u$ factors through $\begin{bmatrix} q \\ i \end{bmatrix}$. As pointed out above, it suffices to consider the case when $U$ is indecomposable.
If $u \colon R \to U$ is a monomorphism, then, since $P$ is injective, there is a morphism $h \colon U \to P$ such that $i=hu$.
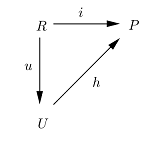
But by Proposition 3.5, since $P$ is indecomposable projective, $i \colon R \to P$ is an irreducible morphism, meaning that in the factorization $i=hu$ either $u$ is a section or $h$ is a retraction. It is assumed $u$ is not a section, so $h$ must be a retraction. Since $U$ is indecomposable, it follows that $h$ is an isomorphism. So $u=h^{-1}i$ and therefore $u$ factors through $P$.
