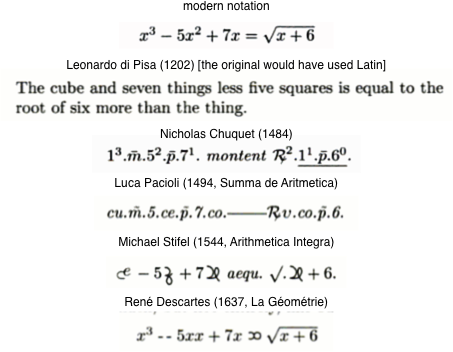29
$\begingroup$

I would like to see a simple example which shows how mathematical notation were evolve in time and space.

Say, consider the formula $$(x+2)^2=x^2+4{\cdot}x+4.$$ If I understand correctly, Franciscus Vieta would write something like this. (Feel free to correct me.)

$\overline{N+2}$ quadr. æqualia $Q+N\,4+4$.

($N$ stays for unknown and $Q$ for its square.)

Can you give me the other examples?

Improve this question
$\endgroup$
6
  • 3
    $\begingroup$ perhaps you might want to focus the question, in particular, to what extent is it not answered here: en.wikipedia.org/wiki/History_of_mathematical_notation $\endgroup$
    – Carlo Beenakker
    Commented Nov 28, 2015 at 12:24
  • 1
    $\begingroup$ @CarloBeenakker No, Wikipedia article does not contain the answer. And example like that would be helpful for me and others. I hope that there are people here on MO who could answer. $\endgroup$
    – Anton Petrunin
    Commented Nov 28, 2015 at 12:37
  • $\begingroup$ Too broad as MO question. I think there is a consensus about the history of the use of letters in mathematics. Apart from that, each single notation has its own history. Which of these have you in mind? $\endgroup$
    – Amir Asghari
    Commented Nov 28, 2015 at 13:05
  • 1
    $\begingroup$ Maybe Joseph Mazur's book Enlightening Symbols has an example of what you want? press.princeton.edu/titles/10204.html $\endgroup$
    – Timothy Chow
    Commented Nov 28, 2015 at 15:19
  • 4
    $\begingroup$ This question may also be a good fit for History of Science and Mathematics. $\endgroup$
    – Danu
    Commented Nov 29, 2015 at 16:22

5 Answers 5

Reset to default
30
$\begingroup$

For a quite extensive overview with many examples, you might want to check out The origins and development of mathematical notation. I also enjoyed reading Stephen Wolfram's take on Mathematical Notation – past and future, with a great variety of illustrations.

The OP asks specifically for the evolution of one formula, "to see the big picture". Here is one example, taken from Math through the ages:

Improve this answer
$\endgroup$
18
$\begingroup$

I think that the most complete source is still :

We can easily find there examples regarding the evolution of "algebraic" symbolism through the modern era :

Improve this answer
$\endgroup$
6
$\begingroup$

"The big picture" that can be seen in Carlo Beenakker's example is

Rhetorical (verbal); Syncopated (abbreviated words); Symbolic.

However, this well-known picture is very algebra-oriented and does not say anything about the use of notations in say geometry as discussed here " The Shaping of Deduction in Greek Mathematics: A Study in Cognitive History " or as asked here Uppercase Point Labels in High-School Diagrams. Thus, at least for me, the interesting answers to OP questions would be those that are not related to algebra.

Improve this answer
$\endgroup$
4
  • 3
    $\begingroup$ As an instance of the picture, the letter "e" in "et" for addition was wasted while "t" evolved to the symbol "+". $\endgroup$
    – Pietro Majer
    Commented Nov 29, 2015 at 8:21
  • $\begingroup$ @PietroMajer what a beautiful bit of history :) $\endgroup$
    – Amir Asghari
    Commented Nov 29, 2015 at 9:10
  • 2
    $\begingroup$ @PietroMajer, do you have evidence for this? Algebra was developed in Italian, where the word is just "e" and the "t" from Latin had been wasted. $\endgroup$
    – Paul Taylor
    Commented Dec 5, 2015 at 8:39
  • 3
    $\begingroup$ According to Florian Cajori, the mathematical symbol + came into use in Germany, between 1450-1480. He mentions a number of manuscripts in Latin from the Dresden Library were both "+" and "et" are used somehow interchangeably both in arithmetic and grammatical use. On the grammatical side, short forms are well-known in paleography. J.L.Walthers listes 102 different forms for the conjunction "et", including "+". $\endgroup$
    – Pietro Majer
    Commented Dec 5, 2015 at 9:51
3
$\begingroup$

You might also enjoy Earliest Uses of Symbols of Operation!!

Improve this answer
$\endgroup$
3
$\begingroup$

Find a quantity such that $\varsigma\;\overline{\beta}$ squared equals $$\Delta^{\Upsilon}\;\varsigma\overline{\delta}\;{\stackrel{o}{M}}\overline{\delta}$$

(My translation into English from Diophantes $\alpha\rho\iota\theta\mu\eta\tau\iota\kappa\eta$.)

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .