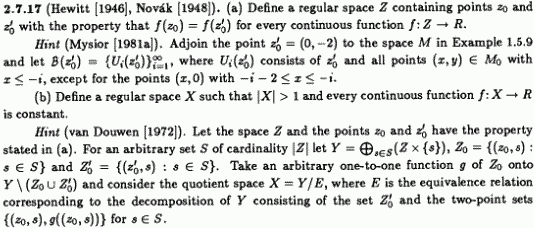
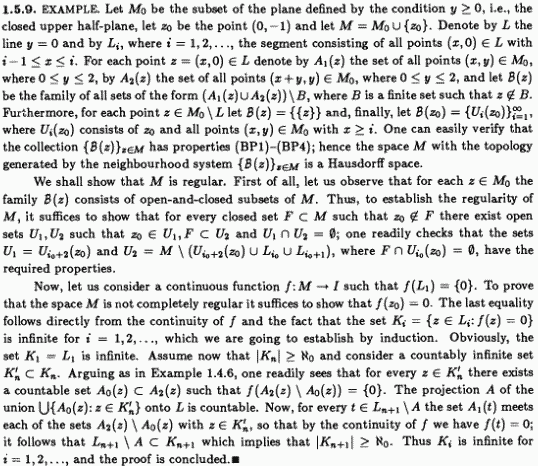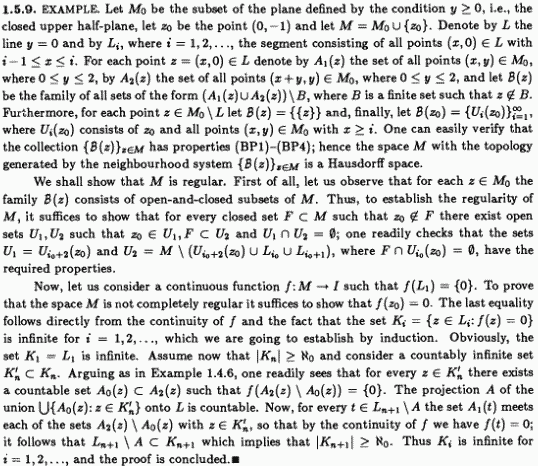Clearly, there are examples for the second question. Each Hausdorff abelian paratopological group (that is, a group endowed with a topology making the multiplication continuous) which is not a topological group (for instance, the Sorgenfrey arrow, that is the real line endowed with the Sorgenfrey topology generated by the base consisting of half-intervals $[a,b)$, $a<b$) is a counterexample. Less trivial and locally compact counterexample (which necessarily is not a group, because each locally compact paratopological group is a topological group) should be the additive semigroup of non-negative real numbers, endowed with the standard topology and then with isolated zero. (Maybe I even wrote a proof somewhere).
Update. A counterexample to your first question. For our purpose it is important that each Hausdorff space which admits a continuous injective map into a Hausdorff (para)topological group is functionally Hausdorff, because each Hausdorff topological group is Tychonoff (and each Hausdorff paratopological group is functionally Hausdorff). I recall that a space is functionally Hausdorff if for each distinct points $x,y\in X$ there exists a continuous function $f:X\to[0;1]$ such that $f(x)=0$ and $f(y)=1$. Clearly, each functionally Hausdorff space is Hausdorff. Let $X$ be an arbitrary Hausdorff space which is not functionally Hausdorff (see, for instance, quotations from “General topology” by Ryszard Engelking (Heldermann Verlag, Berlin, 1989) added at the end of the answer). Let $S(X)$ be a free abelian semigroup over a the space $X$, that is $S(X)$ is a set of formal sums $x=x_1+\cdots+x_n$ of elements of the set $X$. Clearly, $S(X)$ is a cancellative abelian semigroup. Determine a topology on the set $S(X)$ by its base consisting of all sets of the form $U_1+\cdots+U_n$, where $U_i$ are arbitrary open subsets of the space $X$ and $U_1+\cdots+U_n$ is a set of formal sums $x=x_1+\cdots+x_n$, where $x_i\in U_i$ for each $i$. Clearly, $S(X)$ is a topological semigroup. Since the space $X$ is naturally homeomorphic to a subspace of $S(X)$, the space $X$ is not functionally Hausdorff. It rests to show that the space $S(X)$ is Hausdorff. Let $x=x_1+\cdots+x_n$ and $y=y_1+\cdots+y_m$ be arbitrary distinct points of the set $S(X)$. Let $\{z_1,\dots, z_k\}=\{x_1,\dots, z_n\}\cup\{y_1,\dots, y_m\}$. Since the space $X$ is Hausdorff, there exist mutually disjoint neighborhoods $U(z_i)\ni z_i$. Put $U(x)=U(x_1)+\cdots+U(x_n)$ and $U(y)=U(y_1)+\cdots+U(y_m)$. Define a (not necessarily continuous) homomorphism $h:S(X)\to S^0(X)$ (where $S^0(X)=S(X)\cup \{0\}$ is the semigroup $S(X)$ with an added zero element $0$ such that $0+x=x+0=x$ for each element $x\in S(X)$) on the set $X$ of generators of the semigroup $S(X)$ by putting $h(z)=z_i$ if $z\in U(z_i)$ for some $i$ and $h(z)=0$, otherwise. Since the map $h$ is homomorhism, $h(U(x))=\{x\}$ and $h(U(y))=\{y\}$, so the neighborhoods $U(x)$ and $U(y)$ are disjoint.