33
$\begingroup$

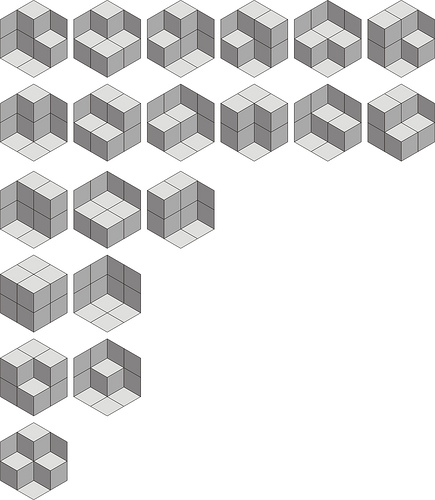
The McMahon formula for the number of tilings of an $a \times b \times c$ hexagon by lozenges:

$$ \Big[H(a)H(b)H(c)\Big] \Big[H(a+b)H(b+c)H(c+a)\Big]^{-1} \Big[H(a+b+c)\Big]$$

looks oddly like the inclusion-exclusion formula:

$$ |A \cup B \cup C| = |A|+|B|+|C| - |A \cap B| - |B \cap C| - |C \cap A| + |A \cap B \cap C|$$

Here $H(a) = 1! 2! \dots a!$ is the hyperfactorial.

Perhaps there is a more general explanation via Gelfand-Tsetlins or something?


(source: microsoft.com)

Improve this question
$\endgroup$
5
  • 10
    $\begingroup$ This is some shameless self-promotion, but it might be related to the observations in my preprint: arxiv.org/abs/1505.02717 where it turns out a lot of tableau-counting can be done with inclusion/exclusion. $\endgroup$
    – Per Alexandersson
    Commented Sep 23, 2015 at 18:38
  • $\begingroup$ There is a very nice post on Gel'fand-Tsetlin patterns by Terry Tao here: terrytao.wordpress.com/tag/gelfand-tsetlin-patterns $\endgroup$
    – Todd Trimble
    Commented Oct 4, 2015 at 12:56
  • 2
    $\begingroup$ The number of tilings is clearly symmetric in $a,b,c$, and also clearly vanishes when one of $a,b,c$ is zero. So if you already somehow know that the answer is going to be a product of functions of $a,b,c,a+b,a+c,b+c,a+b+c$, it has to basically be of the above form (but with an unknown function $H$). $\endgroup$
    – Terry Tao
    Commented Sep 29, 2017 at 1:19
  • $\begingroup$ There is a ``heuristic'' explanation of the hook-length formula counting Standard Young Tableaux (attributed to Knuth), where you ask for a random filling of the diagram what is the probability for each box that its entry is filled with the smallest value among all entries in its hook: this is one over the hook length; then, the events are not independent, but if you multiply all these probabilities together it turns out you get the right probability that they all are the smallest (namely, that the tableau is standard). Maybe some similar heuristic can explain the MacMahon (not "Mc") formula. $\endgroup$
    – Sam Hopkins
    Commented Sep 29, 2017 at 1:37
  • $\begingroup$ Just FYI I think your indices are off by 1 and you need to define the hyperfactorial as $H(a) = 1!\cdots (a-1)!$ for this to work (you can check the $c=1$ case and the $a=b=c=2$ example below) $\endgroup$
    – Roger Van Peski
    Commented Jan 24, 2019 at 1:45

1 Answer 1

Reset to default
2
$\begingroup$

This is not an answer, but seeing as this is a fresh post, I would like to add:

lozenges22

These are the $20\; 2\times2\times2$ plane partitions. Note there are $4$ of each color when viewed as hexagons.

If we let $a=1$, we get:

$$\dbinom{b+c}{c}=\prod_{i=1}^b\prod_{j=1}^c \dfrac{i+j}{i+j-1}=\prod_{i=1}^c \dfrac{i+b}{i}$$

A few more links:

Note the I-E principle is divisive and not subtractive.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .