Here are three facts which sound potentially related. What are the actual relationships?
In 1900, Dedekind constructed the free modular lattice on 3 generators as a sublattice of the lattice of subspaces of an 8-dimensional vector space. If the basis of the vector space is $e_1, \dots, e_8$, he looked at the subspaces $$ X = \langle e_2, e_4, e_5, e_8 \rangle , \; Y = \langle e_2, e_3, e_6, e_7 \rangle, \; Z = \langle e_1, e_4, e_6, e_7 + e_8 \rangle $$ By repeatedly taking intersections and unions, you can build up 28 subspaces starting from these three. This proves the free modular lattice on 3 generators has at least 28 elements. In fact it has exactly 28 elements. I think Dedekind showed this by working out the free modular lattice 'by hand' and noting that it, too, has 28 elements.
The dimension of $\mathrm{SO}(8)$ is 28. Its Lie algebra $\mathfrak{so}(8)$, also called $D_4$, has 12 positive roots, and its Cartan algebra has dimension 4. As usual, the Lie algebra is spanned by positive roots, an equal number of negative roots, and the Cartan subalgebra, so we get $$ 28 = 12 + 12 + 4 $$
The 3 subspace problem asks us to classify triples of subspaces of a finite-dimensional vector space $V$, up to invertible linear transformations of $V$. There are finitely many possibilities, unlike the situation for the 4 subspace problem. One way to see this is to note that 3 subspaces $X, Y, Z \subseteq V$ give a representation of the $D_4$ quiver. This is nothing profound: a representation of the $D_4$ quiver is just 3 linear maps $X \to V$, $Y \to V$, $Z \to V$, and here we are taking those to be inclusions. The nontrivial part is that indecomposable representations of any Dynkin quiver correspond in a natural one-to-one way with positive roots of the corresponding Lie algebra. So, in particular, the $D_4$ quiver has 12 indecomposable representations. The representation coming from $X, Y, Z \subseteq V$ must be a direct sum of indecomposable representations, so we can classify the possibilities and solve the 3 subspace problem.
Here is a way of making my question more concrete. However, it may be on the wrong track:
Is there a natural 1-1 correspondence between the 28 elements of the free modular lattice on 3 generators and some basis of $\mathfrak{so}(8)$?
Here is another more cautious way:
What is known about the relation between the free modular lattice on 3 generators and the 3 subspace problem?
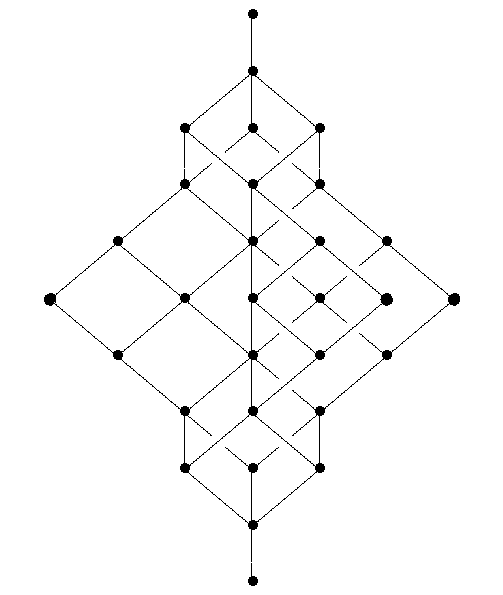
It would help if one could somehow directly relate the free modular lattice on 3 generators to something built from $D_4$. The free modular lattice on 3 generators looks like this:
For more background information, see:
- John Baez, The free modular lattice on 3 generators, The n-Category Café, September 19, 2015.