The Schwede-Shipley theorem gives a criterion for a presentable stable $\infty$-category to be the category of modules over an $\mathcal{E}_1$-algebra. Is there any similar criterion for a monoidal presentable stable $\infty$-category to be the category of modules over an $\mathcal{E}_2$-algebra?
4
-
8$\begingroup$ True iff the unit for the monoidal structure is a compact generator. $\endgroup$– Jacob LurieCommented Aug 15, 2015 at 22:39
-
1$\begingroup$ What theorem? They have more than one. $\endgroup$– Fernando MuroCommented Aug 15, 2015 at 22:51
-
2$\begingroup$ @FernandoMuro: I think based on context the OP must mean their paper "Stable Model Categories are Categories of Modules" $\endgroup$– David WhiteCommented Aug 16, 2015 at 12:42
-
$\begingroup$ @DavidWhite thanks! So maybe abc.xyz could restate the question saying that he's considering the category of modules over an $E_1$(resp. $E_2$)-algebra in the stable $\infty$-category of spectra. $\endgroup$– Fernando MuroCommented Aug 16, 2015 at 14:50
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
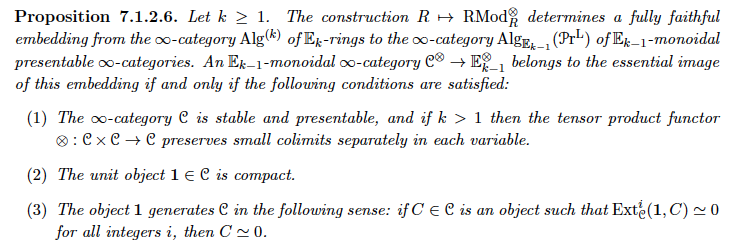
This is an old question, but just for completeness, the answer to this question is that yes there is a similar criterion (as Jacob Lurie comments above). This is Proposition 7.1.2.6 of Jacob's Higher Algebra. I'll post a screenshot here instead of transcribing the entire thing, as it's sort of long.
answered Sep 1, 2023 at 16:12