Suppose $G \subseteq \mathbb{R}^2$ is dense $G_\delta$. Must there (in ZFC) exist non meager sets of reals $A, B$ such that $A \times B \subseteq G$?
$\begingroup$
$\endgroup$
2
-
3$\begingroup$ Note that the measure analogue is false: H. Friedman and independently Shelah showed that in the Cohen real model there is a co-null set which does not contain a non null rectangle. See J. Pawlikowski: On a rectangle inclusion problem, Proc. Amer. Math. Soc. 123 No. 10 (1995), 3189-95 $\endgroup$– AshutoshCommented Jun 14, 2015 at 20:32
-
$\begingroup$ A related question is: (M. Burke, Note of 3-7-1991) Is it consistent that every null additive subgroup of reals is meager? $\endgroup$– AshutoshCommented Jun 14, 2015 at 21:09
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
I pointed this question to Taras Banakh, who pointed to me his joint paper with Lyubomyr Zdomskyy “Non-meager free sets for meager relations on Polish spaces” which contains an answer.
Abstract. We prove that for each meager relation $E\subset X\times X$ on a Polish space $X$ there is a nowhere meager subspace $F\subset X$ which is $E$-free in the sense that $(x,y)\not\in E$ for any distinct points $x,y\in F$.
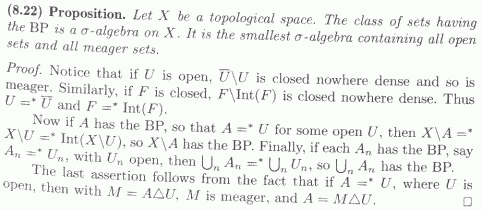
From myself I add a simple negative answer for descriptively good $A$ and $B$, that is when both $A$ and $B$ have the Baire Property. I recall, that a subset $B$ of a topological space $X$ has the Baire Property (BP) in $X$ if $B$ contains a $G_\delta$-subset $C$ of $X$ such that $B\setminus C$ is meager in $X$. By [Kech, 8.22] each Borel subset of a space $X$ has the Baire Property in $X$. Put $G=\mathbb{R}^2\setminus \{(x,y):x-y\in\Bbb Q\}.$ Assume that both $A$ and $B$ are non meager subsets of the space $\Bbb R$ with the Baire Property and $A\times B\subset G$. By [Kech, Prop. 8.22] both sets $A+\Bbb Q$ and $B+\Bbb Q$ have the Baire Property. By [Kech, Th. 8.46] both sets $A+\Bbb Q$ and $B+\Bbb Q$ are comeger. So $A+Q\cap B+Q\ne\varnothing$. That is, there exist points $a\in A$, $b\in B$, $q, q’\in\Bbb Q$ such that $a+q=b+q’$. Then $(a,b)\in A\times B\setminus G$, a contradiction.
References
[Kech] A. Kechris. Classical Descriptive Set Theory, – Springer, 1995.
answered Jun 15, 2015 at 19:22
-
2$\begingroup$ To see how the quoted result implies a solution to the given question (just because it took me a couple minutes): take $X = \mathbb{R}$ and $E = \mathbb{R}^2 \setminus G$, apply the quoted result to produce $F$; then $F \times F \subset G \cup D$, where $D$ is the diagonal. Now let $U,V$ be any two nonempty disjoint open subsets of $\mathbb{R}$; take $A = F \cap U$ and $B = F \cap V$. Since $F$ is nowhere meager, $A,B$ are nonmeager. We have $A \times B \subset F \times F \subset G \cup D$, and since $A,B$ are disjoint, we have $A \times B$ disjoint from $D$. So $A \times B \subset G$. $\endgroup$ Commented Jun 15, 2015 at 21:34