4
$\begingroup$

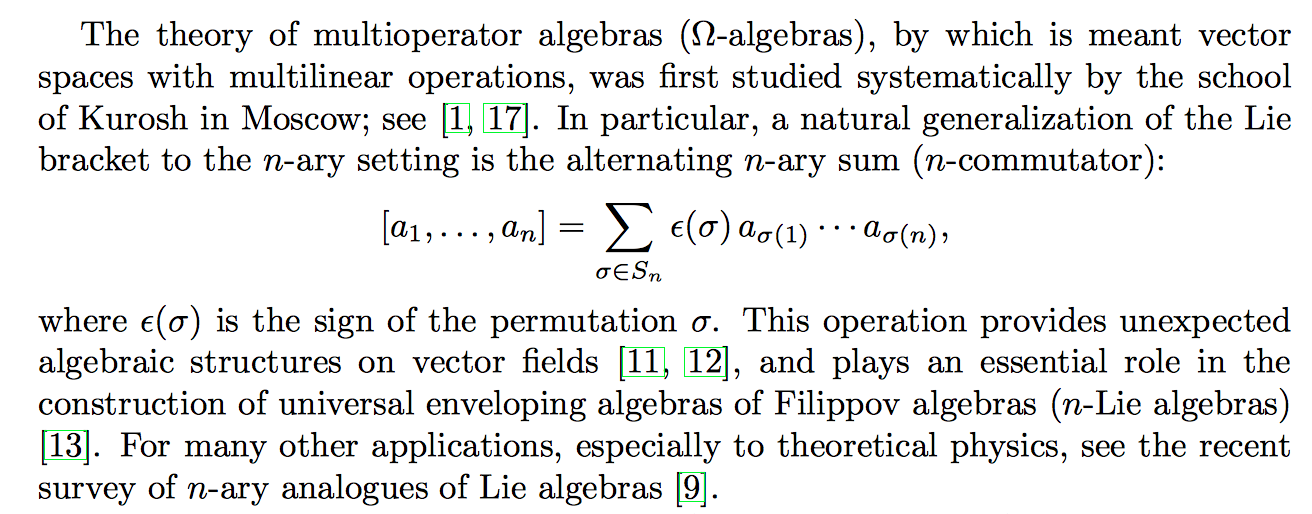
I was wondering if there's any attempt to generalize the commutator for something general for more than two terms.

Here's what I was thinking of, for $[A,B]=AB-BA$, so for three terms:

$[A,B,C] = ABC+BCA+CAB-BAC-CBA-ACB$

where I have taken a plus sign for a cyclic permutation of $ABC$, and a minus sign for acyclic permutation of $ABC$.

Is such a generalization still called commutator or something else?

Improve this question
$\endgroup$
3
  • $\begingroup$ A little remark: Your $[A,B,C]$ is invariant under cyclic permutations by definition and $[A,B,C]+[B,A,C]=0$ is the Jacobi identity. $\endgroup$
    – Joonas Ilmavirta
    Commented Jun 11, 2015 at 14:48
  • $\begingroup$ @arsmath I usually first ask and then edit only if the author doesn't react. Also I expected the author to add the setting (associative rings...) (For cleaning I'll erase this comment, please do the same with yours.) $\endgroup$
    – YCor
    Commented Jun 11, 2015 at 16:20
  • $\begingroup$ Of course, there is the "associator" $[x,y,z]:=(xy)z-x(yz)$, which is identically zero if and only if the ambient algebra is associative. $\endgroup$
    – Christopher Drupieski
    Commented Jun 11, 2015 at 16:59

2 Answers 2

Reset to default
7
$\begingroup$

This generalization is called a ternary commutator, and there are $n$-commutator generalizations, as explained here:

Improve this answer
$\endgroup$
3
$\begingroup$

It should be noted that commutator has been generalized to congruences for results in universal algebra. Look up reviews of "Commutator Theory For Congruence Modular Varieties" by Freese and McKenzie. Many far-reaching results are obtained by considering a commutator operation on congruence lattices.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .