10
$\begingroup$

I am wondering about the existence of incompressible surfaces in the Weeks manifold. Is this space a Haken manifold?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
10
$\begingroup$

No, it is not Haken.

You can test this yourself by looking for incompressible normal surfaces with respect to a triangulation of the manifold, this functionality is included in Regina. Moreover, the Weeks manifold is a premade example so determining if it is Haken can be done directly from within the Recognition tab. This completes in a few seconds on my laptop. You can read more about the normal surface theory algorithms used by Regina to do this in the documentation or in the original paper by Jaco and Oertel.

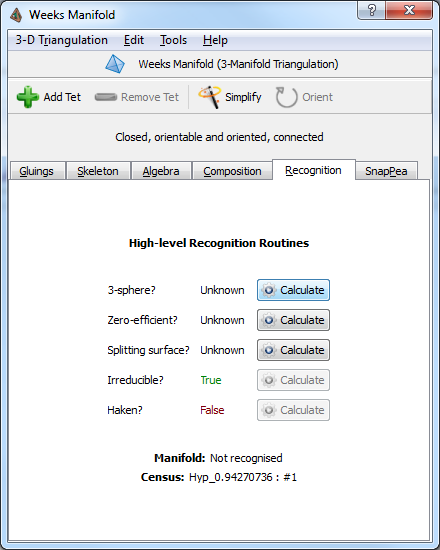
Testing within Regina 4.96

Improve this answer
$\endgroup$
2
  • $\begingroup$ I did not know this software. Thank you very much. $\endgroup$
    – Vanderson Lima
    Commented May 18, 2015 at 2:07
  • 3
    $\begingroup$ I should add that Regina is able to do this calculation for any triangulation of a closed or cusped manifold. The algorithm is at least exponential in the number of tetrahedra but is still practical even when this number becomes quite large. For example in 2009 Burton, Rubinstein and Tillmann used it to show that the Seifert-Weber dodecahedral space is not Haken using a triangulation with 23 tetrahedra. See arxiv.org/abs/0909.4625 $\endgroup$
    – Mark Bell
    Commented May 18, 2015 at 7:40
2
$\begingroup$

No, it is not. see, for example this reference: https://books.google.com/books?id=3s4bCAAAQBAJ&pg=PA101&lpg=PA101&dq=Weeks+manifold+haken&source=bl&ots=jCQ_jlddLA&sig=QMTywVy5BPwqsLnCPduk0XSzRuA&hl=en&sa=X&ei=5rdYVdTuO8ifyASB94LwBg&ved=0CEkQ6AEwBg#v=onepage&q=Weeks%20manifold%20haken&f=false on MOM technology (in the tradition of Ahlfors-Bers, V)

Improve this answer
$\endgroup$
0

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .