I have a problem concerning a fact which is stated without proof in this Rezk's draft: http://www.math.uiuc.edu/~rezk/i-hate-the-pi-star-kan-condition.pdf . In the proof of Lemma 2.11, we are given a fibration $p:E \to B$ and a diagram $$V: J \to Psh(I)/B$$ where $Psh(I)$ denotes simplicial presheaves on $I$, such that any arrow $V(j) \to B$ is such that the pullback of $p$ along it gives us a square (a homotopy cartesian one, of course) which remains homotopy cartesian after having applied the homotopy colimit functor $$hocolim:Psh(I)\to sSet$$ The claim is that the map $$hocolim(V)\to B$$ should then have the same property. What I have done so far is firstly having interpreted this claim as: pick a cofibrant replacement $\mathcal{Q}V\simeq V$ in $Psh(I)^J$ and consider the map $colim \mathcal{Q}V \to B$, we want it to have the abovementioned property (notice that the maps $\mathcal{Q}V(j) \to B$ have it, since they are weakly equivalent to the $V(j) \to B$ which have it by assumption).
I would like to use the following result, but I haven't find a way in which it could turn out to solve my problem:
Here, $E \to B$ is equifibered if the following square is homotopy cartesian for any choice of $J_1,J_2,\alpha$: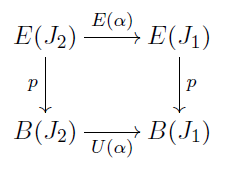
And $E \to B$ is a realization-fibration if any homotopy cartesian square of the form 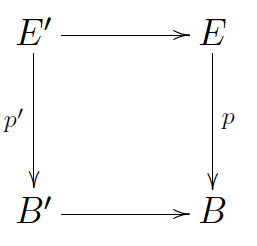 remains homotopy cartesian after having applied the homotopy colimit functor
remains homotopy cartesian after having applied the homotopy colimit functor
Thanks in advance for any hint or advice.
