This is the standard definition for a tensor in a smooth manifold (Nakahara, for example):
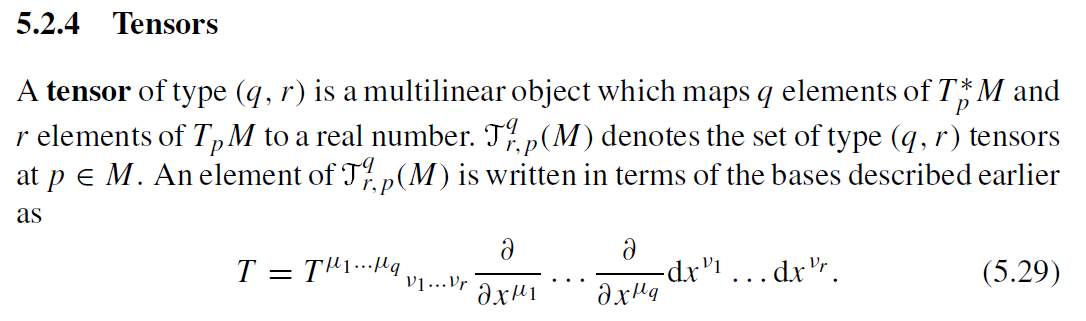
But while reading Salamon's Riemannian Geometry and Holonomy Groups I have found this rather different definition (page 11):
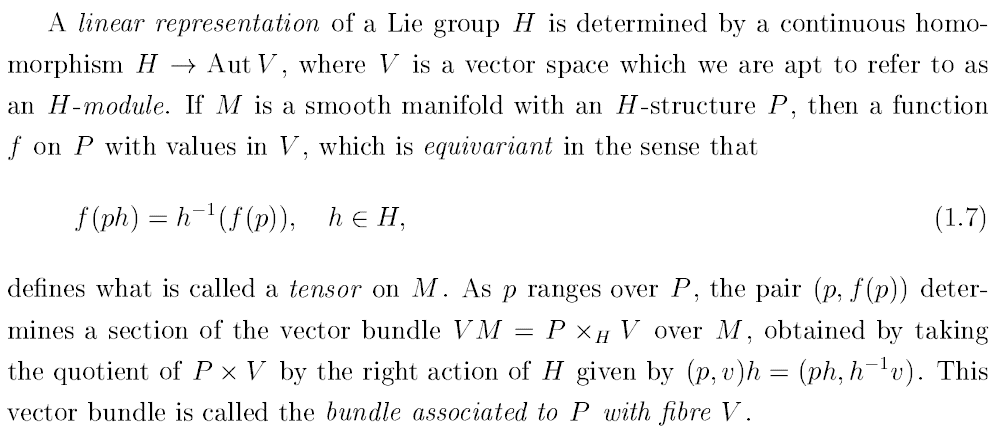
Is this second definition similar to the first one? Is it a generalisation? I would find it very useful if somebody could help me improving my skills in this new setting.
The second definition is a generalization of the first. It is not equivalent. To see the first definition arising from the second, take $P$ to be the frame bundle so $H=GL(V)$ with $V=\mathbb{R}^n$ Then take as representations the tensor product $V^{\otimes p} \otimes V^{* \otimes q}$.
For an example where the two definitions are very different, take $H$ to be a compact and simply connected subgroup of $SO(n)$. For example, take $H=G_2 \subset SO(7)$. Then $H$ has a spin representation, because there is a lift $H \subset Spin(n)$. But $GL(7,\mathbb{R})$ and $SO(7)$ don't have spin representations. Therefore $H$-manifolds have spinor fields, which Salamon is defining to be tensors, but no physicist would like that sort of definition.
