In this paper, Guilherme França and André LeClair show that $$\gamma_{y}\sim 2 \pi \left(y-11/8\right)/W\left((y-11/8)e^{-1}\right)$$ where $W$ is the Lambert W function, and $\gamma_{y}$ is the imaginary part of the the $y$th non-trivial zero along the critical line. This formula can then be used in a similar way to Gram points for finding zeta zeros.
When this function replaces $y$ in $\zeta(1/2+iy)$, and the real and imaginary parts are plotted parametrically, the result shares superficial similarities with a limaçon:
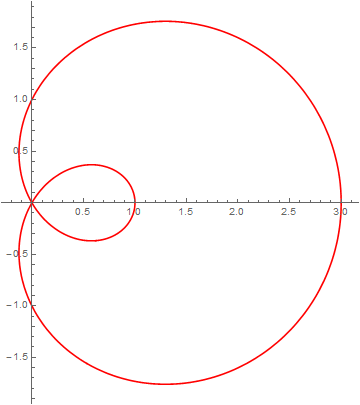
where one complete orbit is made approximately in every integer interval.
Firstly, analysing the limaçon in terms of its orbit seen as a particle travelling at constant velocity around the origin, comparing curvature, distance from origin, and non-directional "speed", where
\begin{align} &x(t)\text{:=}\cos (t)+\cos (2 t)+1&\\ &y(t)\text{:=}\sin (t)+\sin (2 t)&\\ \end{align}
the solutions to
\begin{align} t\in\mathbb{R}:\frac{2 \left(x'(t)^2+y'(t)^2\right)^{3/2}}{\left| x'(t) y''(t)-x''(t) y'(t)\right| }=\sqrt{x'(t)^2+y'(t)^2} \end{align}
are clearly
\begin{align} t=2\pi n-\cos^{-1}(-5/4),n\in\mathbb{Z}\\ t=2\pi n+\cos^{-1}(-5/4),n\in\mathbb{Z}\\ t=\dfrac{2}{3}(3\pi n-\pi),n\in\mathbb{Z}\\ t=\dfrac{2}{3}(3\pi n+\pi),n\in\mathbb{Z}\\ \end{align}
Interestingly, the zeta function has similar properties when analysed in this way. Since
\begin{align} |Z(x)|=\sqrt{\Im\left(\zeta \left(\frac{1}{2}+i y\right)\right)^2+\Re\left(\zeta \left(\frac{1}{2}+i y\right)\right)^2} \end{align}
where $Z$ is the Riemann-Siegel Z function, $\sqrt{\Im(f(y))^2+\Re(f(y))^2}$ is zero at $\gamma=2 \pi \left(y-11/8\right)/W\left((y-11/8)e^{-1}\right),$ for
\begin{align} &f(\text{y})\text{:=}\zeta \left(2 i \pi \left(y-11/8\right)/W\left((y-11/8)e^{-1}\right)+1/2\right)&\\ &v(y)\text{:=}\sqrt{\Im\left(f'(y)\right)^2+\Re\left(f'(y)\right)^2}\\ &\kappa (y)\text{:=}\frac{\left| \Re\left(f'(y)\right) \Im\left(f''(y)\right)-\Im\left(f'(y)\right) \Re\left(f''(y)\right)\right| }{\left(\Im\left(f'(y)\right)^2+\Re\left(f'(y)\right)^2\right)^{3/2}}\\ &d(y)\text{:=}\sqrt{\Im(f(y))^2+\Re(f(y))^2} \end{align}
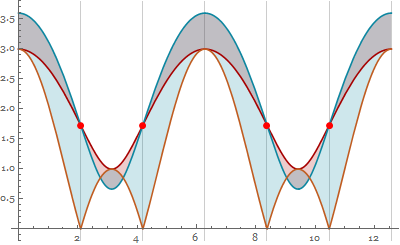
a plot of $\{v(y),2 \pi /\kappa (y),\pi d(y)\}$ with gridlines at $\gamma=2 \pi \left(y-11/8\right)/W\left((y-11/8)e^{-1}\right)$ looks like this:

where it can be seen that $\{v(y),2 \pi /\kappa (y)\}$ intersect at the zeta zeros.
f[y_] := InputForm[Zeta[Rational[1, 2] + Complex[0, 2] Pi (Rational[-11, 8]
+ y)/ProductLog[E^(-1) (Rational[-11, 8] + y)]]]
v[y_] := InputForm[(Im[Derivative[1][f][y]]^2 + Re[Derivative[1][f][y]]^2)^
Rational[1, 2]]
\[Kappa][y_] := InputForm[Abs[Im[Derivative[2][f][y]] Re[Derivative[1][f][y]] -
Im[Derivative[1][f][y]] Re[Derivative[2][f][y]]] (Im[Derivative[1][f][y]]^2 + Re[
Derivative[1][f][y]]^2)^Rational[-3, 2]]
d[y_] := InputForm[(Im[f[y]]^2 + Re[f[y]]^2)^Rational[1, 2]]
r = 2; rr = 15;
zeros = Rest@ Table[y /. FindRoot[
2 Pi (-11/8 + y)/LambertW[(-11/8 + y)/E] == Im[ZetaZero[n]], {y,n}], {n, r, rr}];
Plot[{v[y], 2 \[Pi]/\[Kappa][y], \[Pi] d[y]}, {y, r, rr},
PlotRange -> All, PlotTheme -> "Garnet",
Filling -> {1 -> {2}, 2 -> {3}}, Axes -> False, Frame -> True,
ImageSize -> 400, GridLines -> {zeros, {}}, Epilog -> {Red, PointSize[Large],
Point[Transpose@{zeros, Table[v[y], {y, zeros}]}]
It can be seen then that there is clearly a close relationship between the curvature of $f(y)$ (ie $\kappa(y)$), the speed of a particle moving along the path of $f(y)$ (ie $v(y)$), and the distance from origin (ie $d(y)$).
My question then is how would I go about finding the solutions to
\begin{align} A=\left\{y\in\mathbb{R}:v(y)=\dfrac{2\pi}{\kappa(y)}\right\} \end{align}
analytically? And for
\begin{align} B=\left\{y\in\mathbb{R}:\gamma=2 \pi \left(y-11/8\right)/W\left((y-11/8)e^{-1}\right)\right\} \end{align}
is it true that $B\in A$ ?
Update
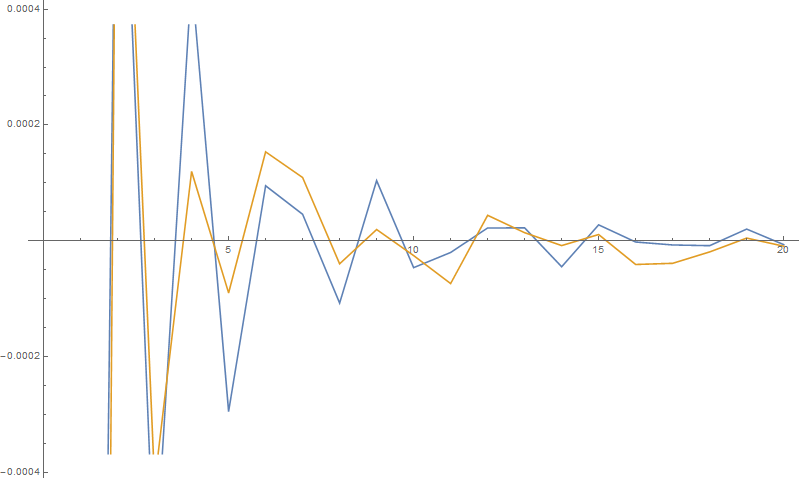
In response to მამუკა ჯიბლაძე's comment, it certainly seems from a quick numerical check that $v(y),2\pi/\kappa(y)$ don't actually intersect at the zeta zeros, but are related to their approximations:
yy = y /. Table[FindRoot[v[y] == 2 Pi/\[Kappa][y], {y, n}], {n, 1, 20}];
y1 = Table[w[yy[[y]]], {y, 1, Length@yy}] // N;
y2 = Table[Im@ZetaZero[y], {y, 1, Length@yy}] // N;
y3 = Table[w[y], {y, 1, Length@yy}] // N;
ListLinePlot[{Table[(Log[y2[[y]]] - Log[y3[[y]]])/(2 Pi y^(3/2)), {y, 1,
Length@yy}], y1 - y2}]
where for
\begin{align} &w(y):=2 \pi \left(y-11/8\right)/W\left((y-11/8)e^{-1}\right)\\ &r_{y}:=\text{solutions to }v(y)=2\pi/\kappa(y) \end{align}
the above is a plot of $(\log(\gamma_{y})-\log(w(y))/(2\pi y^{3/2})$ against $w(r_{y})-\gamma_{y},$ so it would seem that $B\notin A.$
However, I am still unsure how to show this analytically, and since $(\log(\gamma_{y})-\log(w(y))/(2\pi y^{3/2})$ is just a rough guess, I would be interested to know whether there is a more accurate definition of the relationship between $r_{y}$ and $\gamma_{y}$.
