Any no-zero homomorphism of holomorphic vector bundles over a compact Riemann surface factors through a maximal rank homomorphism

Question

I was reading the paper "Stable and Unitary vector bundles on a compact surface" by Narashiman and Seshadri.

I quote from the paper-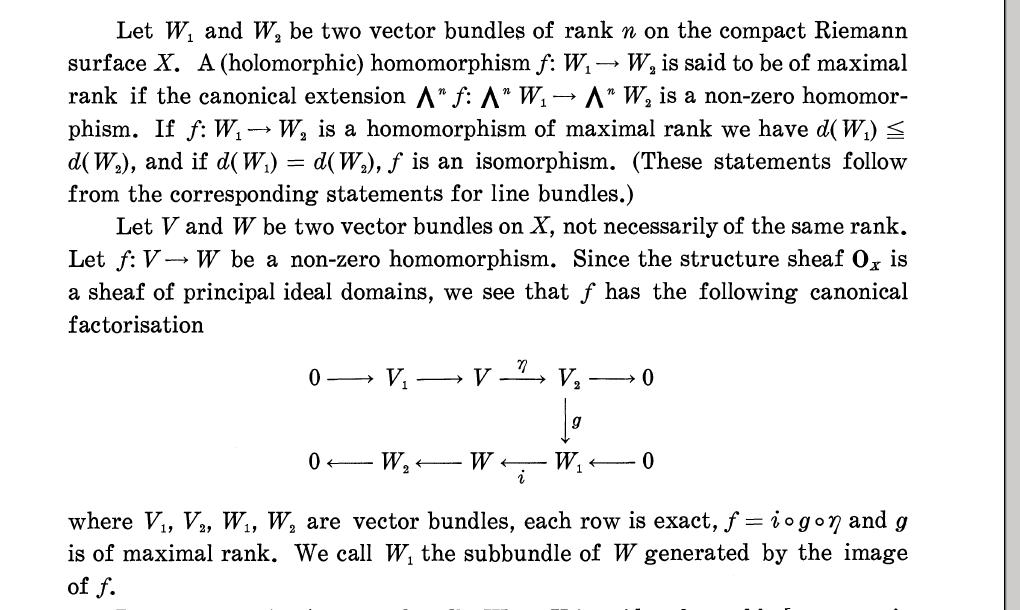

Can someone please explain how does any non-zero homomorphism of vector bundles can be factored through a maximal rank homomorphisms? It will be helpful if someone provides with an simple to read reference.

Answer 1

Let $V_2$ be the image of $f:V\rightarrow W$; it is a subsheaf of $W$, hence locally free. Let $W_2$ be the quotient of $W/V_2$ by its torsion subsheaf, and let $W_1$ be the kernel of the projection $W\rightarrow W_2$. The induced map $V_2\rightarrow W_1$ has maximal rank, and you get exactly the situation described in the paper.