1
$\begingroup$

The notion of formal proof is defined by finite sequences ($<\omega$ - sequences) of sentences. In some sense if a sentence $\sigma$ is (finitely) provable from the theory $T$ it is very "near" to the "home theory $T$". In this meaning a (finitely) consistent theory is an "origin" with no "near" inconsistency. Also one can think about possibility of "reaching" to an unprovable sentence by increasing the length of the proofs. Now let's replace the notion of first order logic ($L_{\omega,\omega}$) which uses finite proofs with some infinitary logic like $L_{\alpha,\beta}$ and look at the the theory $\text{ZFC}$ using this new glasses.

Definition 1: A theory $T$ in logic $L_{\alpha , \beta}$ is "totally consistent" if for all ordinals $\gamma\geq \alpha , \delta\geq \beta$ the theory $T$ is consistent in logic $L_{\gamma,\delta}$ too.

Question 1: Assuming consistency of $\text{ZFC}$ in first order logic, is the $L_{\omega , \omega}$ - theory $\text{ZFC}$ totally consistent? In the other words: is it possible to prove an inconsistency from $\text{ZFC}$ by allowing proofs in higher length in infinatry logics?

Question 2: Is any unprovable sentence from $\text{ZFC}$ somewhere decidable? Precisely: let $\sigma$ be an unprovable sentence from $\text{ZFC}$ in logic $L_{\omega ,\omega}$. Are there two large enough ordinals $\alpha , \beta$ such that in the logic $L_{\alpha,\beta}$ there is a proof from $\text{ZFC}$ for $\sigma$ or $\neg \sigma$?

Definition 2: Let $T$ be a first order theory and $\sigma$ a sentence. Define the "distance of $\sigma$ from $T$" as follows: $d(T,\sigma):=min\{\alpha\in \text{Ord}~|~\text{In the logic}~L_{\alpha,\alpha}~\text{there is a proof from}~T~\text{for}~\sigma\}$ and if $\{\alpha\in \text{Ord}~|~\text{In the logic}~L_{\alpha,\alpha}~\text{there is a proof from}~T~\text{for}~\sigma\}=\emptyset$ then $d(T,\sigma)=\infty$

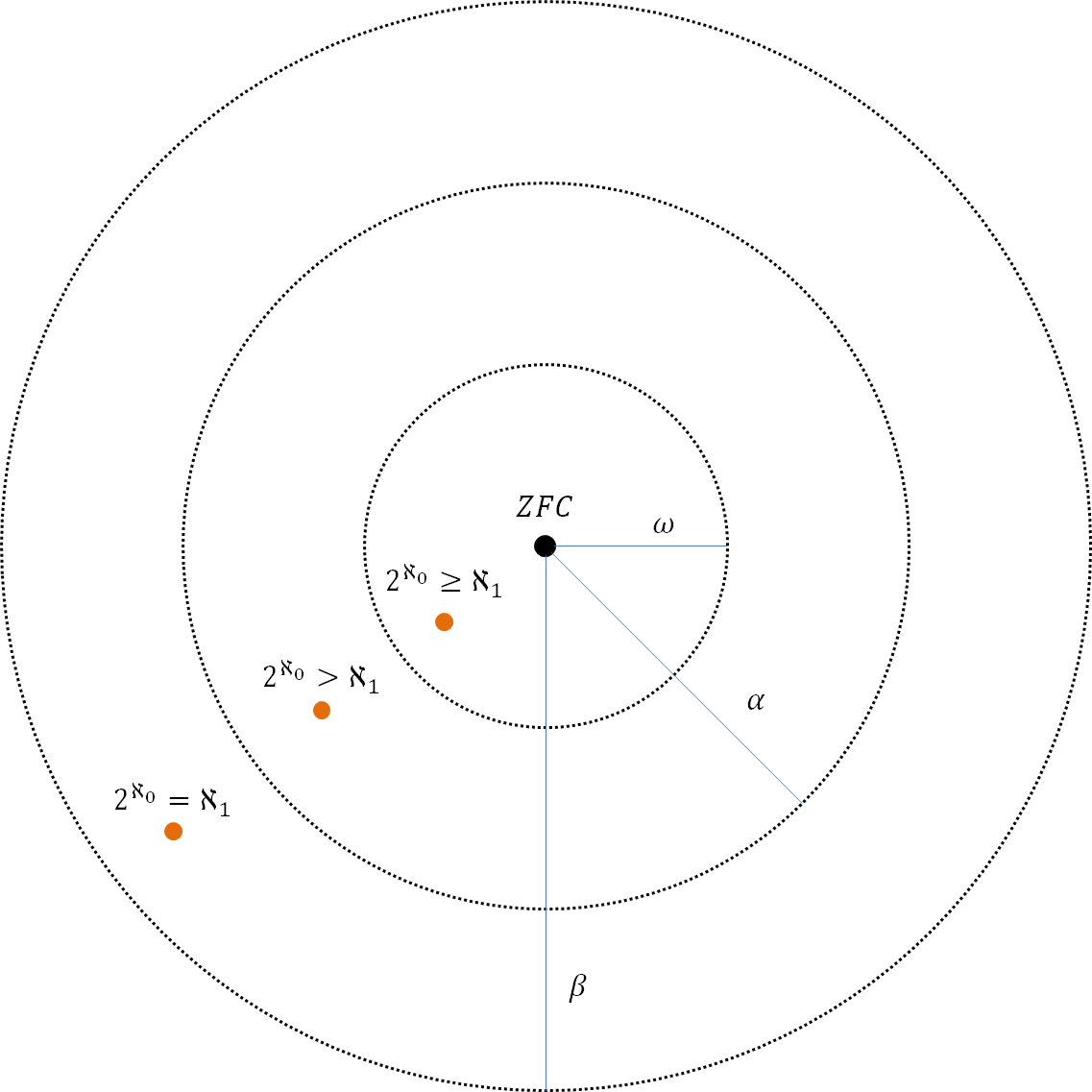
Remark 1: We have $d(\text{ZFC},2^{\aleph_{0}}\geq\aleph_{1})\leq \omega$.

Question 3: What are the values of $d(\text{ZFC},\text{CH})$ and $d(\text{ZFC},\neg\text{CH})$?

Remark 2: The following imaginary diagram shows that $d(\text{ZFC},\text{CT})\leq \omega \leq d(\text{ZFC},\neg\text{CH})\leq \alpha\leq d(\text{ZFC},\text{CH})\leq \beta$ which $\text{CT}$ is Cantor's Theorem. Also it shows that $\text{ZFC}$ as a first order theory is not totally consistent and one reachs to an inconsistency if he/she goes too far from it.

enter image description here

Improve this question
$\endgroup$
12
  • 1
    $\begingroup$ Why the downvotes? Even though there is an obvious gap in the question (see Simon Henry's answer) it is not unfixable and given that St Georg has obviously put some effort into making this a good MO question, we should give him the chance to close the gap and provide the missing information. $\endgroup$
    – Johannes Hahn
    Commented Nov 29, 2013 at 14:33
  • 5
    $\begingroup$ @Johannes: I didn't downvote but there are two reasons, in addition to the uninformative title, why this question is not well thought out. 1.- The Compactness Theorem trivializes the notions defined in the question. 2.- A Wikipedia search for "infinitary logic" or related terms would direct to the correct definitions. $\endgroup$
    – François G. Dorais
    Commented Nov 29, 2013 at 15:15
  • 2
    $\begingroup$ Already at Definition 1, there's a problem - what does "consistent" mean for infinitary logics? $\endgroup$
    – Noah Schweber
    Commented Nov 30, 2013 at 5:05
  • 6
    $\begingroup$ The question is flawed in the sense that a competent researcher in logic and set theory would be aware of the problems it contains. But I see completely trivial questions about constructive math asked all the time and nobody seems to mind. To my mind, there is a strong bias here. If I downvoted the trivialities asked about cosntructive math everyone would get on my case. So please the set-theory posse, show some mercy. $\endgroup$
    – Andrej Bauer
    Commented Nov 30, 2013 at 9:33
  • 1
    $\begingroup$ Andrej, that's a good point. I hope I didn't come off as aggressive above. @Georg: The notions of "proof" and "contradiction" for infinitary logics are a bit more subtle than you seem to be giving them credit for. In particular - at least, in all systems I know about - there are unsatisfiable $L_{\infty, \infty}$-sentences which do not "prove" a contradiction in any nice sense of the word "prove." The point is: you need to actually define what you mean by "proof," etc. $\endgroup$
    – Noah Schweber
    Commented Nov 30, 2013 at 16:04

1 Answer 1

Reset to default
7
$\begingroup$

If you don't allow in your theory (or your logic) axioms or rules that allow to use an infinite number of proposition to deduce a new one (which is not possible if you stick to "finitary logic" ) then any "infinite length proof" of arbitrary length can be turn in a finite length proof, indeed, when you reach the first limite ordinal $w$ the fact that the statement $A_w$ is deducible from the $a_i$ for $i$ finite immediately yields that it is deducible from a finite number of them and hence you could have proved it with a finite proof. An ordinal induction based on this argument immediately give the desired result:

At each step $\alpha$, the statement $A_\alpha$ is deducible from a finite number of $A_\beta$ for $\beta < \alpha$ which all admit a finite proof, hence $A_\alpha$ admit a finite proof.

Maybe if you allow infinite conjunction/disjunction in your proposition it may change something but you have to be more precise on the deduction you will use at each steps.

Improve this answer
$\endgroup$