12
$\begingroup$

Let $P$ be a convex $n$-gon. Suppose that we have an infinite number of $P$s, and that each of them is colored either red or blue. Here, let us consider the following operations :

Operation 1 : Place a red $P$ on a plane.

$\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ $enter image description here

Operation 2 : Place $n$ blue $P$s around the red $P$ such that each of the blue $P$s and the red $P$ are laid symmetrically with each $E_i\ (i=1,2,\cdots,n)$ where $E_i$ is an edge of the red $P$.

$\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ $enter image description here

Operation 3 : Place $n$ red $P$s around every blue $P$ in the same way as operation 2.

$\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ $enter image description here

Operation 4 : Place $n$ blue $P$s around every red $P$ in the same way as operation 2.

Operation 5 : Repeat operation 3 and 4.

Here, let us consider the following conditions :

Condition 1 : These $P$s are plane tessellation figures.

Condition 2 : There exists no place where both red $P$ and blue $P$ are placed.

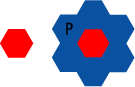
Note that a regular hexagon, for example, does not satisfy the condition 2. See the figure below. The blue $P$ on which a letter $P$ is written, for example, will be colored red by the next operation.

$\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ $enter image description here

Then, here is the first question.

Question 1 : Is the following true?

$P$ satisfies these two conditions $\iff$ $P$ is either "a $45–45–90$ triangle", "a $30–60–90$ triangle", "an equilateral triangle" or "a rectangle".

I reached this conjecture by considering the inner angles of $P$. The followings are what I've thought : Every inner angle, say $\alpha$, of $P$ has to satisfy $2m\alpha=360^{\circ}$ where $m\ge 2\in\mathbb N$. Hence, $\alpha$ has to be any of the positive divisors of $180$ except $180$. This leads $n\le 4$ and so on.

Then, here is the second question.

Question 2 : Letting $P$ be a convex polyhedron, how about the three dimensional version of this question? Suppose that we consider the plane symmetry instead of the line symmetry.

In the two dimensional version, I think we can consider the inner angles of $P$. However, I don't have any good idea for the higher dimensional version. Can anyone help?

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ Your operations are quite hard to follow - can you please edit to make it more precise? In operation 1, I take it that $P_0$ is a convex $n$-gon - you did not state this explicitly? Then in operation 2, are you constructing the reflections of $P_0$ in each of its edges? And in what follows I think you should use a better notation or set of names than "Let these $P$s be $P_1$" which seems at odds with your earlier conventions. $\endgroup$
    – j.c.
    Commented Nov 13, 2013 at 12:52
  • 3
    $\begingroup$ It seems what you are after is related to tilings generated by reflection symmetries, so I would suggest reading about Poincaré's polyhedron theorem. There is an accessible account in Bonahon's book "Low-Dimensional Geometry". $\endgroup$
    – j.c.
    Commented Nov 13, 2013 at 12:53
  • 1
    $\begingroup$ One difficulty is that there isn't a general formula like $\pi(n-2)$, which was nicely exploited in the answer for your first question, for the sum of the interior angles of a polyhedron. If you generalize the notion of an interior angle of a polygon to, say, a solid angle (see, e.g., Wikipedia), then you might find the following paper of interest: Barnette, D. (1972). The sum of the solid angles of a d-polytope. Geometriae dedicata, 1(1), 100-102. $\endgroup$
    – Benjamin Dickman
    Commented Nov 16, 2013 at 13:55
  • 1
    $\begingroup$ The group generated by the reflexions along faces of your polyhedron must be a finite group in order to get a tilling; you should therefore consider the classical classification of finite subgroups of $O(3)$. $\endgroup$
    – Benoît Kloeckner
    Commented Nov 16, 2013 at 15:27
  • 4
    $\begingroup$ Your question has a nice connection to billiards (which you might include as a tag). Instead of reflecting the path of the billiard particle, it is often useful to reflect the table. Certainly in 2D and I think beyond, this question generates exactly the set of polygonal billiard tables with integrable ("regular") dynamics. $\endgroup$
    – user25199
    Commented Nov 20, 2013 at 17:34

3 Answers 3

Reset to default
7
+50
$\begingroup$

The short answer, for arbitrary dimensions is this: You are asking for what polytopes can serve as the fundamental domain of an affine Coxeter group (represented as a group of Euclidean isometries of R^n). These are classified, and in particular, you are right about Question 1. I'll try to elaborate. A good reference for this is Humphreys "Reflection groups and Coxeter groups" which has a whole chapter on affine Coxeter groups. Much of what you need is there or is implicit, with maybe some connecting of dots needed.

First, extend the facets (maximal proper faces) of P to form a hyperplane (so a point in R, a line in R^2, a plane in R^3, etc.). The following facts are easy and/or standard (for people who think about reflection groups). None of these hyperplanes cuts the interior of any other copy of P in the tessellation. Also, reflection in each of these hyperplanes is a symmetry of the uncolored tiling. All of these reflections together generate a discrete group of Euclidean motions. It is known that an infinite discrete group of Euclidean motions generated by reflections is an affine Coxeter group. (Going the other direction, if you start with an affine Coxeter group represented as a group of Euclidean motions, you will get a polytope P fitting your description.)

In Humphreys, or for example at

http://en.wikipedia.org/wiki/Coxeter_group#Affine_Coxeter_groups

you'll find a list of diagrams (edge-labeled graphs) that classify affine Coxeter groups. Here's how to read your P from these diagrams.

Your graph will have c components, each of which is one of the connected graphs shown in the list. If there are k total vertices in all of the components, you will be constructing a polytope in R^n for n=k-c.

For a moment, take c=1. Then each vertex will be a facet of your polytope and the edges tell the angles between facets by the following rule:

no edge: angle is pi/2 unlabled edge: angle is pi/3 edge labeled m: angle is pi/m

The edge labeled infinity only happens for n=1, and that is saying that the two "facets" (points) of a line segment meet at an angle pi/infinity=0, which is right if you think about it.

If c>1, then you construct P_i for each component, and your P is the Cartesian product of all of the P_i. So for example, If P_1 is a line segment and P_2 is a triangle, you get a triangular prism, etc. But more interesting things can happen in R^3 than prisms. For example the diagram called "A_3 tilde" gives a tetrahedron. One pair of facets meets at right angles, the other two also meet at right angles, and all four other pairwise angles are pi/3.

If you put a tag of "Coxeter groups" on this question, I'm sure there will be plenty of people who can add details.

Improve this answer
$\endgroup$
17
$\begingroup$

Suppose your polygon has angles $\alpha_i=\frac{\pi}{k_i}$ where $k_i\geq 2$ are integers. Then they must satisfy $$\frac{1}{k_1}+\frac{1}{k_2}+\cdots+\frac{1}{k_n}=n-2,$$ which means $n-2\leq \frac{n}{2}$, so $n\leq 4$.

When $n=3$, one finds the solutions $(k_1,k_2,k_3)=(2,4,4),(3,3,3),(2,3,6)$ which correspond to the 45-45-90, equilateral, and 30-60-90 triangles respectively. When $n=4$ one gets only the solution $(k_1,k_2,k_3,k_4)=(2,2,2,2)$ which corresponds to rectangles. There are no more solutions.

Improve this answer
$\endgroup$
0
2
$\begingroup$

This is in response to questions posed in the comments, but is too long for a comment.

The use of the reflection trick in mathematical billiards, and detailed results for regular billiards are contained in

S. Tabachnikov Geometry and billiards (AMS, 2005).

including the classification of regular 2D billiards already given in another answer here. The connection between higher dimensional billiards and Coxeter groups was made in

A. Y. Plakhov and A. M. Stepin Scattering and Coxeter groups Russ. Math. Surv. 53 401-402 (1998).

Both conditions 1 and 2 are needed for regular polytope billiards. The red and blue polytopes then correspond to regions where the mapping back to the original space is a translation or a reflection. The condition that angles in a polygon are of the form $\pi/n$ is exactly what is needed for corners to be regular, in other words, parallel billiard paths that are close but pass on opposite sides of a corner remain close after reflections near the corner. The example of a hexagon, which tesselates the plane but does not satisfy condition 2, has corners not of this form and so parallel billiard paths on opposite sides of the corner emerge at different angles. I am not aware of results which distinguish this case from the very popular more general case of billiards with rational angles. These are described by translation surfaces of higher genus, for example see

V. Delecroix, P. Hubert and S. Lelievre Diffusion for the periodic wind-tree model arxiv:1107.1810

Improve this answer
$\endgroup$
0

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .