5
$\begingroup$

What does minimizing the Dirichlet energy of a mapping $\Phi$ achieve intuitively? Roughly it is the integral (or sum, if discrete) of $|\nabla \Phi(\;)|^2 dV$, with $V$ the volume. So is it, in some sense, a minimally distorting (on average) mapping? Here is an example,
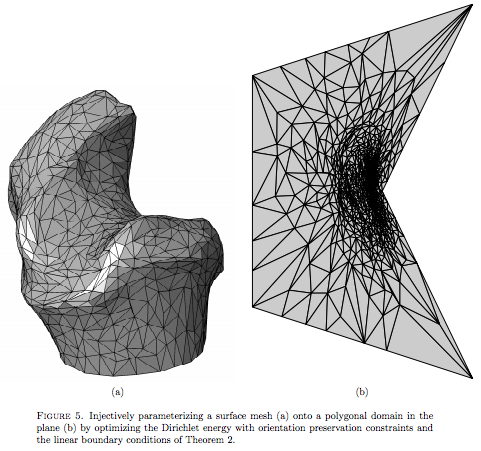
Figure 5
from

Yaron Lipman. "Construction of Injective Mappings Of Meshes." arXiv:1310.0955 [cs.CG].

But when I look at this figure and try to view it as minimally distorting, I don't see in what sense it minimally distorts—it greatly distorts! I feel like I am missing some basic intuition here. Any insights would be appreciated. Thanks!

Improve this question
$\endgroup$
3

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .